The rate constant of the first order reaction , that is decomposition of ethylene oxide into and CO, may be described by the following equation
rate constant at
The correct Answer is:
To find the rate constant k for the decomposition of ethylene oxide at 397∘C, we will use the given equation:
logk(s−1)=14.34−1.25×104T
Step 1: Convert the temperature from Celsius to Kelvin
To use the equation, we first need to convert the temperature from Celsius to Kelvin. The conversion formula is:
T(K)=T(°C)+273.15
For 397∘C:
T=397+273.15=670.15K
Step 2: Substitute the temperature into the equation
Now, we substitute T=670.15K into the equation for logk:
logk=14.34−1.25×104670.15
Step 3: Calculate the value of 1.25×104670.15
Now we calculate 1.25×104670.15:
1.25×104670.15≈18.65
Step 4: Substitute this value back into the equation
Now we can substitute this value back into the equation for logk:
logk=14.34−18.65
Step 5: Calculate logk
Now we perform the subtraction:
logk≈14.34−18.65≈−4.31
Step 6: Convert logk to k
To find k, we need to convert from logarithmic form to exponential form:
k=10logk=10−4.31
Step 7: Calculate k
Now we calculate k:
k≈4.88×10−5s−1
Final Answer
The rate constant k at 397∘C is approximately:
k≈4.88×10−5s−1
---
To use the equation, we first need to convert the temperature from Celsius to Kelvin. The conversion formula is:
Now, we substitute T=670.15K into the equation for logk:
Now we calculate 1.25×104670.15:
Now we can substitute this value back into the equation for logk:
Now we perform the subtraction:
To find k, we need to convert from logarithmic form to exponential form:
Now we calculate k:
The rate constant k at 397∘C is approximately:
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - RC MUKHERJEECHAPTER - CHEMICAL KINETICSEXERCISE - OBJECTIVE PROBLEMS
51 Videos
- CHEMICAL KINETICSBOOK - RC MUKHERJEECHAPTER - CHEMICAL KINETICSEXERCISE - OBJECTIVE PROBLEMS
51 Videos
- CHEMICAL EQUIVALENCEBOOK - RC MUKHERJEECHAPTER - CHEMICAL EQUIVALENCEEXERCISE - PROBLEMS
24 Videos
- CHEMICAL THERMODYNAMICSBOOK - RC MUKHERJEECHAPTER - CHEMICAL THERMODYNAMICSEXERCISE - Objective problems
58 Videos
Similar Questions
The rate constant of the first order reaction , that is decomposition of ethylene oxide into CH4 and CO, may be described by the following equation
logk(s−1)=14.34−1.25×104TK
Find (a) energy of activation ,
View SolutionRate constant for the decomposition of ethylene oxide into CH4 and CO may be described be the equation. logk(s)−1=14.34−1.25×104T
(a) What is the energy of activation of this reaction ?
(b) What is the value of k at 670K ?
View Solution
Knowledge Check
The rate constant for the first order decomposition of ethylene oxide into CH4 and CO,
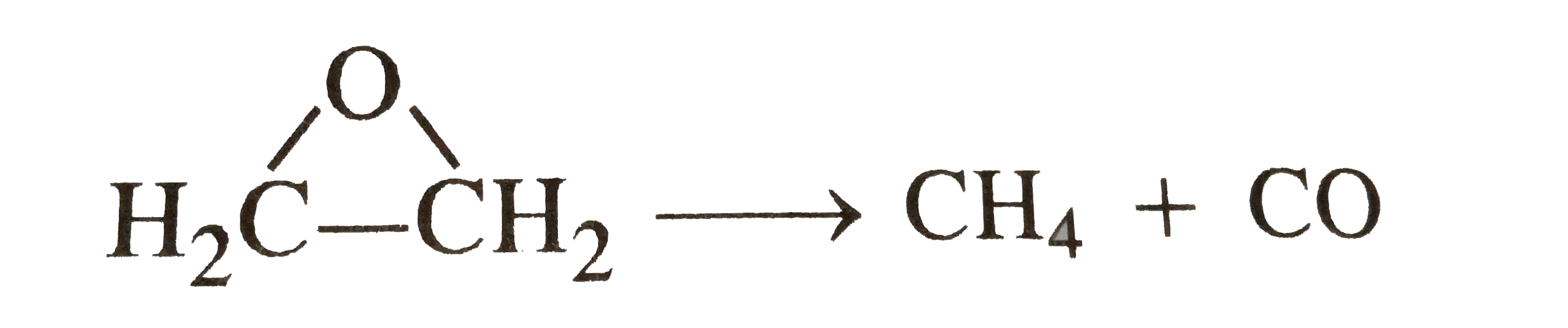
may be describe by
logk(ins−1)=14.34−1.25×104KT
The activation energy of the reaction isA4.8×10−5kJmol−1B4.8×105kJmol−1C2.39×102kJmol−1D2.39×105kJmol−1The rate constant for the decompoistion of a certain reaction is described by the equation:
logk(s−1)=14−1.25×104KT
Energy of activation (in kcal) isA57.6kcalB1.25×104kcalC14.0kcalD14×104kcalThe rate constant for the decompoistion of a certain reaction is described by the equation:
logk(s−1)=14−1.25×104KT
Pre-exponential factor for this reaction isA14s−1B1014s−1C10−14s−1D1.25×104s−1
Rate constant for the decomposition of ethylene oxide into CH4 and CO may be described by the equation logl(s−1)=14.34−1.25×104T
(a) What is the energy of activation of this reaction ?
(b) What is the value of k at 670K ?
View SolutionThe rate constant for the first order decompoistion of a certain reaction is described by the equation
logk(s−1)=14.34−1.25×104KT
(a) What is the energy of activation for the reaction?
(b) At what temperature will its half-life periof be 256min?
View SolutionThe rate constant for the first order decompoistion of a certain reaction is described by the equation
logk(s−1)=14.34−1.25×104KT
(a) What is the energy of activation for the reaction?
(b) At what temperature will its half-life periof be 256min?
View SolutionThe rate constant for the first order decomposition of H2O2 is given by the following equation : log K = 14.2 - 1.0×104TK
Calculate Ea for this reaction and rate constant k if its half life period be 200 minutes. (Given : R = 8.314 JK−1mol−1)
View SolutionThe rate constant for the decompoistion of a certain reaction is described by the equation:
logk(s−1)=14−1.25×104KT
At what temperature, rate constant is equal to pre-exponential factor?
View Solution
