Arrange the wavelengths (λ) of the following emission lines of H-atom in an increasing order. (1) n=3λ1−−→n=1 (2) n=5λ2−−→n=3 (3) n=12λ3−−→n=10 (4) n=22λ4−−→n=20
λ4<λ3<λ2<λ1
λ1<λ2<λ3<λ4
λ1<λ2<λ4<λ3
λ1<λ3<λ4<λ2
To solve the problem of arranging the wavelengths of the emission lines of the hydrogen atom in increasing order, we will use the Rydberg formula for hydrogen. The formula for the wavelength (λ) of the emitted light when an electron transitions between energy levels is given by:
1λ=RH⎛⎝1n2f−1n2i⎞⎠
Where:
- RH is the Rydberg constant (approximately 1.097×107m−1),
- nf is the final energy level,
- ni is the initial energy level.
1. Calculate 1λ1 for n=3→n=1:
1λ1=RH(112−132)=RH(1−19)=RH(89)
2. Calculate 1λ2 for n=5→n=3:
1λ2=RH(132−152)=RH(19−125)=RH(25−9225)=RH(16225)
3. Calculate 1λ3 for n=12→n=10:
1λ3=RH(1102−1122)=RH(1100−1144)=RH(144−10014400)=RH(4414400)
4. Calculate 1λ4 for n=22→n=20:
1λ4=RH(1202−1222)=RH(1400−1484)=RH(484−400193600)=RH(84193600)
5. Compare the values of 1λ:
- 1λ1=RH(89)
- 1λ2=RH(16225)
- 1λ3=RH(4414400)
- 1λ4=RH(84193600)
6. Convert to wavelengths:
- The smaller the value of 1λ, the larger the wavelength λ.
- Therefore, we need to find the order of 1λ values to arrange the wavelengths in increasing order.
7. Final Arrangement:
- Calculate the numerical values:
- 89 is the largest,
- 16225 is smaller than 89,
- 4414400 is smaller than 16225,
- 84193600 is the smallest.
- Thus, the order of wavelengths from smallest to largest is:
λ4<λ3<λ2<λ1
Final Answer:
The increasing order of wavelengths is:
λ4<λ3<λ2<λ1
- RH is the Rydberg constant (approximately 1.097×107m−1),
- nf is the final energy level,
- ni is the initial energy level.
1λ1=RH(112−132)=RH(1−19)=RH(89)
1λ2=RH(132−152)=RH(19−125)=RH(25−9225)=RH(16225)
1λ3=RH(1102−1122)=RH(1100−1144)=RH(144−10014400)=RH(4414400)
1λ4=RH(1202−1222)=RH(1400−1484)=RH(484−400193600)=RH(84193600)
- 1λ1=RH(89)
- 1λ2=RH(16225)
- 1λ3=RH(4414400)
- 1λ4=RH(84193600)
- The smaller the value of 1λ, the larger the wavelength λ.
- Therefore, we need to find the order of 1λ values to arrange the wavelengths in increasing order.
- Calculate the numerical values:
- 89 is the largest,
- 16225 is smaller than 89,
- 4414400 is smaller than 16225,
- 84193600 is the smallest.
- Thus, the order of wavelengths from smallest to largest is:
λ4<λ3<λ2<λ1
The increasing order of wavelengths is:
λ4<λ3<λ2<λ1
Topper's Solved these Questions
Similar Questions
A radioactive element decays by following three different parallel paths :
Aλ1−−→B,λ1=5×10−2sec−1
2Aλ2−−→C,λ2=3×10−2sec−1
3Aλ3−−→D,λ3=5×10−3sec−1
Calculate average life- time of element (in sec.)
[λ1,λ2,λ3are decay constans for respective reactions]
View SolutionLet P(n):3n<1×2×3×........×n,n∈N is always true for n≥λ then smallest value of λ is
View Solution
Knowledge Check
Arrange the wavelengths (a) of the following emission lines of H-atom in an increasing order.
(1) n=3λ1−−→n=1 (2) n=5λ2−−→n=3
(3) n=12λ3−−→n=10 (4) n=22λ4−−→n=20Aλ4<λ3<λ2<λ1Bλ1<λ2<λ3<λ4Cλ1<λ2<λ4<λ3Dλ1<λ3<λ3<λ4λ2BaC2+N2Δ−−→(A)CaC2+N2Δ−−→(B)
(A) and (B) areABaCN2,CaCN2BBa(CN)2,Ca(CN)2CBa(CN)2,CaCN2Dnone of the aboveAλ−−→B2λ−−→C
T=0,N0,0,
TN1N2N3
The ratio of N1 to N2 is maximum I sAat no time this is possibleB2C1/2Dln22
How many N atom are present in final product
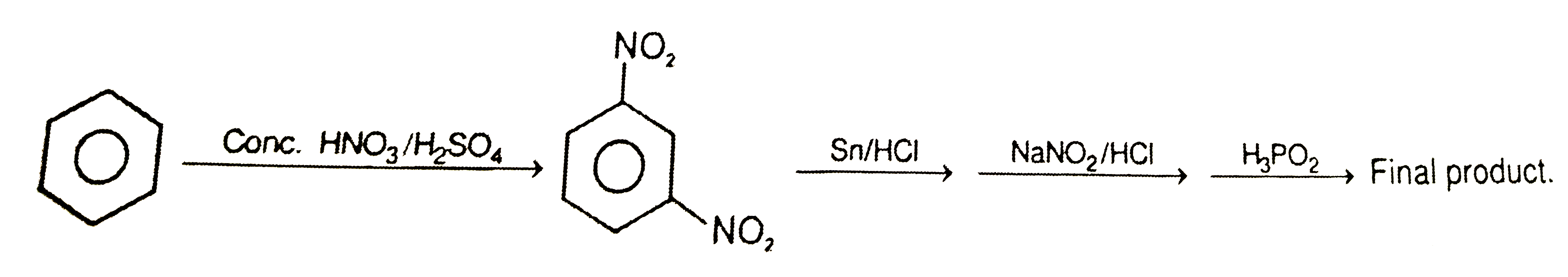 Sn/HCl−−−−→NaNO2/HCl−−−−−−−→H3PO2−−−→ Final product.
Sn/HCl−−−−→NaNO2/HCl−−−−−−−→H3PO2−−−→ Final product.
View SolutionA' in the following series of reaction is :
A[O]−−→BNH3OH−−−−→(CH3)2C=N−OH
View SolutionAλ1−−→Bλ2−−→C
t=0 N0 0 0
tN1N2N3 In the above radioactive decay C is stable nucleus. Then:
View SolutionThe average (mean) life at a radio nuclide which decays by parallel path is :
Aλ1−−→B,λ1=1.8×10−2sec−1
2Aλ2−−→C,λ2=10−3sec−1
View SolutionIf →B=n→A and →A is antiparallel with →B, then n is :
View Solution
