State Raoult's law for a solution of volatile liquids.
Step-by-Step Text Solution:
1. Definition of Volatile Liquid:
- A volatile liquid is defined as a liquid that has a tendency to evaporate easily at a given temperature. This means that it has a relatively high vapor pressure compared to non-volatile liquids.
2. Statement of Raoult's Law:
- Raoult's Law states that for a solution of volatile liquids, the partial vapor pressure of each component in the solution is directly proportional to the vapor pressure of the pure component and its mole fraction in the solution.
3. Mathematical Representation:
- For a binary solution containing two volatile liquids, A and B, we denote their mole fractions as XA and XB.
- The vapor pressures of the pure components are denoted as P0A for component A and P0B for component B.
- According to Raoult's Law:
- The partial vapor pressure of component A in the solution is given by:
PA=P0A⋅XA
- The partial vapor pressure of component B in the solution is given by:
PB=P0B⋅XB
4. Total Vapor Pressure:
- According to Dalton's Law of Partial Pressures, the total vapor pressure P of the solution is the sum of the partial pressures of its components:
P=PA+PB
- Substituting the expressions for PA and PB from Raoult's Law, we get:
P=P0A⋅XA+P0B⋅XB
5. Using the Mole Fraction Relationship:
- Since the sum of the mole fractions in a binary solution is equal to 1:
XA+XB=1⇒XA=1−XB
- Substituting XA in the total pressure equation gives:
P=P0A⋅(1−XB)+P0B⋅XB
- This can be rearranged to express the total pressure in terms of the mole fraction of component B:
P=P0A+P0B−P0A⋅XB
- A volatile liquid is defined as a liquid that has a tendency to evaporate easily at a given temperature. This means that it has a relatively high vapor pressure compared to non-volatile liquids.
- Raoult's Law states that for a solution of volatile liquids, the partial vapor pressure of each component in the solution is directly proportional to the vapor pressure of the pure component and its mole fraction in the solution.
- For a binary solution containing two volatile liquids, A and B, we denote their mole fractions as XA and XB.
- The vapor pressures of the pure components are denoted as P0A for component A and P0B for component B.
- According to Raoult's Law:
- The partial vapor pressure of component A in the solution is given by:
PA=P0A⋅XA
- The partial vapor pressure of component B in the solution is given by:
PB=P0B⋅XB
- According to Dalton's Law of Partial Pressures, the total vapor pressure P of the solution is the sum of the partial pressures of its components:
P=PA+PB
- Substituting the expressions for PA and PB from Raoult's Law, we get:
P=P0A⋅XA+P0B⋅XB
- Since the sum of the mole fractions in a binary solution is equal to 1:
XA+XB=1⇒XA=1−XB
- Substituting XA in the total pressure equation gives:
P=P0A⋅(1−XB)+P0B⋅XB
- This can be rearranged to express the total pressure in terms of the mole fraction of component B:
P=P0A+P0B−P0A⋅XB
Topper's Solved these Questions
- SOLUTIONSBook:ICSEChapter:SOLUTIONSExercise:EXERCISE (PART-II Descriptive Questions) (SHORT ANSWER QUESTIONS)
Explore 34 Videos
- SOLUTIONSBook:ICSEChapter:SOLUTIONSExercise:EXERCISE (PART-II Descriptive Questions) (LONG ANSWER QUESTIONS)
Explore 21 Videos
- SOLUTIONSBook:ICSEChapter:SOLUTIONSExercise:EXERCISE (PART-I Objective Questions) (Choose the correct alternative)
Explore 35 Videos
- SOLID STATEBook:ICSEChapter:SOLID STATEExercise:ISC EXAMINATION QUESTIONS PART-I (Numerical Problems)
Explore 6 Videos
- SOME IMPORTANT ORGANIC NAME REACTIONS Book:ICSEChapter:SOME IMPORTANT ORGANIC NAME REACTIONS Exercise:Questions
Explore 278 Videos
Similar Questions
State Raoult's law for solution containing volatile liquid components. Taking a sutiable example, explan the meaning of positive deviation from Raoult's law .
View SolutionState Raoult's law for a solution containing volatile components. Write two characteristics of the solution which obeys Raoult's law at all concentrations.
View Solution(a) State Raoult's law for a solution containing volatile components.
How does Raoult' s law become a special case of Henry's law?
(b) 1.00 g of a non-electrolyte solute dissolved in 50 g of benzene lowered the freezing point of benzene by 0.40 K. Find the molar mass of the solute. (Kf for benzene =5.12kgmol−1)
View SolutionState Raoult's law for the solution containing volatile components. What is the similarity .
between Raoult's law and Henry's law ?
View SolutionState Raoult's law for a binary solution containing volatile components.
View SolutionExplain Raoult's law for a solution containing non-volatile solute.
View SolutionWrite expressions for the Raoult's law for non-volatile solutes.
View SolutionDefine Raoult's law for the elevation of boiling point of a solution.
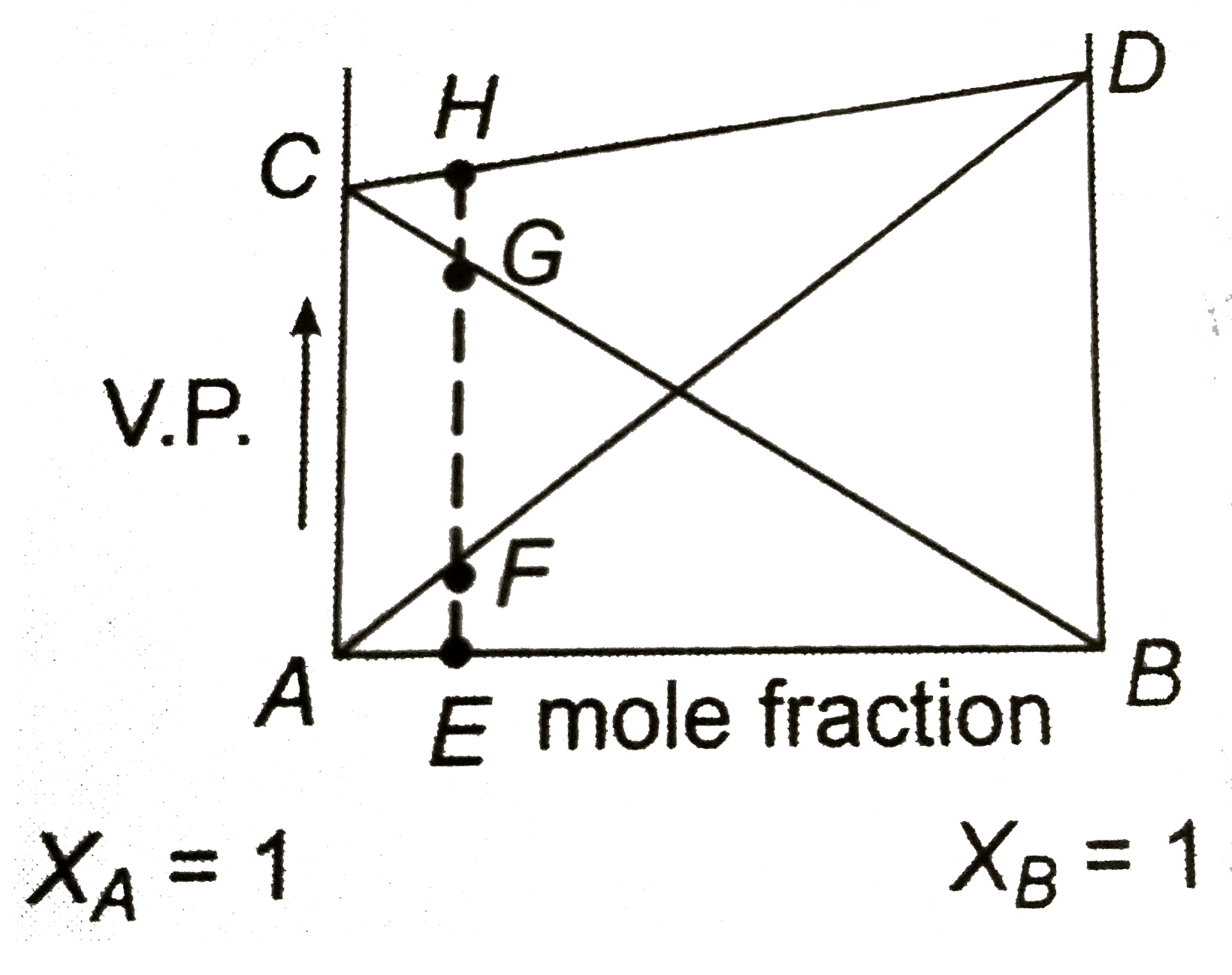
View SolutionBased on the given diagram, which of the following statements regarding the homogenous solution of two volatile liquids are correct? (1) Plots AD and BC show that Raoult's law is obeyed for the solution in which B is a solvent and A is the solute and as well as for that in which A is solvent and B is solute. (2) Plot CD shows that Dalton's law of partial pressures is obeyed by the binary solution of components A and B. (3) EF + EG = EH; and AC and BD correspond to the vapour pressure of the pure solvents A and B respectively.
View SolutionSTATEMENT-1 : For solution of volatile liquids, the partial vapour pressure of each component in the solution is directly proportional to its mole fraction.
STATEMENT-2 : Always there will be lowering of vapour pressure on addition of non-volatile solute to a solvent.
STATEMENT-3 : If there is dissociation of non-volatile then the V.P. of solution increases.
View Solution