In parallelogram ABCD, E is a point in AB and DE meets diagonal AC at point F. If DF:FE= 5:3 and area of ΔADF is 60cm2, find :
(i) area of ΔADE
(ii) if AE:EB= 4:5, find the area of ΔADB
(iii) also, find area of parallelogram ABCD.
The correct Answer is:(i) 96cm2(ii)216cm2 (iii) 432cm2
To solve the problem step by step, we will break it down into three parts as per the question.
Given Information:
- In parallelogram ABCD, E is a point on AB.
- DE meets diagonal AC at point F.
- DF:FE = 5:3
- Area of triangle ADF = 60 cm²
(i) Find the area of triangle ADE
1. Understanding the relationship between triangles ADF and AFE:
- Triangles ADF and AFE share the same vertex A and have the same base DE.
- Therefore, the ratio of their areas is equal to the ratio of their corresponding segments on line DE.
2. Setting up the ratio:
- Given DF:FE = 5:3, we can denote the area of triangle AFE as x.
- Thus, we have:
Area of △ADFArea of △AFE=DFFE=53
- Substituting the known area of triangle ADF:
60x=53
3. Cross-multiplying to find x:
60⋅3=5⋅x⟹180=5x⟹x=1805=36 cm2
4. Finding the area of triangle ADE:
- Area of triangle ADE = Area of triangle ADF + Area of triangle AFE
Area of △ADE=60+36=96 cm2
(ii) If AE:EB = 4:5, find the area of triangle ADB
1. Understanding the relationship between triangles ADE and EDB:
- Triangles ADE and EDB share the same vertex D and lie on the same line AD.
- Therefore, the ratio of their areas is equal to the ratio of AE to EB.
2. Setting up the ratio:
- Let the area of triangle EDB be y.
- Given AE:EB = 4:5, we have:
Area of △ADEArea of △EDB=AEEB=45
- Substituting the known area of triangle ADE:
96y=45
3. Cross-multiplying to find y:
96⋅5=4⋅y⟹480=4y⟹y=4804=120 cm2
4. Finding the area of triangle ADB:
- Area of triangle ADB = Area of triangle ADE + Area of triangle EDB
Area of △ADB=96+120=216 cm2
(iii) Find the area of parallelogram ABCD
1. Using the relationship between triangle ADB and parallelogram ABCD:
- The area of triangle ADB is half of the area of parallelogram ABCD.
Area of △ADB=12×Area of parallelogram ABCD
- Substituting the area of triangle ADB:
216=12×Area of parallelogram ABCD
2. Solving for the area of parallelogram ABCD:
Area of parallelogram ABCD=216×2=432 cm2
Final Answers:
(i) Area of triangle ADE = 96 cm²
(ii) Area of triangle ADB = 216 cm²
(iii) Area of parallelogram ABCD = 432 cm²
- In parallelogram ABCD, E is a point on AB.
- DE meets diagonal AC at point F.
- DF:FE = 5:3
- Area of triangle ADF = 60 cm²
- Triangles ADF and AFE share the same vertex A and have the same base DE.
- Therefore, the ratio of their areas is equal to the ratio of their corresponding segments on line DE.
- Given DF:FE = 5:3, we can denote the area of triangle AFE as x.
- Thus, we have:
Area of △ADFArea of △AFE=DFFE=53
- Substituting the known area of triangle ADF:
60x=53
60⋅3=5⋅x⟹180=5x⟹x=1805=36 cm2
- Area of triangle ADE = Area of triangle ADF + Area of triangle AFE
Area of △ADE=60+36=96 cm2
- Triangles ADE and EDB share the same vertex D and lie on the same line AD.
- Therefore, the ratio of their areas is equal to the ratio of AE to EB.
- Let the area of triangle EDB be y.
- Given AE:EB = 4:5, we have:
Area of △ADEArea of △EDB=AEEB=45
- Substituting the known area of triangle ADE:
96y=45
96⋅5=4⋅y⟹480=4y⟹y=4804=120 cm2
- Area of triangle ADB = Area of triangle ADE + Area of triangle EDB
Area of △ADB=96+120=216 cm2
- The area of triangle ADB is half of the area of parallelogram ABCD.
Area of △ADB=12×Area of parallelogram ABCD
- Substituting the area of triangle ADB:
216=12×Area of parallelogram ABCD
Area of parallelogram ABCD=216×2=432 cm2
(i) Area of triangle ADE = 96 cm²
(ii) Area of triangle ADB = 216 cm²
(iii) Area of parallelogram ABCD = 432 cm²
Topper's Solved these Questions
- AREA THEOREMSBOOK - ICSECHAPTER - AREA THEOREMSEXERCISE - Exercies 16(B)
16 Videos
- AREA AND PERIMETER OF PLANE FIGURESBOOK - ICSECHAPTER - AREA AND PERIMETER OF PLANE FIGURESEXERCISE - EXERCISE 20(D)
12 Videos
- CHAPTER REVISION (STAGE 2) BOOK - ICSECHAPTER - CHAPTER REVISION (STAGE 2) EXERCISE - DISTANCE FORMULA
12 Videos
Similar Questions
In parallelogram ABCD, P is mid-point of AB. CP and BD intersect each other at point O. If area of ΔPOB=40cm2 and OP:OC= 1:2, find :
(i) Areas of ΔBOC and ΔPBC
(ii) Areas of ΔABC and parallelogram ABCD.
View SolutionABCD is a parallelogram. Find the ratio of the area of Δ ABC to the area of the parallelogram ABCD.
View Solution
Knowledge Check
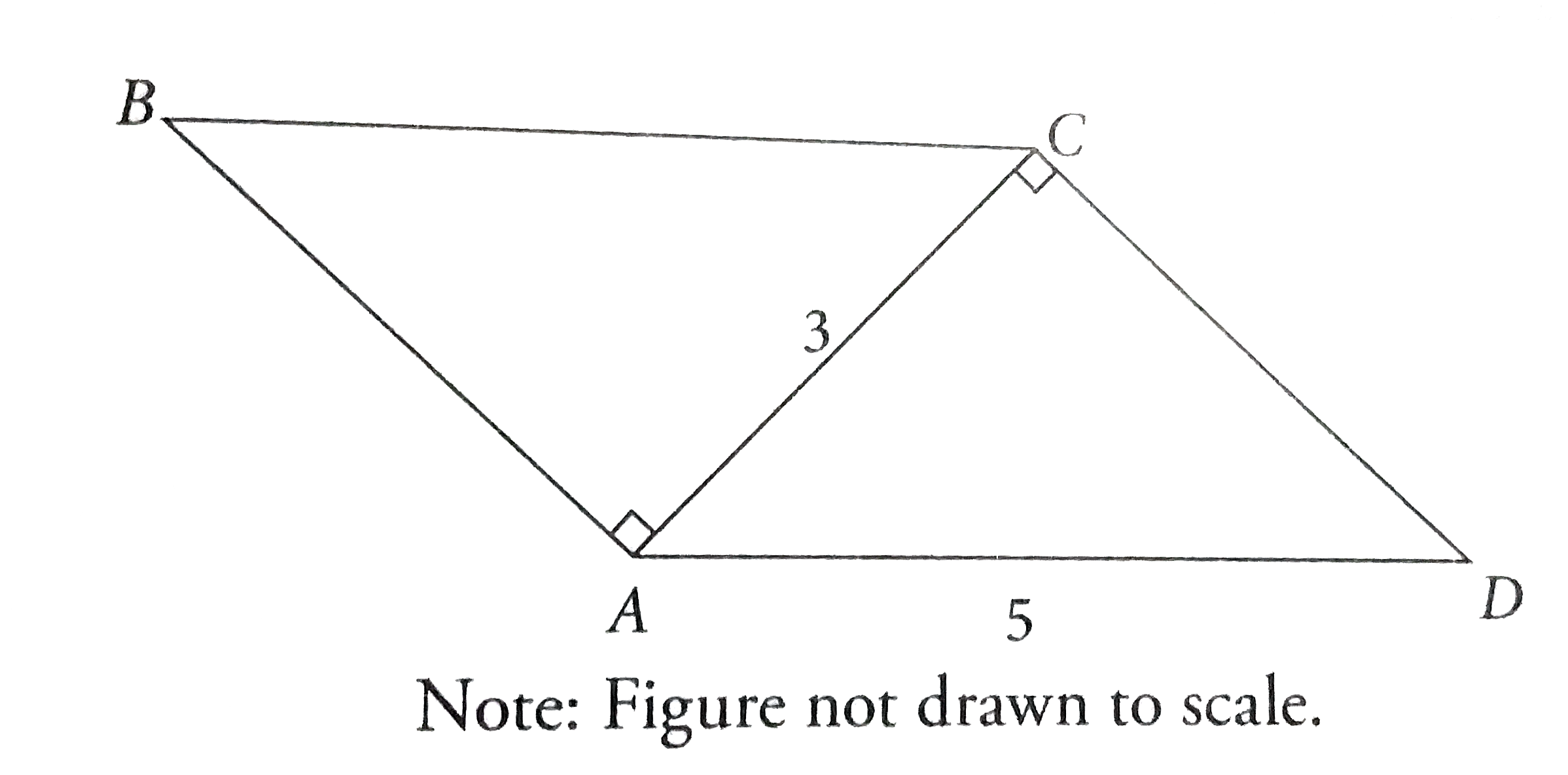
In parallelogram ABCD above, AC=3 and AD=5. What is the area of ABCD?A12B15C18D20
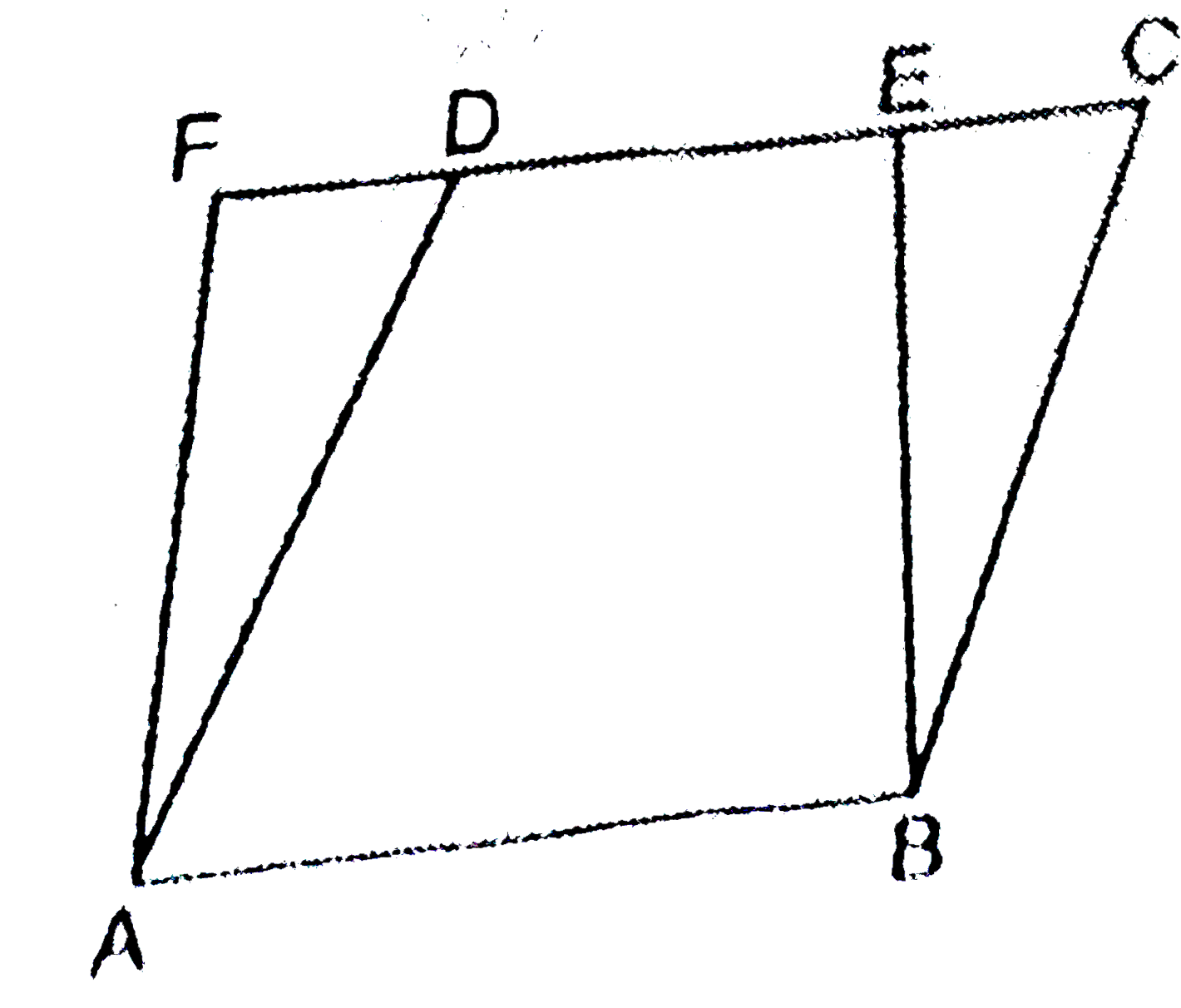
In the given figure, if the area of parallelogram ABCD is 40cm2, find the area of parallelogram ABEF
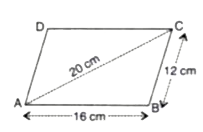
View SolutionIn parallelogram ABCD, AB = 16 cm, BC = 12 cm and diagonal AC = 20 cm. Find the area of the parallelogram.
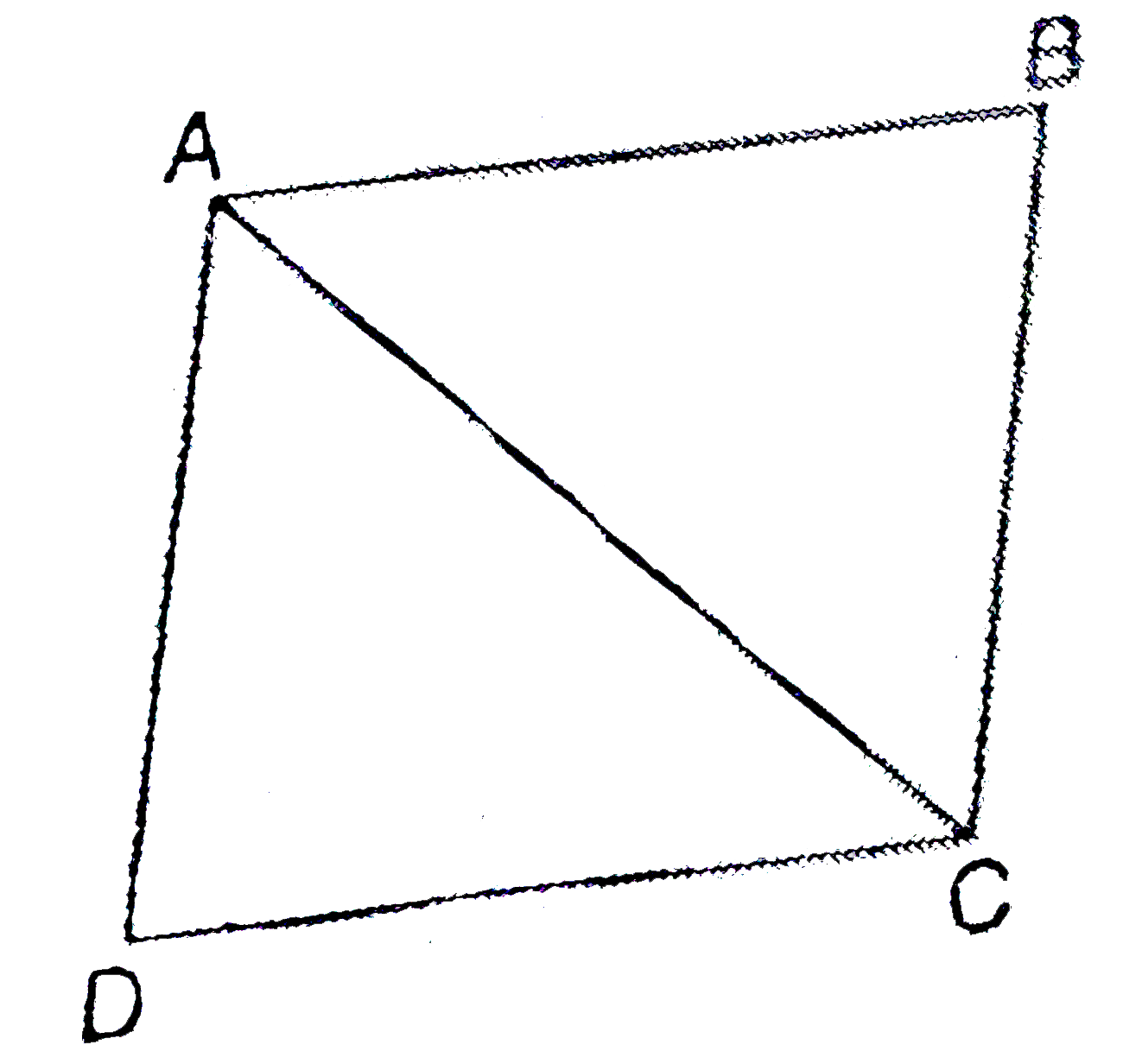
View SolutionIn the given figure, ABCD is a parallelogram whose area is 60cm2. Find the area of ΔACB
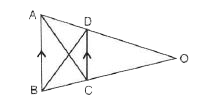
View SolutionIn the following figure, OAB is a triangle and AB//DC.
If the area of ΔCAD=140cm2 and the area of ΔODC=172cm2, find
(i) the area of ΔDBC
(ii) the area of ΔOAC
(iii) the area of ΔODB.
View SolutionIn ΔABC, E is mid-point of side AB and EBCD is a parallelogram. If the area of ΔABC is 80 cm, find the area of parallelogram EBCD.
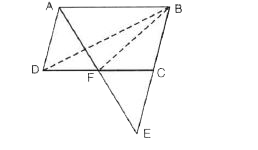
View SolutionABCD is a parallelogram in which BC is produced to E such that CE=BC and AE intersects CD at F.
If ar.(ΔDFB)=30cm2, find the area of parallelogram
View SolutionIn a parallelogram ABCD, point P lies in DC such that DP:PC= 3:2. If area of ΔDPB=30sq. cm, ffind the area of the parallelogram ABCD.
View Solution
