A shopkeeper mixes a batch of 200 apples of mean mass 150 g and standard deviation 30 g with another batch of 300 apples of mean 100 g and standard deviation 20 g. Find the standard deviation for the combined batch of 500 apples.
The correct Answer is:36.64 g
To find the standard deviation of the combined batch of apples, we can follow these steps:
Step 1: Gather the given data
- For the first batch:
- Number of apples, n1=200
- Mean mass, ¯x1=150g
- Standard deviation, σ1=30g
- For the second batch:
- Number of apples, n2=300
- Mean mass, ¯x2=100g
- Standard deviation, σ2=20g
Step 2: Calculate the combined mean
The combined mean ¯x12 can be calculated using the formula:
¯x12=n1¯x1+n2¯x2n1+n2
Substituting the values:
¯x12=200×150+300×100200+300=30000+30000500=60000500=120g
Step 3: Calculate deviations from the combined mean
- Deviation for the first batch:
d1=¯x12−¯x1=120−150=−30
- Deviation for the second batch:
d2=¯x12−¯x2=120−100=20
Step 4: Use the formula for combined standard deviation
The formula for the combined standard deviation σ is:
σ=√n1σ21+n1d21+n2σ22+n2d22n1+n2
Substituting the values:
σ=√200×302+200×(−30)2+300×202+300×202500
Calculating each component:
- 302=900
- (−30)2=900
- 202=400
Now substituting these values:
σ=√200×900+200×900+300×400+300×400500
=√180000+180000+120000+120000500
=√600000500=√1200
Step 5: Calculate the final standard deviation
σ=√1200≈34.64g
Final Answer
The standard deviation for the combined batch of 500 apples is approximately 34.64g.
---
- For the first batch:
- Number of apples, n1=200
- Mean mass, ¯x1=150g
- Standard deviation, σ1=30g
- Number of apples, n2=300
- Mean mass, ¯x2=100g
- Standard deviation, σ2=20g
The combined mean ¯x12 can be calculated using the formula:
¯x12=n1¯x1+n2¯x2n1+n2
¯x12=200×150+300×100200+300=30000+30000500=60000500=120g
- Deviation for the first batch:
d1=¯x12−¯x1=120−150=−30
d2=¯x12−¯x2=120−100=20
The formula for the combined standard deviation σ is:
σ=√n1σ21+n1d21+n2σ22+n2d22n1+n2
σ=√200×302+200×(−30)2+300×202+300×202500
- 302=900
- (−30)2=900
- 202=400
σ=√200×900+200×900+300×400+300×400500
=√180000+180000+120000+120000500
=√600000500=√1200
σ=√1200≈34.64g
The standard deviation for the combined batch of 500 apples is approximately 34.64g.
Topper's Solved these Questions
Similar Questions
Find the mean and the standard deviation from the following:
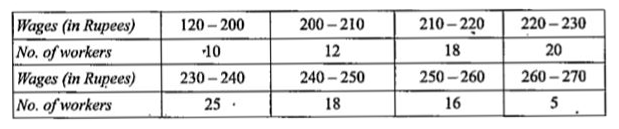
View SolutionFind the mean and standard deviation from the following data :

View SolutionThe first of two samples has 100 times with mean 15 and standard deviations 3. If the whose group has 250 items with mean 15.6 and standard deviation √3.44 Find the standard deviation of the second group.
View SolutionFind the mean and standard deviation using short cut method

View SolutionA sample of 35 observations has mean 80 men and standard deviation 4. A second sample of 65 observations has mean 70 and standard deviation =3. Find the combined mean and standard deviation.
View SolutionFind the standard deviation from the following datas, using assumed mean:

View SolutionFind the standard deviation from the following datas, using assumed mean:
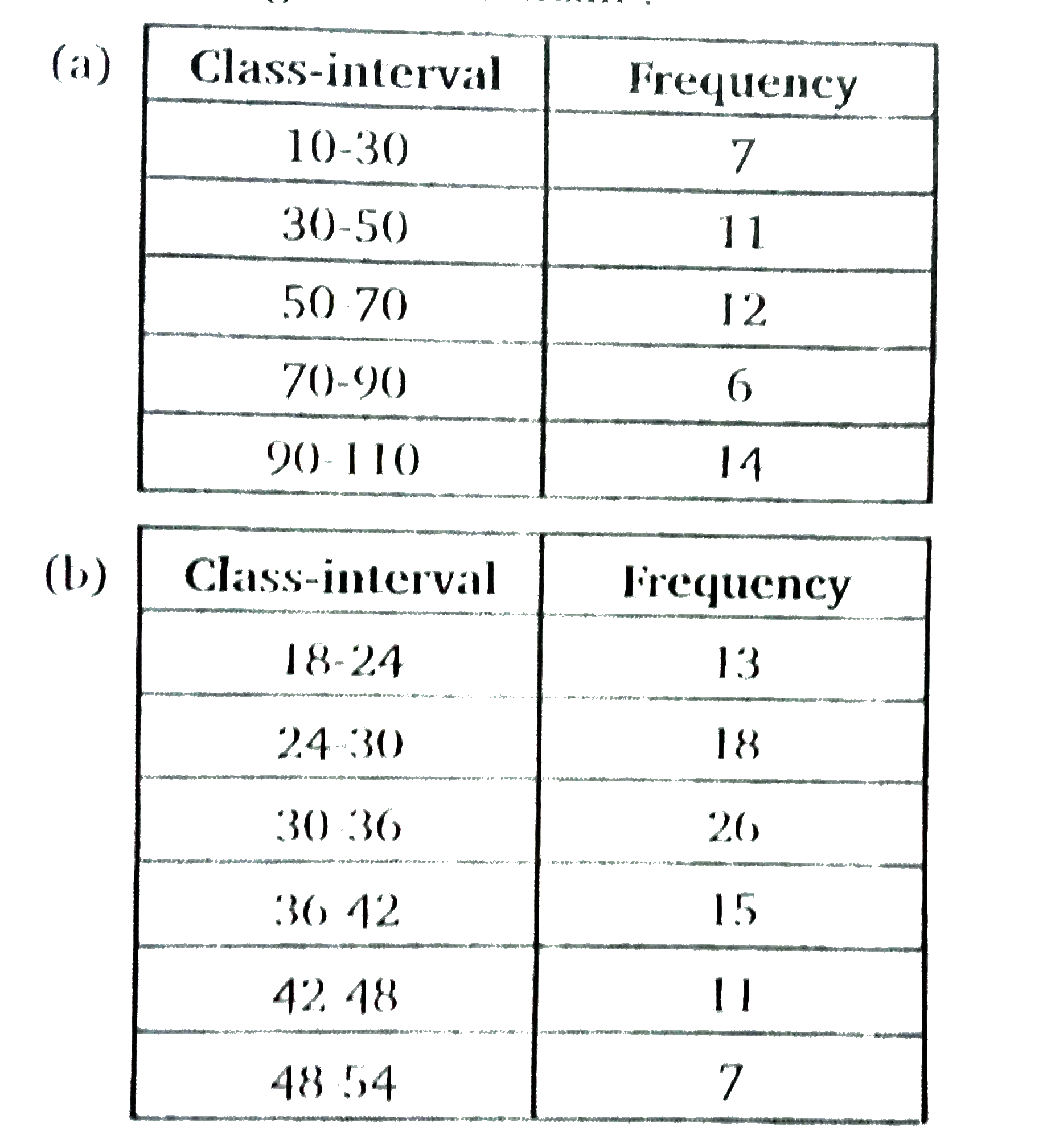
View SolutionFind the standard deviation by using 20 as assumed mean.

View SolutionOut of total sample survey of 100 nuts, in a first sample survey of 35 items has mean 80 and standard deviation 4. A second sample survey of 65 items has mean 70 and standard deviation 3. Find the mean and variance of combined 100 sample nuts.
View SolutionIf the mean of a binomial distribution is 20 and its standard deviation is 4, find p .
View Solution
