Following is a data sample of 8 observations in ascending order. If the mean of this data is 31 and the mode is 35. Find the missing observations.
20 24 x 30 31 35 y 45
The correct Answer is:28,35
To solve the problem step by step, we need to find the missing observations x and y in the data set:
20, 24, x, 30, 31, 35, y, 45.
Given:
- Mean = 31
- Mode = 35
Step 1: Understand the Mode
The mode is the value that appears most frequently in a data set. Since the mode is 35, it must appear more than once. In the given data, we can see that 35 appears only once. Therefore, for the mode to be 35, y must also be 35.
Conclusion:
y=35
Step 2: Calculate the Mean
The mean is calculated using the formula:
Mean=Sum of observationsNumber of observations
Here, the number of observations is 8. So, we can express the mean as:
31=20+24+x+30+31+35+y+458
Step 3: Substitute y in the Mean Formula
Now, substituting y=35 into the equation:
31=20+24+x+30+31+35+35+458
Step 4: Calculate the Sum of Known Values
Calculating the sum of the known values:
20+24+30+31+35+35+45=220
So, we can rewrite the equation:
31=220+x8
Step 5: Multiply Both Sides by 8
To eliminate the fraction, multiply both sides by 8:
31×8=220+x
Calculating 31×8:
248=220+x
Step 6: Solve for x
Now, isolate x:
x=248−220
Calculating the right side:
x=28
Final Conclusion
Thus, the missing observations are:
x=28andy=35
Summary of Results
The final values are:
- x=28
- y=35
---
- Mean = 31
- Mode = 35
The mode is the value that appears most frequently in a data set. Since the mode is 35, it must appear more than once. In the given data, we can see that 35 appears only once. Therefore, for the mode to be 35, y must also be 35.
y=35
The mean is calculated using the formula:
Mean=Sum of observationsNumber of observations
Here, the number of observations is 8. So, we can express the mean as:
31=20+24+x+30+31+35+y+458
Now, substituting y=35 into the equation:
31=20+24+x+30+31+35+35+458
Calculating the sum of the known values:
20+24+30+31+35+35+45=220
So, we can rewrite the equation:
31=220+x8
To eliminate the fraction, multiply both sides by 8:
31×8=220+x
Calculating 31×8:
248=220+x
Now, isolate x:
x=248−220
Calculating the right side:
x=28
Thus, the missing observations are:
x=28andy=35
The final values are:
- x=28
- y=35
Topper's Solved these Questions
- DATA HANDLING AND MEASURES OF CENTRAL TENDENCYBOOK - ICSECHAPTER - DATA HANDLING AND MEASURES OF CENTRAL TENDENCYEXERCISE - WARM-UP EXERCISE
5 Videos
- CONSTRUCTIONS BOOK - ICSECHAPTER - CONSTRUCTIONS EXERCISE - REVISION EXERCISE
12 Videos
- DATA HANDLING BOOK - ICSECHAPTER - DATA HANDLING EXERCISE - EXERCISE 24D
13 Videos
Similar Questions
Following is a data sample in descending order.
If the mean is 18.2 and the mode of this data is 17.5. find the missing numbers. 22.1, 20.9, x , 18.6, y, 17.5 ,13.5
View SolutionFollowing are the observations of a data sample in ascending order. If the mean of the sample is 18.5 and the mode is 15.5, find the missing data items. 12.5 13.8 15.5 x 19.2 20.4 y 23.5 24.6
View SolutionFollowing is a data sample in ascending order. The data has mean 4.1 and mode 4.9. Find the missing numbers. 2.4 3.1 3.8 X 4.9 Y 5.4
View SolutionCreate data of 8 observations with mean 10.
View SolutionThe mean of 20 observations is 30. If the mean of first 15 observations is 32, find the mean of last 5 observations.
View SolutionA data has 19 observation arranged in ascending order. Which observation reprsent the median.
View SolutionFind the mean of 32, 35, 36, 31 and 41.
View SolutionThe following observations have been arranged in ascending order. If the median of the data is 63, find the value of x. 29, 32, 48, 50, x, x + 2, 72, 78, 84, 95
View SolutionThe following observations have been arranged in ascending order. If the median of the data is 63, find the value of x. 29, 32, 48, 50, x, x + 2, 72, 78, 84, 95
View SolutionIf the mean of the following data is 20.2, then find the value of p.
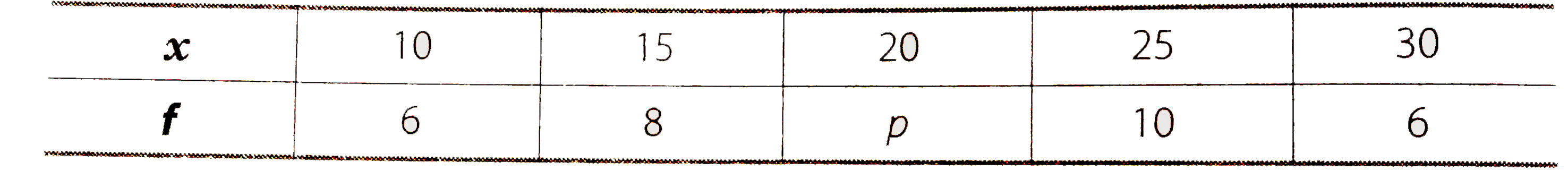
View Solution
