A median and an altitude of an obtuse-angled triangle lie outside the triangle. It is true ?
The correct Answer is:Median does not lie outside, whereas the altidue does.
To determine whether the statement "A median and an altitude of an obtuse-angled triangle lie outside the triangle" is true or false, we can analyze the properties of medians and altitudes in obtuse-angled triangles step by step.
1. Understanding the Obtuse-Angled Triangle:
- An obtuse-angled triangle has one angle that is greater than 90 degrees. Let’s denote the triangle as △ABC where ∠A is the obtuse angle.
2. Defining the Median:
- A median of a triangle is a line segment joining a vertex to the midpoint of the opposite side. For example, if we draw the median from vertex A to the midpoint D of side BC, this median will always lie inside the triangle.
3. Defining the Altitude:
- An altitude of a triangle is a perpendicular line segment from a vertex to the line containing the opposite side. For instance, if we draw the altitude from vertex A to side BC, it must meet side BC at a right angle (90 degrees).
4. Analyzing the Altitude in an Obtuse Triangle:
- In the case of an obtuse triangle, the altitude from the vertex opposite the obtuse angle (vertex A) will not meet side BC at a point within the triangle. Instead, it will extend outside the triangle to meet side BC at a right angle.
5. Conclusion:
- Based on the definitions and properties discussed:
- The median from vertex A to side BC lies inside the triangle.
- The altitude from vertex A to side BC lies outside the triangle.
- Therefore, the statement that "A median and an altitude of an obtuse-angled triangle lie outside the triangle" is false.
Final Answer:
The statement is false.
- An obtuse-angled triangle has one angle that is greater than 90 degrees. Let’s denote the triangle as △ABC where ∠A is the obtuse angle.
- A median of a triangle is a line segment joining a vertex to the midpoint of the opposite side. For example, if we draw the median from vertex A to the midpoint D of side BC, this median will always lie inside the triangle.
- An altitude of a triangle is a perpendicular line segment from a vertex to the line containing the opposite side. For instance, if we draw the altitude from vertex A to side BC, it must meet side BC at a right angle (90 degrees).
- In the case of an obtuse triangle, the altitude from the vertex opposite the obtuse angle (vertex A) will not meet side BC at a point within the triangle. Instead, it will extend outside the triangle to meet side BC at a right angle.
- Based on the definitions and properties discussed:
- The median from vertex A to side BC lies inside the triangle.
- The altitude from vertex A to side BC lies outside the triangle.
- Therefore, the statement that "A median and an altitude of an obtuse-angled triangle lie outside the triangle" is false.
The statement is false.
Topper's Solved these Questions
Similar Questions
State true or false.
(a) The altitude of an acute-angled triangle lies outside the triangle.
(b) the orthocentre of an obtuse-angled triangle lies outside the triangle.
(c) the orthocentre is the point of concurrency of three medians of a triangle.
(d) The vertex containing the right angle is also the orthocentre of a right-angled triangle.
View SolutionFind the altitude of an equilateral triangle of side 8 cm.
View SolutionFind the ara of the given obtuse-angled triangle ABC.
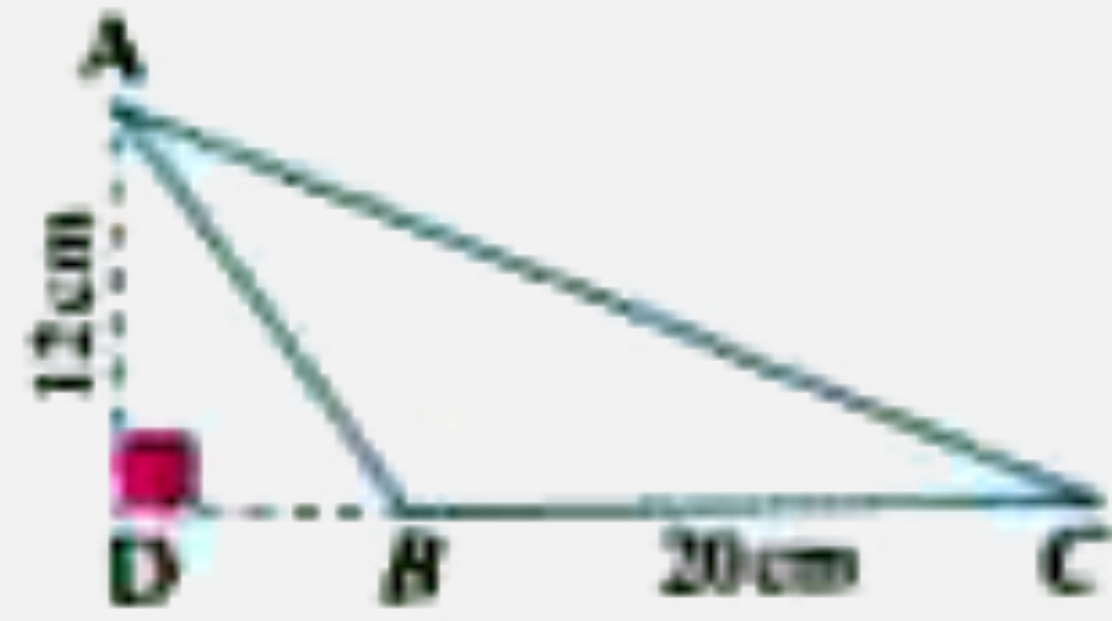
View SolutionIf the altitudes of a triangle are in A.P,then the sides of the triangle are in
View SolutionState true or false.
(a) A median of a triangle may lie outside the triangle.
(b). The medians of a triangle meet at more than one points.
(c) The centroid of a triangle always lies inside the triangle.
View SolutionBy giving a counter example, show that the following statement is not true: p: if all the angles of a triangle are equal, then the triangle is an obtuse angled triangle.
View SolutionIf two sides and an angle of one triangle are equal to two sides and an angle of another triangle , then the two triangles must be congruent’. Is the statement true? Why?
View SolutionIf two sides and an angle of one triangle are equal to two sides and an angle of another triangle , then the two triangles must be congruent’. Is the statement true? Why?
View SolutionCan an equilateral triangle be a, an acute-,b. a right-, and c. an obtuse-angled triangle ? What about isosceles and scalene triangles ?
View SolutionThe points (0,83),(1,3) and (82,30) are the vertices of (A) an obtuse-angled triangle (B) an acute-angled triangle (C) a right-angled triangle (D) none of these
View Solution
