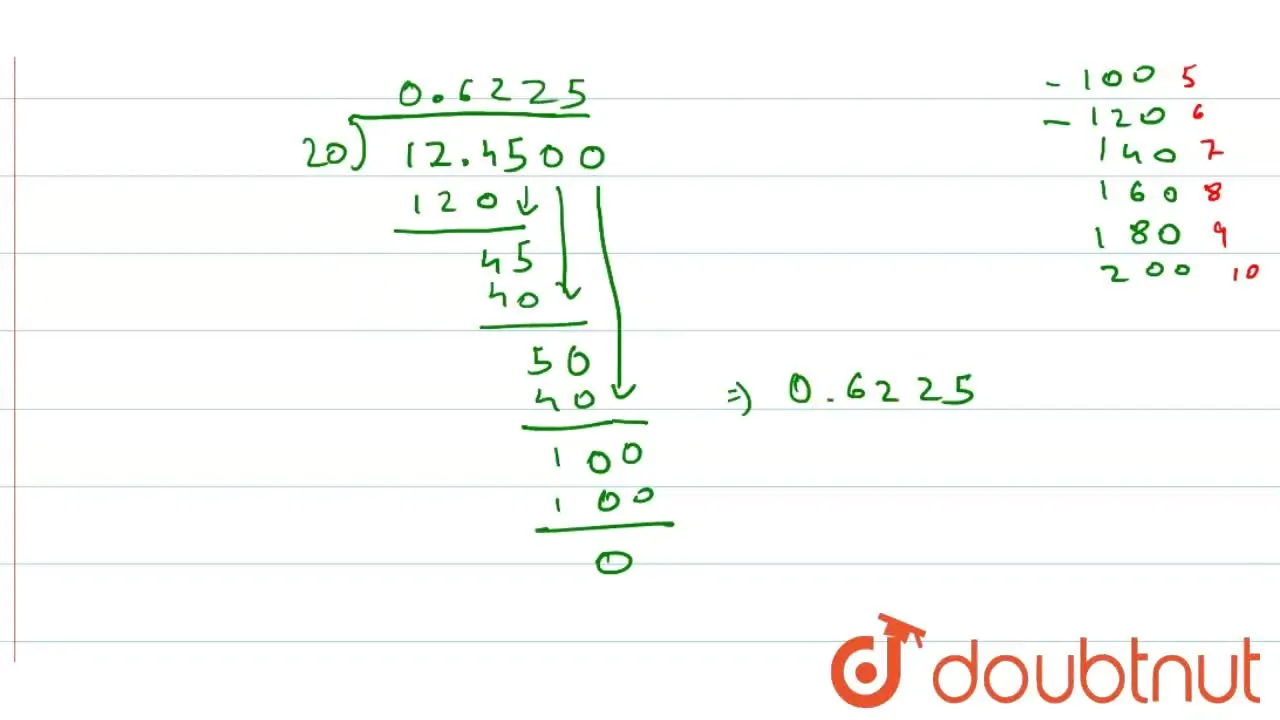
Evaluate:
12.45÷20
Video Solution
Text Solution
The correct Answer is:0.6225
|
Answer
Step by step video, text & image solution for Evaluate: 12.45 div 20 by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023
Related Playlists
- BOOK - ICSECHAPTER - DECIMALS EXERCISE - EXERCISE 3.319videos
DECIMALS
- BOOK - ICSECHAPTER - DECIMALS EXERCISE - REVISION EXERCISE60videos
DECIMALS
- BOOK - ICSECHAPTER - DECIMALS EXERCISE - EXERCISE 3.127videos
DECIMALS
- BOOK - ICSECHAPTER - DATA HANDLING EXERCISE - EXERCISE 24D13videos
DATA HANDLING
- BOOK - ICSECHAPTER - EXPONENTS EXERCISE - EXERCISE 530videos
EXPONENTS
Similar Questions
Evaluate: 1.458÷6
View SolutionEvaluate:
162.5÷5View SolutionEvaluate:
2.145÷5View SolutionEvaluate:
16.50÷132View SolutionEvaluate:
24.55÷20View SolutionEvaluate:
2.568÷12View SolutionEvaluate:
12.18÷28View SolutionEvaluate:
32.54÷10View SolutionEvaluate:
175.44÷12View SolutionEvaluate:
15.848÷40View Solution