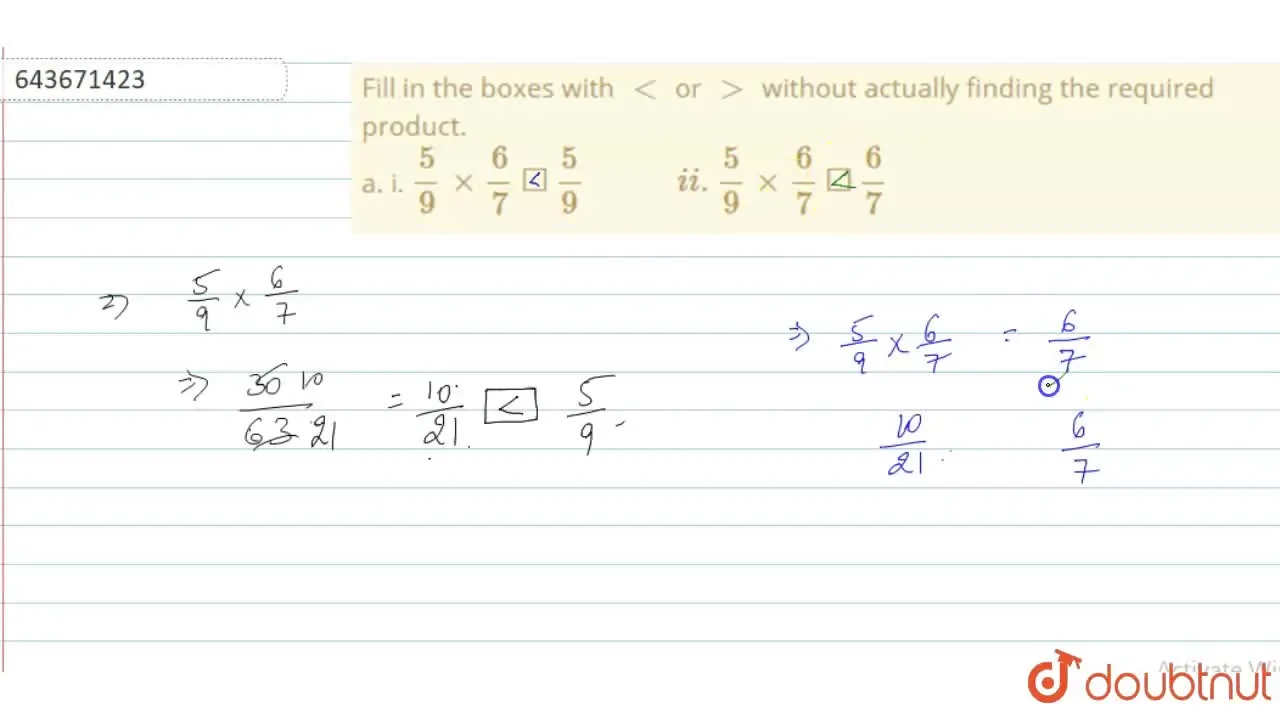
Fill in the boxes with < or > without actually finding the required product.
a. i. 59×67□59 ii.59×67□67
The correct Answer is:i. < ii. <
Step by step video, text & image solution for Fill in the boxes with lt or gt without actually finding the required product. a. i. (5)/(9) xx (6)/(7) square (5)/(9)" "ii. (5)/(9) xx (6)/(7) square (6)/(7) by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Related Playlists
- BOOK - ICSECHAPTER - FRACTIONSEXERCISE - Try This37videos
FRACTIONS
- BOOK - ICSECHAPTER - FRACTIONSEXERCISE - Warm-up Exercise24videos
FRACTIONS
- BOOK - ICSECHAPTER - FRACTIONSEXERCISE - EXERCISE 3B30videos
FRACTIONS
- BOOK - ICSECHAPTER - EXPONENTS AND POWERSEXERCISE - Unit practice paper -17videos
EXPONENTS AND POWERS
- BOOK - ICSECHAPTER - FUNDAMENTAL GEOMETRICAL CONCEPTSEXERCISE - Exercise14videos
FUNDAMENTAL GEOMETRICAL CONCEPTS
Similar Questions
Fill in the boxes with < or > without actually finding the required product.
c. i.95×43□95 ii.95×43□43View SolutionFill in the boxes with < or > without actually finding the required product.
b. i.74×25□74 ii.74×25□25View SolutionCompare the following without evaluating the product:
a. i. 35×79□35 ii.35×79□79View SolutionFill in < or > sign in the boxes without actually carrying out the multiplication.
a. i. 311×29□29 ii.311×29□311View SolutionFill in < or > sign in the boxes without actually carrying out the multiplication.
b. i. 95×813□95 ii.95×813□813View SolutionCompare the following without evaluating the product:
b. i. 127×85□125 ii.127×85□85View SolutionFill in < or > sign in the boxes without actually carrying out the multiplication.
i. 113×65□113 113×65□65View SolutionFind: c. 579×6
View SolutionFill in the box with >,<or= signs,
1:12□2:9View SolutionFind the square root of:
(i) 279
(ii) 4.41View Solution