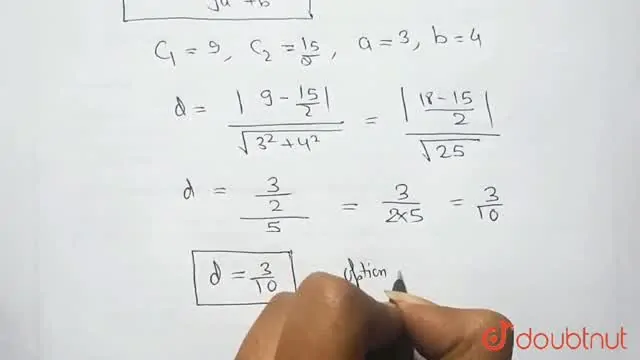The distance between the lines 3x + 4y = 9 and 6x + 8y = 15 is :
2
The correct Answer is:B
Step by step video, text & image solution for The distance between the lines 3x + 4y = 9 and 6x + 8y = 15 is : by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - ICSECHAPTER - MODEL TEST PAPER - 20 EXERCISE - SECTION - B10videos
MODEL TEST PAPER - 20
- BOOK - ICSECHAPTER - MODEL TEST PAPER - 20 EXERCISE - SECTION - C10videos
MODEL TEST PAPER - 20
- BOOK - ICSECHAPTER - MODEL TEST PAPER - 17EXERCISE - SECTION -C10videos
MODEL TEST PAPER - 17
- BOOK - ICSECHAPTER - MODEL TEST PAPER - 9 EXERCISE - SECTION - C9videos
MODEL TEST PAPER - 9
Similar Questions
The distance between the lines 4x+3y=11 and 8x+6y=15 is
View SolutionThe distance between the lines 4x+3y=11 and 8x+6y=15 is
View SolutionDistance between the lines 3x+4y−5=0 and 6x+8y−45=0 is
View SolutionFind the perpendicular distance between the lines 3x+4y+9=0 and to 6x+8y+15=0 is
View SolutionThe perpendicular distance between the lines 3x+4y=6 and 3x+4y+4=0 is :
View SolutionThe angle between the lines 2x=3y=−zand6x=−y=−4x is
View SolutionThe ratio in which the line 3x+4y+2=0 divides the distance between the lines 3x+4y+5=0 and 3x+4y−5=0
View SolutionFind the distance between the parallel lines 3x+4y+7=0and3x+4y+5=0.
View SolutionFind the distance between the parallel lines 3x+4y+7=0and3x+4y+5=0.
View SolutionThe distance between the parallel lnes y=2x+4and6x−3y−5 is (A) 1 (B) 17√3 (C) 7√515 (D) 3√515
View Solution