P and Q are centres of circles of radii 9 cm and 2 cm respectively. PQ = 17 cm. R is the centre of a circle of radius x cm which touches the above circles externally. Given that ∠PRQ=90∘ , write an equation in x and solve it.
The correct Answer is:x2+11x−102=0andx=6
To solve the problem step by step, we will follow the given information and apply the Pythagorean theorem.
Step 1: Understand the given information
We have:
- Circle with center P has a radius of 9 cm.
- Circle with center Q has a radius of 2 cm.
- Distance PQ = 17 cm.
- R is the center of a circle with radius x cm that touches both circles externally.
- Angle PRQ = 90°.
Step 2: Set up the triangle PQR
In triangle PQR, we know that:
- PR = radius of circle at P + radius of circle at R = 9 + x.
- RQ = radius of circle at Q + radius of circle at R = 2 + x.
- PQ = 17 cm.
Step 3: Apply the Pythagorean theorem
According to the Pythagorean theorem:
PQ2=PR2+RQ2
Substituting the values we have:
172=(9+x)2+(2+x)2
Step 4: Calculate PQ2
Calculating 172:
172=289
Step 5: Expand PR2 and RQ2
Now we expand (9+x)2 and (2+x)2:
(9+x)2=81+18x+x2
(2+x)2=4+4x+x2
Step 6: Combine the equations
Now substituting back into the equation:
289=(81+18x+x2)+(4+4x+x2)
Combine like terms:
289=81+4+18x+4x+2x2
289=85+22x+2x2
Step 7: Rearrange the equation
Rearranging gives:
2x2+22x+85−289=0
2x2+22x−204=0
Step 8: Simplify the equation
Dividing the entire equation by 2:
x2+11x−102=0
Step 9: Factor the quadratic equation
Now we need to factor the quadratic equation:
We are looking for two numbers that multiply to -102 and add to 11. The numbers are 17 and -6.
So we can write:
(x+17)(x−6)=0
Step 10: Solve for x
Setting each factor to zero gives us:
1. x+17=0 → x=−17 (not valid since radius cannot be negative)
2. x−6=0 → x=6
Thus, the radius x of the circle centered at R is 6 cm.
Final Answer
The value of x is 6 cm.
---
We have:
- Circle with center P has a radius of 9 cm.
- Circle with center Q has a radius of 2 cm.
- Distance PQ = 17 cm.
- R is the center of a circle with radius x cm that touches both circles externally.
- Angle PRQ = 90°.
In triangle PQR, we know that:
- PR = radius of circle at P + radius of circle at R = 9 + x.
- RQ = radius of circle at Q + radius of circle at R = 2 + x.
- PQ = 17 cm.
According to the Pythagorean theorem:
PQ2=PR2+RQ2
172=(9+x)2+(2+x)2
Calculating 172:
172=289
Now we expand (9+x)2 and (2+x)2:
(9+x)2=81+18x+x2
(2+x)2=4+4x+x2
Now substituting back into the equation:
289=(81+18x+x2)+(4+4x+x2)
Combine like terms:
289=81+4+18x+4x+2x2
289=85+22x+2x2
Rearranging gives:
2x2+22x+85−289=0
2x2+22x−204=0
Dividing the entire equation by 2:
x2+11x−102=0
Now we need to factor the quadratic equation:
We are looking for two numbers that multiply to -102 and add to 11. The numbers are 17 and -6.
So we can write:
(x+17)(x−6)=0
Setting each factor to zero gives us:
1. x+17=0 → x=−17 (not valid since radius cannot be negative)
2. x−6=0 → x=6
The value of x is 6 cm.
Topper's Solved these Questions
- MIXED PRACTICE BOOK - ICSECHAPTER - MIXED PRACTICE EXERCISE - SET B
52 Videos
- MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)BOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)EXERCISE - EXERCISE 24 (E)
23 Videos
- PROBABILITYBOOK - ICSECHAPTER - PROBABILITYEXERCISE - EXERCISE 25(C)
106 Videos
Similar Questions
Knowledge Check
The centres of two circles with radii 6 cm and 2 cm are 10 cm apart. Calculate the length of the transverse common tangent.
A5cmB6cmC7cmD2cm
Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
View SolutionTwo concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
View SolutionTwo concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
View SolutionThe radii of two circles are 8 cm and 6 cm respectively. Find the radius of the circle having area equal to the sum of the areas of the two circles.
View SolutionTwo concentric circle of radii 3cm and 5cm are given. Find the chord BC which touches the inner circle at P.
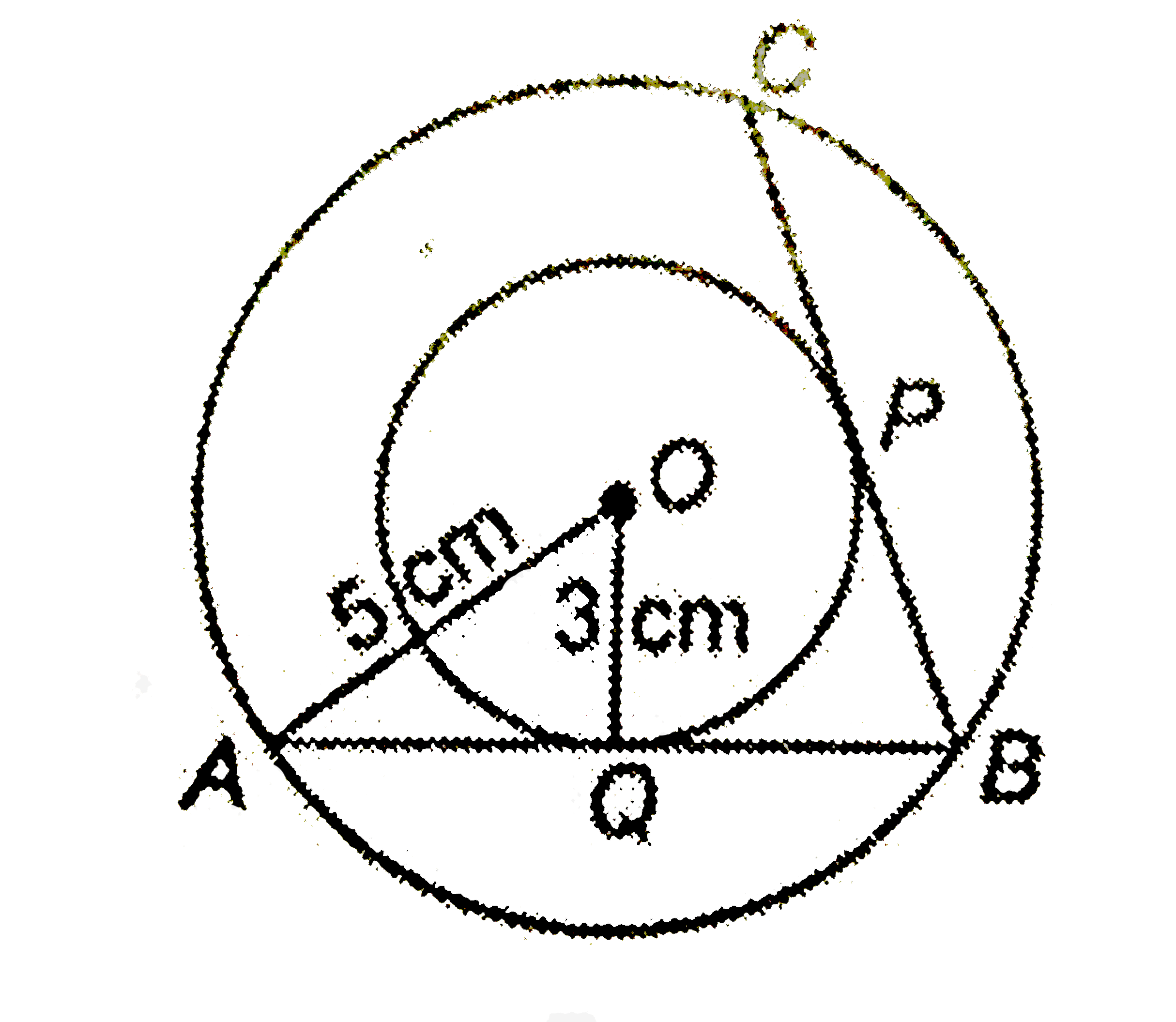
View SolutionThe radii of two circles are 8 cm and 6 cm respectively. Find the radius of the circle having its area equal to the sum of the areas of the two circles.
View SolutionFind the length of a chord which is at a distance of 4 cm from the centre of the circle of radius 6 cm.
View Solution
