Using a graph paper, plot the points A (6, 4)and B(0, 4).
Write the co-ordinates of A' and B'.
The correct Answer is:A' = (-6,-4) , B' = (0,-4)
To solve the problem of plotting points A (6, 4) and B (0, 4) on a graph paper and finding their reflections A' and B', follow these steps:
1. Understanding the Points:
- We have two points: A (6, 4) and B (0, 4).
- The coordinates are in the form (x, y), where x is the horizontal position and y is the vertical position.
2. Plotting Point A (6, 4):
- Locate the x-coordinate (6) on the x-axis.
- From that point, move vertically up to the y-coordinate (4).
- Mark this point as A on the graph paper.
3. Plotting Point B (0, 4):
- Locate the x-coordinate (0) on the x-axis, which is the origin.
- From the origin, move vertically up to the y-coordinate (4).
- Mark this point as B on the graph paper.
4. Finding the Reflections A' and B':
- To find the reflection of point A (6, 4) across the origin:
- Change the signs of both coordinates: A' = (-6, -4).
- To find the reflection of point B (0, 4) across the origin:
- Change the sign of the y-coordinate: B' = (0, -4).
5. Writing the Coordinates of A' and B':
- The coordinates of A' are (-6, -4).
- The coordinates of B' are (0, -4).
Final Answer:
- A' = (-6, -4)
- B' = (0, -4)
- We have two points: A (6, 4) and B (0, 4).
- The coordinates are in the form (x, y), where x is the horizontal position and y is the vertical position.
- Locate the x-coordinate (6) on the x-axis.
- From that point, move vertically up to the y-coordinate (4).
- Mark this point as A on the graph paper.
- Locate the x-coordinate (0) on the x-axis, which is the origin.
- From the origin, move vertically up to the y-coordinate (4).
- Mark this point as B on the graph paper.
- To find the reflection of point A (6, 4) across the origin:
- Change the signs of both coordinates: A' = (-6, -4).
- To find the reflection of point B (0, 4) across the origin:
- Change the sign of the y-coordinate: B' = (0, -4).
- The coordinates of A' are (-6, -4).
- The coordinates of B' are (0, -4).
- A' = (-6, -4)
- B' = (0, -4)
Similar Questions
Using a graph paper, plot the points A (6, 4)and B(0, 4).
Find its perimeter.
View SolutionUsing a graph paper, plot the points A (6, 4)and B(0, 4).
State the geometrical name for the figure ABA'B'.
View SolutionUsing a graph paper, plot the points A (6, 4)and B(0, 4).
Reflect A and B in the origin to get the images A and B'.
View SolutionThe ordinate of a point lying on the line joining the points (6, 4) and (7, -5) is -23. Find the co-ordinates of that point.
View SolutionIf A and B are the points (−3,4) and (3,−4) respectively then the co-ordinates of the point C on AB produced such that AC=3BC are
(A) (0,0)
(B) (−6,8)
(C) (6,−8)
(D) (−6,−8)
View SolutionUse graph paper for this question.
The points A(2, 3), B(4, 5) and C(7, 2) are the vertices of ΔABC
Write down the co-ordinates of A', B', C' if Δ A' B' C' is the image of ΔABC , when reflected in the origin.
View SolutionUse graph paper for this question.
The points A(2, 3), B(4, 5) and C(7, 2) are the vertices of ΔABC
Write down the co-ordinates of A", B", C" if Δ A''B" C" is the image of ΔABC , when reflected in the x-axis.
View SolutionPoint A (4,-1) is reflected as A' in the y - axis . Point B on reflection in the x - axis is mapped as B' (-2,5) . Write the co- ordinates of A' and B.
View SolutionThe mid-point of the A segment AB, as shown in diagram, is C (4, -3). (4, -3) Write down the co ordinates of A and B.
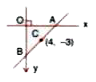
View SolutionThe co-ordinates of two points A and B are (-3, 4) and (2, -1). Find :
the co-ordinates of the point where the line AB intersects the y-axis.
View Solution
