The mass of a molecule of hydrogen is 3.332×10−27 kg. Find the mass of 1 kg mol of hydrogen gas.
To find the mass of 1 kg mole of hydrogen gas, we can follow these steps:
Step 1: Understand the given data
We are given the mass of a single molecule of hydrogen, which is:
Mass of one molecule of hydrogen=3.332×10−27 kg
Step 2: Know Avogadro's number
Avogadro's number, which is the number of molecules in one mole, is approximately:
NA=6.022×1023 molecules/mol
Step 3: Calculate the mass of 1 mole of hydrogen
To find the mass of 1 mole of hydrogen gas, we multiply the mass of one molecule by Avogadro's number:
Mass of 1 mole of hydrogen=NA×Mass of one molecule
Substituting the values:
Mass of 1 mole of hydrogen=(6.022×1023 molecules/mol)×(3.332×10−27 kg/molecule)
Step 4: Perform the multiplication
Calculating the above expression:
Mass of 1 mole of hydrogen=6.022×3.332×1023×10−27
=6.022×3.332×10−4 kg
Step 5: Calculate the numerical value
Now, calculating 6.022×3.332:
6.022×3.332≈20.007 (approximately)
So,
Mass of 1 mole of hydrogen≈20.007×10−4 kg=0.020007 kg
Step 6: Convert to grams
Since 1 kg = 1000 grams, we can convert the mass into grams:
0.020007 kg=20.007 grams
Final Answer
Thus, the mass of 1 kg mole of hydrogen gas is approximately:
Mass of 1 kg mole of hydrogen gas≈2 grams
We are given the mass of a single molecule of hydrogen, which is:
Mass of one molecule of hydrogen=3.332×10−27 kg
Avogadro's number, which is the number of molecules in one mole, is approximately:
NA=6.022×1023 molecules/mol
To find the mass of 1 mole of hydrogen gas, we multiply the mass of one molecule by Avogadro's number:
Mass of 1 mole of hydrogen=NA×Mass of one molecule
Substituting the values:
Mass of 1 mole of hydrogen=(6.022×1023 molecules/mol)×(3.332×10−27 kg/molecule)
Calculating the above expression:
Mass of 1 mole of hydrogen=6.022×3.332×1023×10−27
=6.022×3.332×10−4 kg
Now, calculating 6.022×3.332:
6.022×3.332≈20.007 (approximately)
So,
Mass of 1 mole of hydrogen≈20.007×10−4 kg=0.020007 kg
Since 1 kg = 1000 grams, we can convert the mass into grams:
0.020007 kg=20.007 grams
Thus, the mass of 1 kg mole of hydrogen gas is approximately:
Mass of 1 kg mole of hydrogen gas≈2 grams
Topper's Solved these Questions
- MEASUREMENTS AND EXPERIMENTATION BOOK - ICSECHAPTER - MEASUREMENTS AND EXPERIMENTATION EXERCISE - EXERCISE 1(A)
51 Videos
- MEASUREMENTS AND EXPERIMENTATION BOOK - ICSECHAPTER - MEASUREMENTS AND EXPERIMENTATION EXERCISE - EXERCISE 1(A) (Multiple Choice type)
5 Videos
- MAGNETISM BOOK - ICSECHAPTER - MAGNETISM EXERCISE - EXERCISE-10(B) (Multiple Choice Type)
2 Videos
- MOTION IN ONE DIMENSION BOOK - ICSECHAPTER - MOTION IN ONE DIMENSION EXERCISE - EXERCISE -2 (C) ( Multiple choice type :)
17 Videos
Similar Questions
The mass of a hydrogen is ..........kg.
View SolutionThe average mass of an atom of uranium is 3.9×10−25 kg. Find the number of atom is 1 g of uranium.
View Solution(a) Calculate (i) root-mean-square speed and (ii) the mean energy of 1 mol of hyderogen at STP given that density of hydrogen is 0.09kg/m3. (b) Given that the mass of a molecule of hydergen is 3.34×10−27 kg, calculate Avogadro's number. (c ) Calculate Boltmann's constant.
View SolutionThe mass of hydrogen molecule is 3.32×10−27 kg. If 1023 hydrogen molecules strikes per second at 2 cm2 area of a rigid wall at an angle of 45∘ from the normal and rebound back with a speed of 1000 ms−1, then the pressure exerted on the wall is
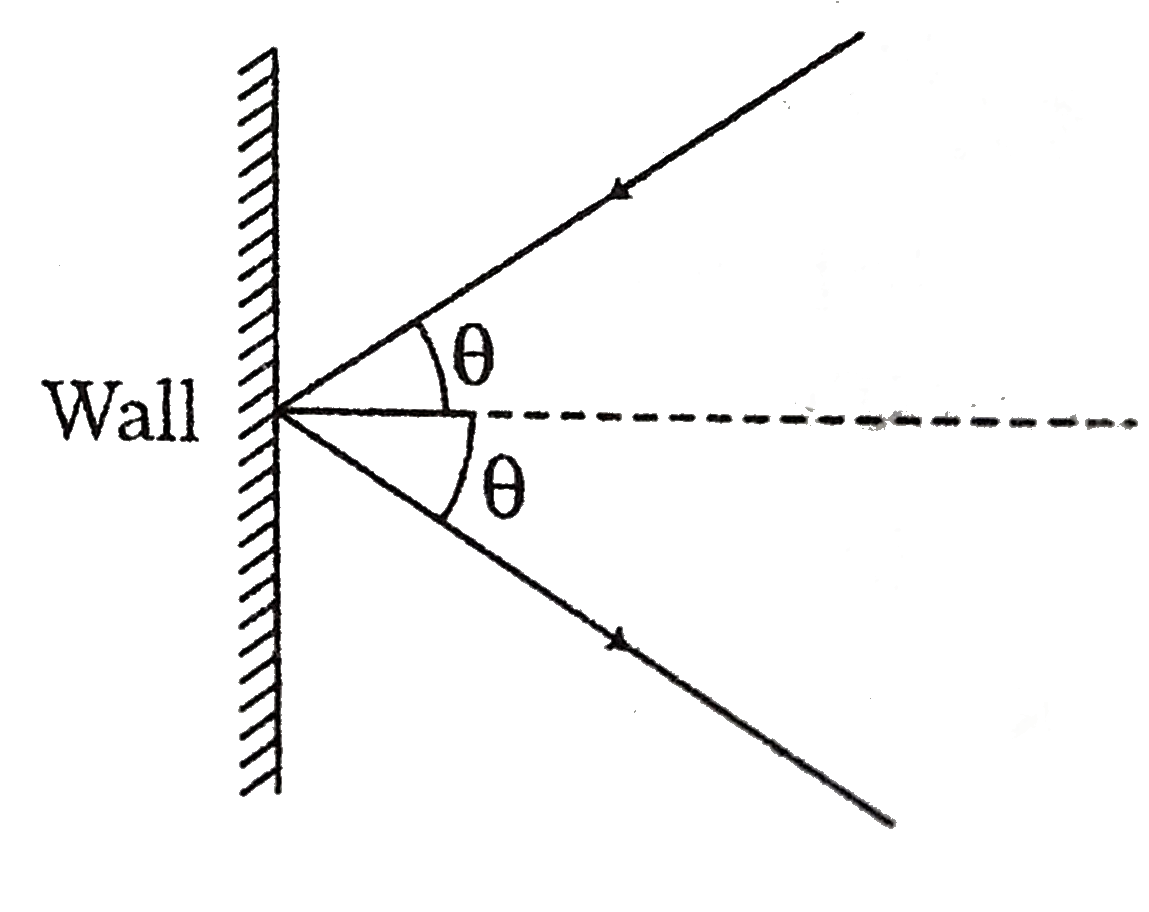
View SolutionA flask contains 10−3m3 gas. At a temperature, the number of molecules of oxygen at 3.0×1022. The mass of an oxygen molecule is 5.3×10−26 kg and at that temperature the rms velocity of molecules is 400m/s. The pressure in N/m2 of the gas in the flask is ..............
View SolutionUsing the fact that hydrogen gas consister of diatomic molecules with M=2kg/K−mol. Find the speed of sound in hydrogen at 27∘C .
View SolutionA vessel of volume 2×10−2m3 contains a mixture of hydrogen and helium at 47∘C temperature and 4.15×105N/m2 Pressure. The mass of the mixture is 10−2kg. Calculate the masses of hydrogen and helium in the given mixture.
View SolutionA vessel of volume 2×10−2m3 contains a mixture of hydrogen at 47∘C temperature and 4.15×105N/m2 Pressure. The mass of the mixture is 10−2kg. Calculate the masses of hydrogen and helium in the given mixture.
View SolutionIf the mass of neutron is 1.7×10−27kg, then the de Broglie wavelength of neutron of energy 3eV is (h=6.6×10−34J.s)
View SolutionThe mass to charge ratio (m/e) for a cation 1.5×10−8 kg/C. What is the mass of this cation?
View Solution
