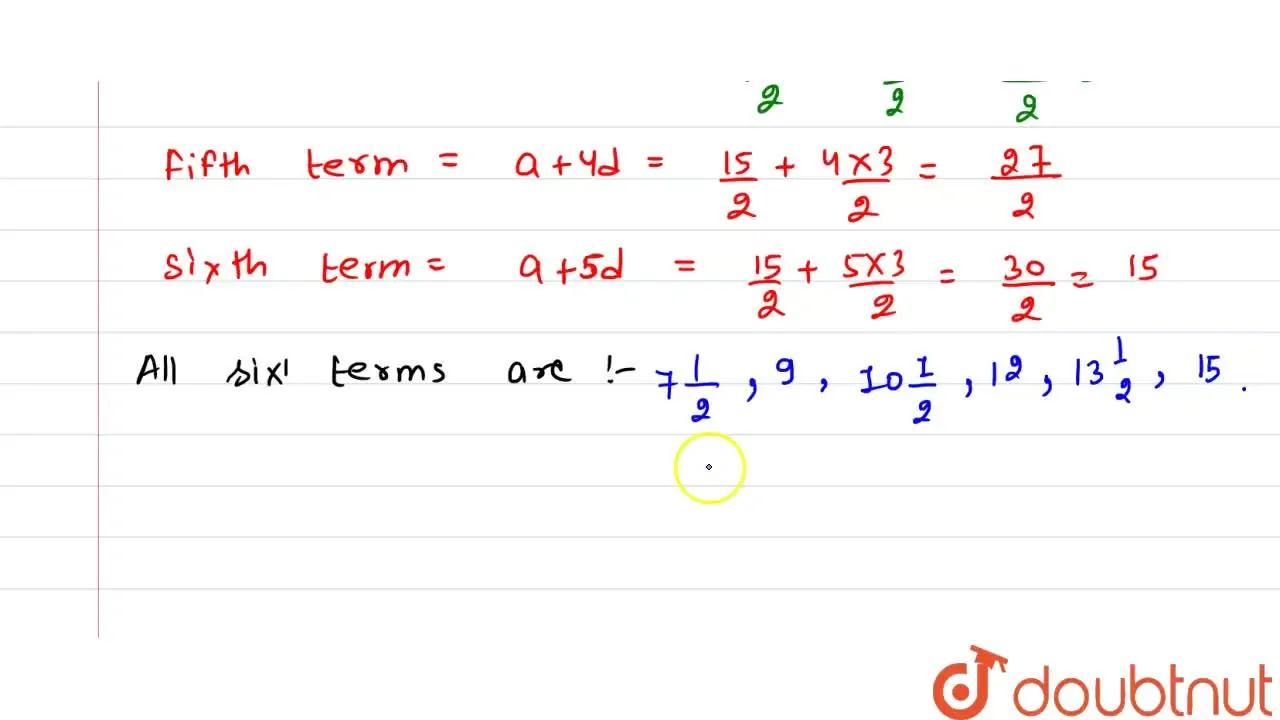
Write the first six terms of an A.P. in which
a=712,d=112
The correct Answer is:712,9,1012,12,1312,15
Step by step video, text & image solution for Write the first six terms of an A.P. in which a= 7 (1)/(2) , d= 1 (1)/(2) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - ICSECHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE 14 (c)32videos
SEQUENCE AND SERIES
- BOOK - ICSECHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE 14 (d)22videos
SEQUENCE AND SERIES
- BOOK - ICSECHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE 14 (a)13videos
SEQUENCE AND SERIES
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 5EXERCISE - SECTION C11videos
SELF ASSESSMENT PAPER 5
- BOOK - ICSECHAPTER - SEQUENCES AND SERIESEXERCISE - MULTIPLE CHOICE QUESTIONS34videos
SEQUENCES AND SERIES
Similar Questions
Write the first six terms of an A.P. in which
a=5 ,d =4View SolutionWrite the first six terms of an A.P. in which
a=98 , d=-3View SolutionWrite the first six terms of an A.P. in which
a=x ,d = 3x +2View SolutionDetermine the sum of first 35 terms of an A.P. if t2, = 1 and t7 , = -22.
View SolutionFind the sum of first 20 terms of an A.P., in which 3rd terms in 7 and 7th term is tow more than thrice of its 3rd term.
View SolutionDetermine the sum of the first 35 terms of an A.P. if a2,=2 and a7,=22.
View SolutionAssertion: The number of terms to be taken in the A.P.9, 17, 25, ... So as to make a sum of 636 is 13.
Reason: The sum of first n terms of an A.P. is given by n2[2a+(n−1)d].View SolutionWrite the next two terms of the A.P. : 3, -1, -5, ... .
View SolutionThe 2nd, 31st and last term of an A.P. are 734,12 and −612 respectively. Find the first term and the number of terms.
View SolutionFind the sum of 50 terms of the A.P. 1 + 4 + 7 + ....
View Solution