There are 12 true - false questions in an examination .How many seqences of answer are possible?
The correct Answer is:4096
To solve the problem of how many sequences of answers are possible for 12 true-false questions, we can follow these steps:
1. Understanding the Problem: Each question in the examination can be answered in one of two ways: True (T) or False (F).
2. Identifying the Choices: For each of the 12 questions, there are 2 choices (True or False).
3. Calculating Total Sequences: Since the choices for each question are independent of each other, the total number of sequences of answers can be calculated using the multiplication principle of counting.
- For the first question, there are 2 choices.
- For the second question, there are also 2 choices.
- This continues for all 12 questions.
4. Using Exponents: The total number of sequences can be expressed as:
Total Sequences=2×2×2×…(12 times)=212
5. Calculating 212: Now we calculate 212:
212=4096
6. Final Answer: Therefore, the total number of sequences of answers possible for the 12 true-false questions is 4096.
Summary:
The total number of sequences of answers for 12 true-false questions is 4096.
---
- For the second question, there are also 2 choices.
- This continues for all 12 questions.
Total Sequences=2×2×2×…(12 times)=212
212=4096
The total number of sequences of answers for 12 true-false questions is 4096.
Similar Questions
There are 4 multiple choice questions in an examination. How many sequences of answers are possible, if each question has 2 choices?
View SolutionThere are 6 multiple choice questions in an examination. How many sequences of answers are possible, if the first three questions have 4 choices each and the next three he 2 each?
View SolutionThere are 6 multiple choice questions in an examination. How many sequence of answers are possible, if the first three questions have 4 choices each and the next three have 5 each?
View SolutionThere are 10 true-false questions in an examination. Then these questions can be answered in
View SolutionHow many stereoisomers are possible for
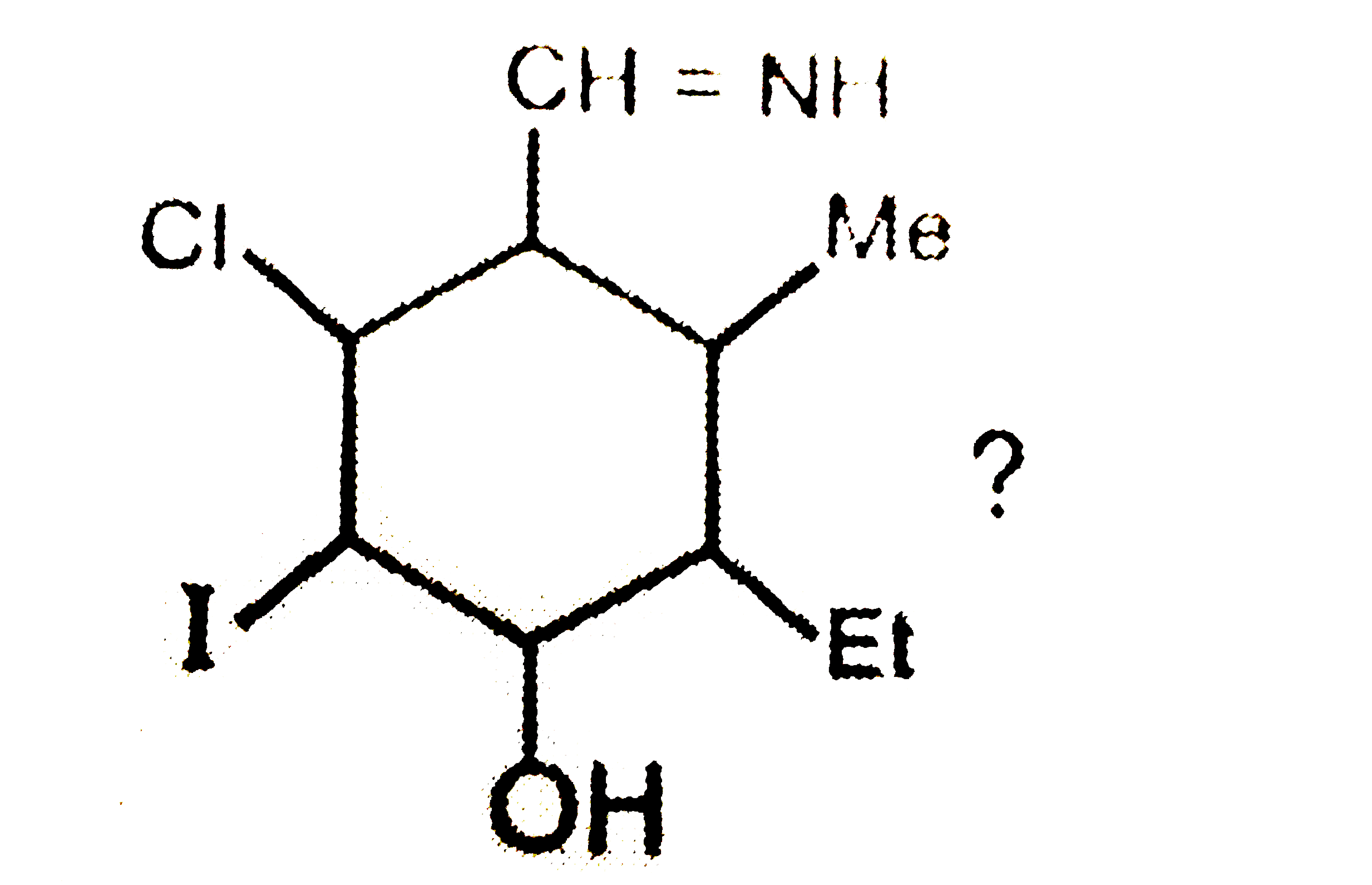
View SolutionHow many structures for F are possible
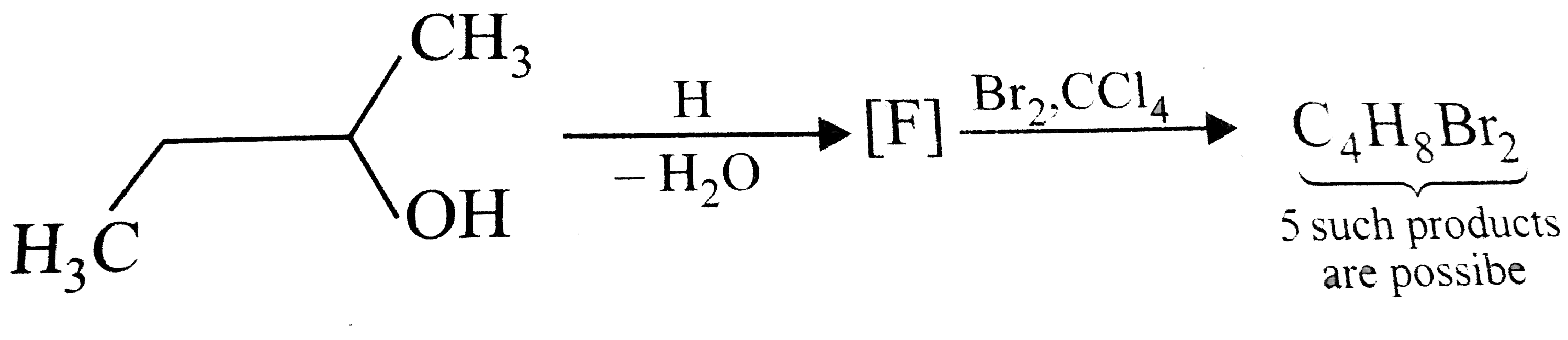 .
.
View SolutionHow many geometrical isomers are possible for

View SolutionHow many tetramethyl benzene are possible:
View Solution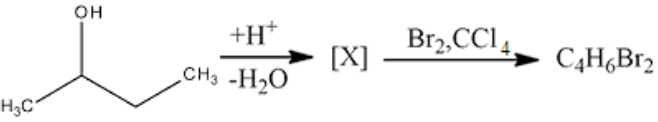
How many structures of X is possible ?
View SolutionThere are 30 objective-type questions in an examination. For every correct answer, a student scores 5 marks and for very incorrect answer, 3 marks are deducted. If a student attempts all the questions and scores 94 marks, find how many questions he answered correctly.
View Solution
