A pendulum is oscillaring on either side its rest position.The correct statement is:
It has the maximum potential energy at its extreme position.
It has the maximum kinetic energy at its extreme position.
it has the maximum potential energy at its mean position.
The sum of its kinetic and potential energies remains constant throughout the motion.
The correct Answer is:D
To solve the question regarding the pendulum oscillating around its rest position, we will analyze the statements provided and determine which one is correct.
Step 1: Understand the motion of a pendulum
A pendulum swings back and forth around a central rest position. As it moves, it converts energy between kinetic energy (due to its motion) and potential energy (due to its height above the lowest point).
Step 2: Analyze the energy at different positions
1. At the extreme positions (the highest points of the swing):
- The pendulum has maximum potential energy because it is at its highest point.
- The kinetic energy is zero because the pendulum momentarily stops before changing direction.
2. At the mean position (the lowest point of the swing):
- The pendulum has maximum kinetic energy because it is moving the fastest at this point.
- The potential energy is zero because it is at the lowest height.
Step 3: Evaluate the statements
1. Maximum potential energy at extreme position: This statement is true.
2. Maximum kinetic energy at extreme position: This statement is false.
3. Maximum potential energy at mean position: This statement is false.
4. Sum of kinetic and potential energy remains constant: This statement is true according to the law of conservation of mechanical energy.
Step 4: Conclusion
The correct statements regarding the pendulum's motion are:
- It has maximum potential energy at its extreme position.
- The sum of the kinetic and potential energy remains constant throughout the motion.
However, since the question asks for the correct statement, the most comprehensive and relevant answer is: The sum of the kinetic and potential energy remains constant throughout the motion.
Final Answer
The correct statement is: The sum of the kinetic and potential energy remains constant throughout the motion.
---
A pendulum swings back and forth around a central rest position. As it moves, it converts energy between kinetic energy (due to its motion) and potential energy (due to its height above the lowest point).
1. At the extreme positions (the highest points of the swing):
- The pendulum has maximum potential energy because it is at its highest point.
- The kinetic energy is zero because the pendulum momentarily stops before changing direction.
- The pendulum has maximum kinetic energy because it is moving the fastest at this point.
- The potential energy is zero because it is at the lowest height.
1. Maximum potential energy at extreme position: This statement is true.
2. Maximum kinetic energy at extreme position: This statement is false.
3. Maximum potential energy at mean position: This statement is false.
4. Sum of kinetic and potential energy remains constant: This statement is true according to the law of conservation of mechanical energy.
The correct statements regarding the pendulum's motion are:
- It has maximum potential energy at its extreme position.
- The sum of the kinetic and potential energy remains constant throughout the motion.
The correct statement is: The sum of the kinetic and potential energy remains constant throughout the motion.
Topper's Solved these Questions
Similar Questions
A pendulum is oscillating on either side of its rest position. Explain the energy changes that take place in the Oscillating pendulum. How does mechanical energy remain constant in it ? Draw the necessary diagram.
View SolutionA pendulum with bob of mass is scillating on either side from its resting position A between the extremes B and Cala vertical height above A. What is the kinetic energy K and potential energy U when the pendulum is al positions (i) A. (ii) B. and (in C?
View Solution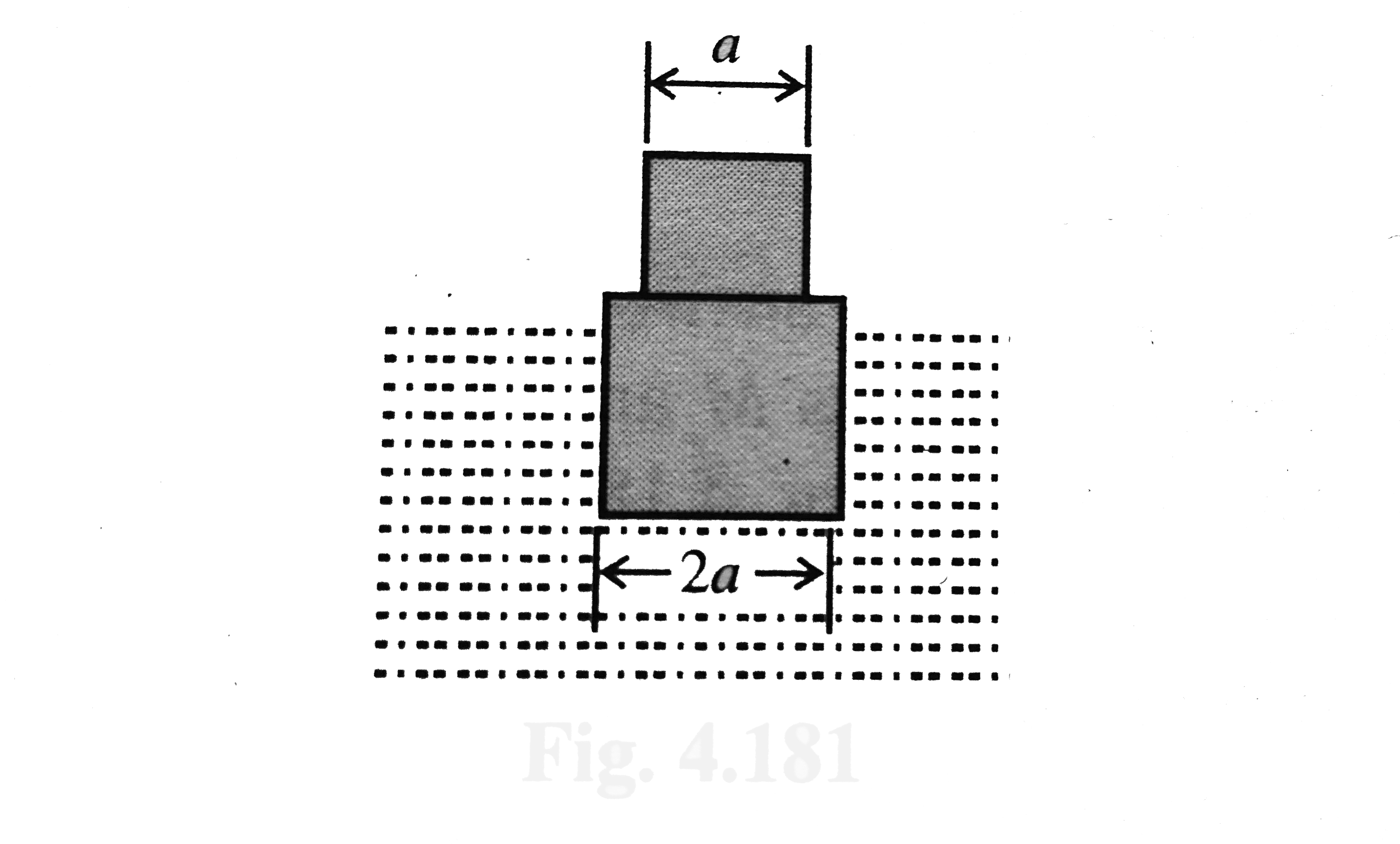
Q. Statement I: Two cubical blocks of same material and of sides a and 2a, respectively are attached rigidley and symmetrically to each other as shown. The system of two blocks is floating in water in such a way that upper surface of bigger blocks is just submerged in the water . If the system of blocks is displaced slightly in vertical directions, then the amplitude of oscillation on either side of equilibrium position would be different. Statement II: The force constant on two sides of equilibrium position in the above described situation is different.
View SolutionA pendulum has a period T for small oscillations. An obstacle is placed directly beneath the pivot, so that only the lowest one - quarter of the string can follow the pendulum bob when it swings to the left of its resting position. The pendulum is released from rest at a certain point. How long will it take to return to that point again ? In answering this question, you may assume that the angle between the moving string and the vertical stays small throughout the motion.

View SolutionA pendulum is displaced to an angle θ from its equilibrium position, then it will pass through its mean position with a velocity v equal to
View SolutionA positively charged pendulum is oscillating in a uniform electric field as shown in Figure. Its time period of SHM as compared to that when it was uncharged. (mg>qE)

View SolutionA simple pendulum is released from rest with the string in horizontal position. The vertical component of the velocity of the bob becomes maximum, when the string makes an angle θ with the vertical. The angle θ is equal to
View SolutionA pendulum bob has a speed 3m/s while passing through its lowest position. What is its speed when it makes an angle of 600 with the vertical? The length of the pendulum is 0.5m Take g=10ms2.
View SolutionA simple pendulum swings with angular amplitude θ. The tension in the string when it is vertical is twice the tension in its extreme position. Then, cosθ is equal to
View SolutionA simple pendulum is suspended from the ceiling of a car and its period of oscillation is T when the car is at rest. The car starts moving on a horizontal road with a constant acceleration g (equal to the acceleration due to gravity, in magnitude) in the forward direction. To keep the time period same, the length of th pendulum
View Solution
