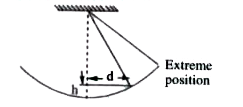
A simple pendulum ,while oscillating ,rises to a maximum vertical height of 5 cm from its rest position when it reaches to its extreme position on one side.If mass of the bob of the simple pendulum is 500 g and g-10 m s−2 find :
(i)the total energy of simple pendulum at any instant while oscillating ,and
(ii)the velocity of bob at its mean position State the assumption made in your calculation .
To solve the problem step-by-step, we will first calculate the total energy of the simple pendulum and then find the velocity of the bob at its mean position.
Step 1: Calculate the Total Energy of the Simple Pendulum
The total mechanical energy (E) of a simple pendulum in the absence of friction is equal to the gravitational potential energy (PE) at its maximum height. The formula for gravitational potential energy is:
PE=m⋅g⋅h
Where:
- m = mass of the bob (in kg)
- g = acceleration due to gravity (in m/s²)
- h = maximum height (in meters)
Given:
- Mass of the bob, m=500g=0.5kg
- g=10m/s2
- Maximum height, h=5cm=0.05m
Now substituting the values into the formula:
PE=0.5kg⋅10m/s2⋅0.05m
Calculating this gives:
PE=0.5⋅10⋅0.05=0.25J
Thus, the total energy of the simple pendulum at any instant while oscillating is:
Total Energy=0.25J
Step 2: Calculate the Velocity of the Bob at its Mean Position
At the mean position, all the potential energy is converted into kinetic energy (KE). The formula for kinetic energy is:
KE=12mv2
At the mean position, the kinetic energy is equal to the potential energy at the maximum height:
12mv2=mgh
We can cancel m from both sides (assuming m≠0):
12v2=gh
Now, solving for v:
v2=2gh
Taking the square root:
v=√2gh
Substituting the values of g and h:
v=√2⋅10⋅0.05
Calculating this gives:
v=√1=1m/s
Final Answers
(i) The total energy of the simple pendulum at any instant while oscillating is 0.25J.
(ii) The velocity of the bob at its mean position is 1m/s.
Assumption Made
The assumption made in our calculations is that there is no energy loss due to friction or air resistance.
- m = mass of the bob (in kg)
- g = acceleration due to gravity (in m/s²)
- h = maximum height (in meters)
- Mass of the bob, m=500g=0.5kg
- g=10m/s2
- Maximum height, h=5cm=0.05m
The assumption made in our calculations is that there is no energy loss due to friction or air resistance.
Topper's Solved these Questions
- WORK ENERGY AND POWERBOOK - ICSECHAPTER - WORK ENERGY AND POWEREXERCISE - EXERCISE-2(A)
31 Videos
- WORK ENERGY AND POWERBOOK - ICSECHAPTER - WORK ENERGY AND POWEREXERCISE - EXERCISE-2(A) (MULTIPLE CHOICE TYPE )
2 Videos
- CALORIMETRYBOOK - ICSECHAPTER - CALORIMETRYEXERCISE - EXERCISE-11(B) (NUMERICALS)
15 Videos
Similar Questions
Knowledge Check
A simple pendulum is oscillating in a stationery lift. When the lift falls freely, the frequency of oscillations of the pendulum is
AzeroBυC2υDinfinite
A simple pendulum completes 40 oscillations in one minute. Find its time period .
View SolutionA simple pendulum swings with angular amplitude θ. The tension in the string when it is vertical is twice the tension in its extreme position. Then, cosθ is equal to
View Solutionwhen the bob of a simple pendulum swings, the work done by tension in the string is :
View SolutionIn a simple pendulum the period of oscillation (T) is related to the length of the pendulum (L) as
View SolutionA simple pendulum of length 1 oscillates aboμt the mean position as shown in the figure. If the total energy of the pendulum is E, the velocity of the pendulum bob of mass m at point P is:
View SolutionIt takes 0.2 s for a pendulum bob to move from the mean position to one end. What is the time period of the pendulum?
View SolutionWhat is the period of oscillation of a simple pendulum if its bob is made of ice ?
View Solution
