O and C are respectively orthocentre and circumcentre of a ΔPQR. P and O joined and produced to meet side QR at S. ∠PQS=60∘,∠QCR=130∘. Find ∠RPS.
30
35
40
45
The correct Answer is:35∘
To solve the problem step-by-step, we will use the properties of triangles and the relationships between angles formed by the orthocenter and circumcenter.
Step 1: Understand the Given Information
- We have triangle PQR with orthocenter O and circumcenter C.
- ∠PQS=60∘
- ∠QCR=130∘
Step 2: Use the Property of the Circumcenter
Since C is the circumcenter, the angle ∠QCR subtended by chord QR at the circumcenter is twice the angle subtended at any point on the circumference. Thus, we can find ∠QPR:
∠QPR=12×∠QCR=12×130∘=65∘
Step 3: Use the Property of the Orthocenter
Since O is the orthocenter, the angle ∠PQR can be found using the fact that the angles in triangle PQR sum up to 180∘:
∠PQR+∠QRP+∠QPR=180∘
Let ∠QRP=x. Thus,
60∘+x+65∘=180∘
x=180∘−125∘=55∘
So, ∠QRP=55∘.
Step 4: Find ∠RPS
Now, we can find ∠RPS using the relationship:
∠RPS=∠QPR−∠PQS
Substituting the known values:
∠RPS=65∘−60∘=5∘
Final Answer
Thus, the angle ∠RPS is 5∘.
---
- We have triangle PQR with orthocenter O and circumcenter C.
- ∠PQS=60∘
- ∠QCR=130∘
Since C is the circumcenter, the angle ∠QCR subtended by chord QR at the circumcenter is twice the angle subtended at any point on the circumference. Thus, we can find ∠QPR:
∠QPR=12×∠QCR=12×130∘=65∘
Since O is the orthocenter, the angle ∠PQR can be found using the fact that the angles in triangle PQR sum up to 180∘:
∠PQR+∠QRP+∠QPR=180∘
Let ∠QRP=x. Thus,
60∘+x+65∘=180∘
x=180∘−125∘=55∘
So, ∠QRP=55∘.
Now, we can find ∠RPS using the relationship:
∠RPS=∠QPR−∠PQS
Substituting the known values:
∠RPS=65∘−60∘=5∘
Thus, the angle ∠RPS is 5∘.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
O and C are respectively orthocentre and circumcentre of a ΔPQR. P and O joined and produced to meet side QR at S. ∠PQS=60∘,∠QCR=130∘. Find ∠RPS.
A30∘B35∘C100∘D60∘ - Question 2 - Select One
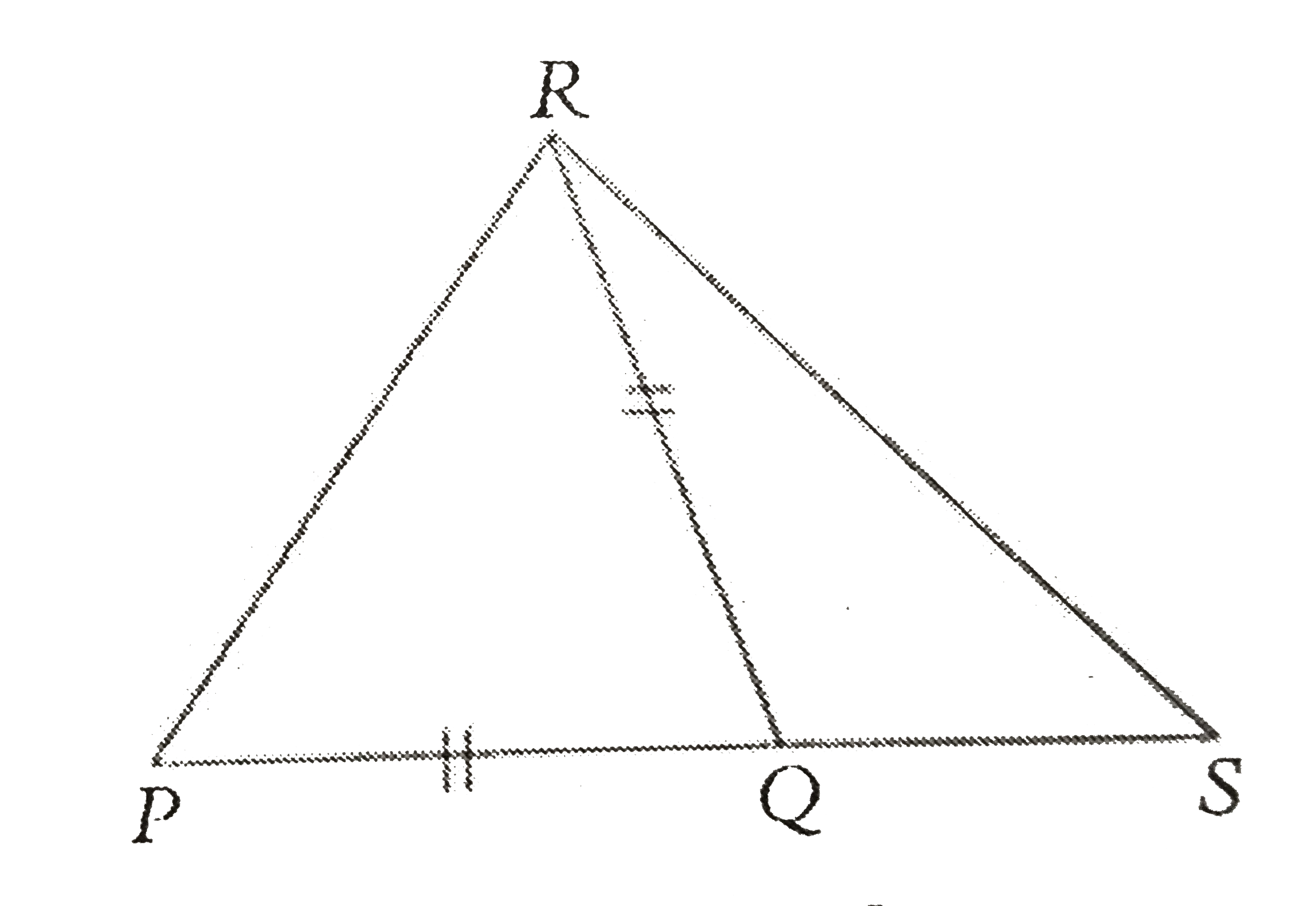
In the figure, PQ=QR,∠RPQ=60∘,and∠QRS=20∘. Find the measure of ∠QSR.A20∘B30∘C40∘D50∘ - Question 3 - Select One

In the figure above, ∠SPT=60∘and PQ=PR. Find ∠PQRA50∘B45∘C60∘D55∘
Suppose H(3,2,−1)andC(3,2,5) are respectively the orthocentre and circumcentre of a triangle PQR. If G is centroid of triangle PQR, then OG2=_________ where O is the origin.
View SolutionIn a △PQR,∠P=110∘, PQ = PR . Find ∠Qand∠R
View SolutionIn ΔPQR, S is a point in QR such that angle PQS= angle SPR show that angle PSR= angle QPR
View Solutionयदि ΔABC~ΔPQR तथा ∠A=60∘,तब ∠P=?
View SolutionO is the circumcentre of a triangle ΔABC.The point A and the chord BC are on the opposite side of O.If ∠BOC=150∘.Then the angle ∠BAC is:
View Solution
