Corner points of the feasible region for an LPP are ( 0,2) (3,0) ,(6,0),(6,8) and (0,5) Let Z=4 x + 6y be the objective function. The minimum value of F occurs at?
The correct Answer is:Any point on line segment joining (0,2) and( 3,0)
To find the minimum value of the objective function Z=4x+6y at the given corner points of the feasible region, we will evaluate the function at each corner point. The corner points provided are:
1. (0,2)
2. (3,0)
3. (6,0)
4. (6,8)
5. (0,5)
Step 1: Evaluate Z at each corner point
1. At (0,2):
Z=4(0)+6(2)=0+12=12
2. At (3,0):
Z=4(3)+6(0)=12+0=12
3. At (6,0):
Z=4(6)+6(0)=24+0=24
4. At (6,8):
Z=4(6)+6(8)=24+48=72
5. At (0,5):
Z=4(0)+6(5)=0+30=30
Step 2: Compare the values of Z
Now, we will list the values of Z calculated at each corner point:
- At (0,2), Z=12
- At (3,0), Z=12
- At (6,0), Z=24
- At (6,8), Z=72
- At (0,5), Z=30
Step 3: Identify the minimum value
From the calculated values, the minimum value of Z occurs at the corner points (0,2) and (3,0), both yielding Z=12.
Conclusion
Thus, the minimum value of Z occurs at the corner points (0,2) and (3,0) with a minimum value of 12.
---
2. (3,0)
3. (6,0)
4. (6,8)
5. (0,5)
Z=4(0)+6(2)=0+12=12
Z=4(3)+6(0)=12+0=12
Z=4(6)+6(0)=24+0=24
Z=4(6)+6(8)=24+48=72
Z=4(0)+6(5)=0+30=30
- At (3,0), Z=12
- At (6,0), Z=24
- At (6,8), Z=72
- At (0,5), Z=30
Topper's Solved these Questions
Similar Questions
Corner points of the feasible region for an LPP are (0,2),(3,0),(6,0),(6,8), and (0,5). Let F-4x+6y be the objective function. Determine the minimum valur of F occurs at
View SolutionThe corner points of the feasible region of an LPP are (0,0), (0,8), (2,7),(5,4),and (6,4). the maximum profit P= 3x + 2y occurs at the point_____.
View Solution
Knowledge Check
If the corner points of the feasible region for an L.P.P. are (0, 2), (3, 0), (6, 0), (6, 8) and (0, 5), then the minimum value of the objective function F=4x+6y occurs at
AA) (0, 2) onlyBB) (3, 0) onlyCC) the mid-point of the line segment joining the point (0, 2) and (3, 0) onlyDD) every point on the line segment joining the point (0, 2) and (3, 0)If the corner points of the feasible region for an LPP are (0,2),(3,0),(6,0),(6,8),(0,5) then maximum of F - minimum of F =
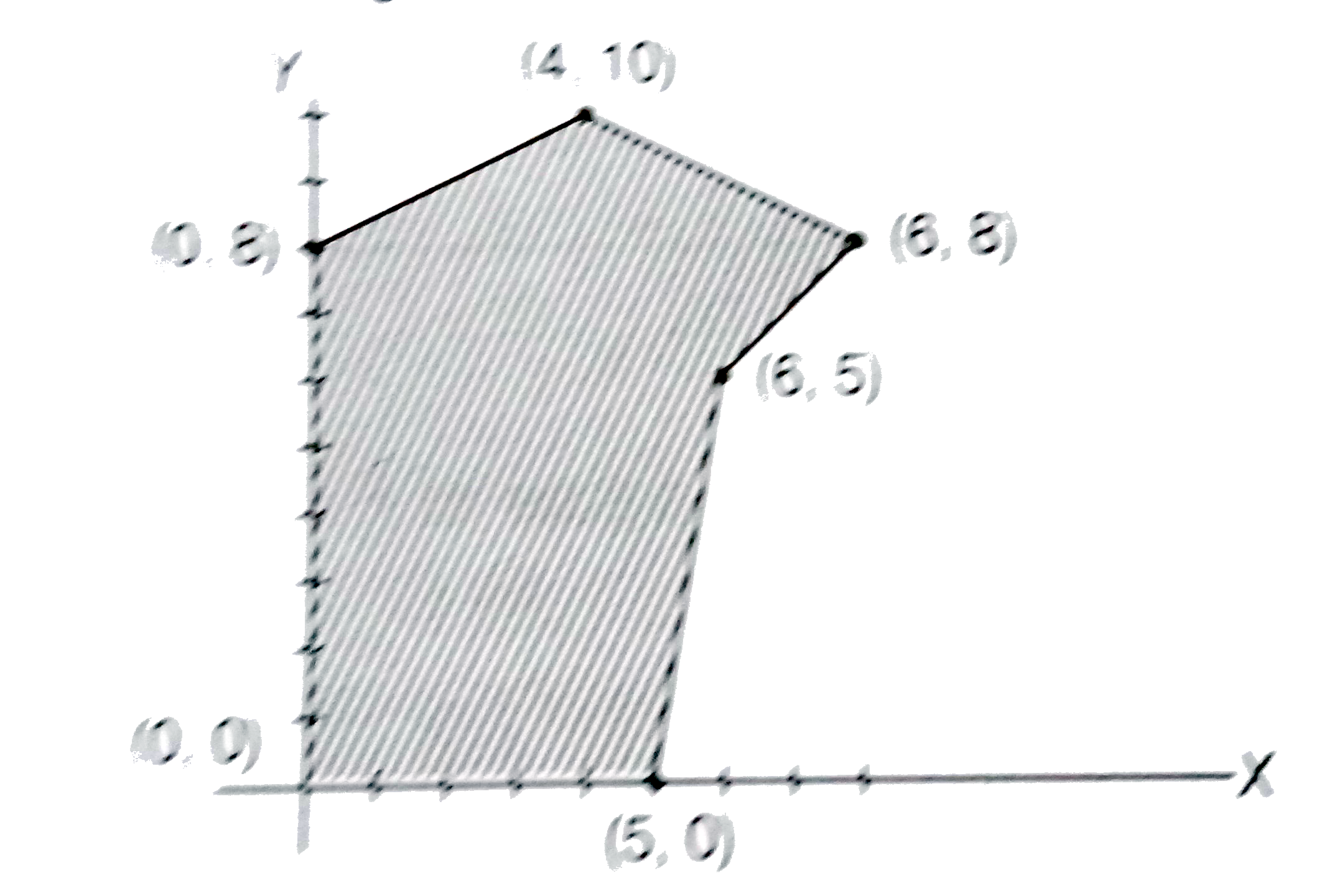
A60B48C42D18If the objective function for an L.P.P. is Z=3x−4y and the corner points for the bounded feasible region are (0, 0), (5, 0), (6, 5), (6, 8), (4, 10) and (0, 8), then the maximum value of Z occurs at
A(0, 0)B(0, 8)C(5, 0)D(4, 10)
The feasible region for an LPP is shown in the following figure. Let F=3x−−4y be the objective function. Maximum value of F is
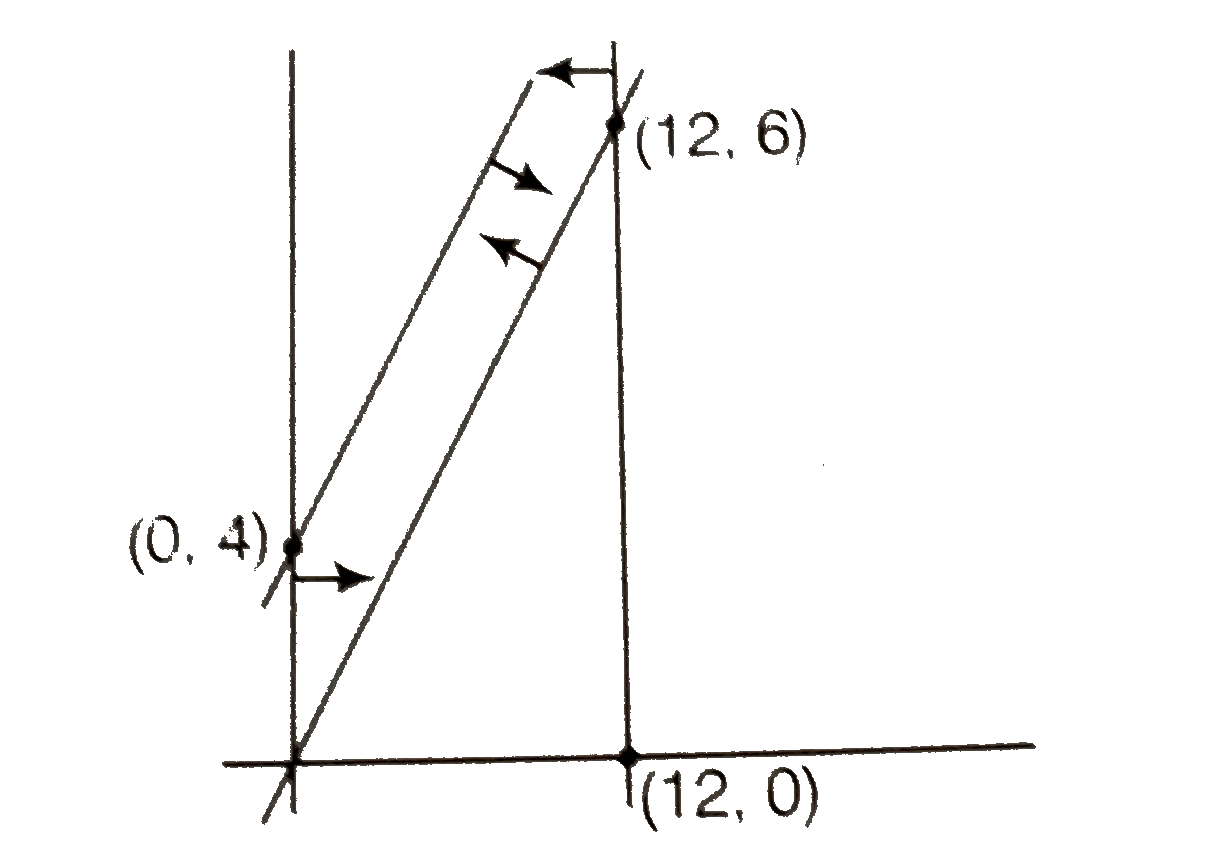
View SolutionThe feasible solution for a LPP is shown in following figure. Let Z=3x-4y be the objective function, Minimum of Z occurs at
View SolutionThe corner points of the feasible region determined by the system of linear contraints are (0,0), (0,40),( 20,40),(60,20),(60,0). The objective function is Z=4x+3y. Compare the quantity in Column A and Column B.
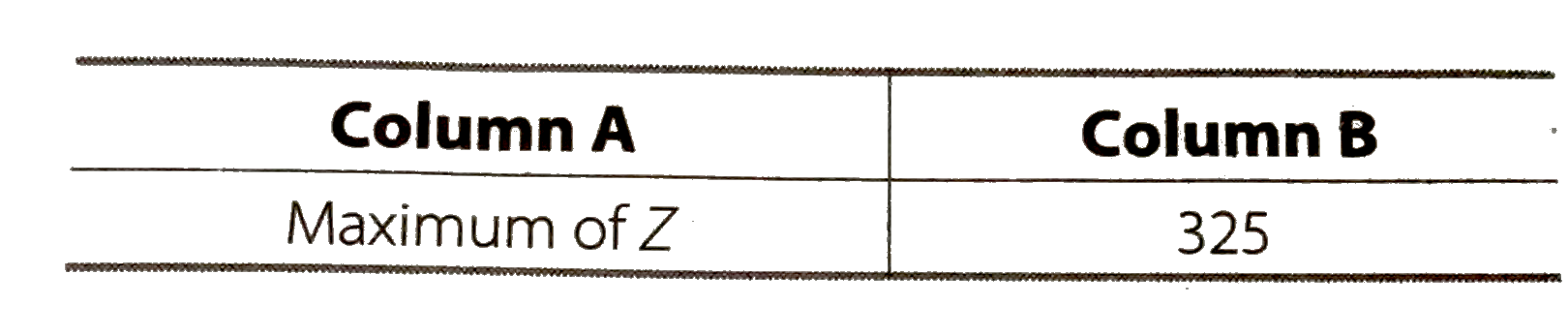
View SolutionIf the objective function for a L.P.P. is Z=5x+7y and the corner points of the bounded feasible region are (0, 0), (7, 0), (3, 4) and (0, 2), then the maximum value of Z occurs at
View SolutionThe occurs point of the feasible region for an L.P.P. are (0, 3), (1, 1) and (3, 0). If objective function is Z=px+qy,p,q>0, then the condition on p and q so that the minimum of Z occurs at (3, 0) and (1, 1) is
View Solution
