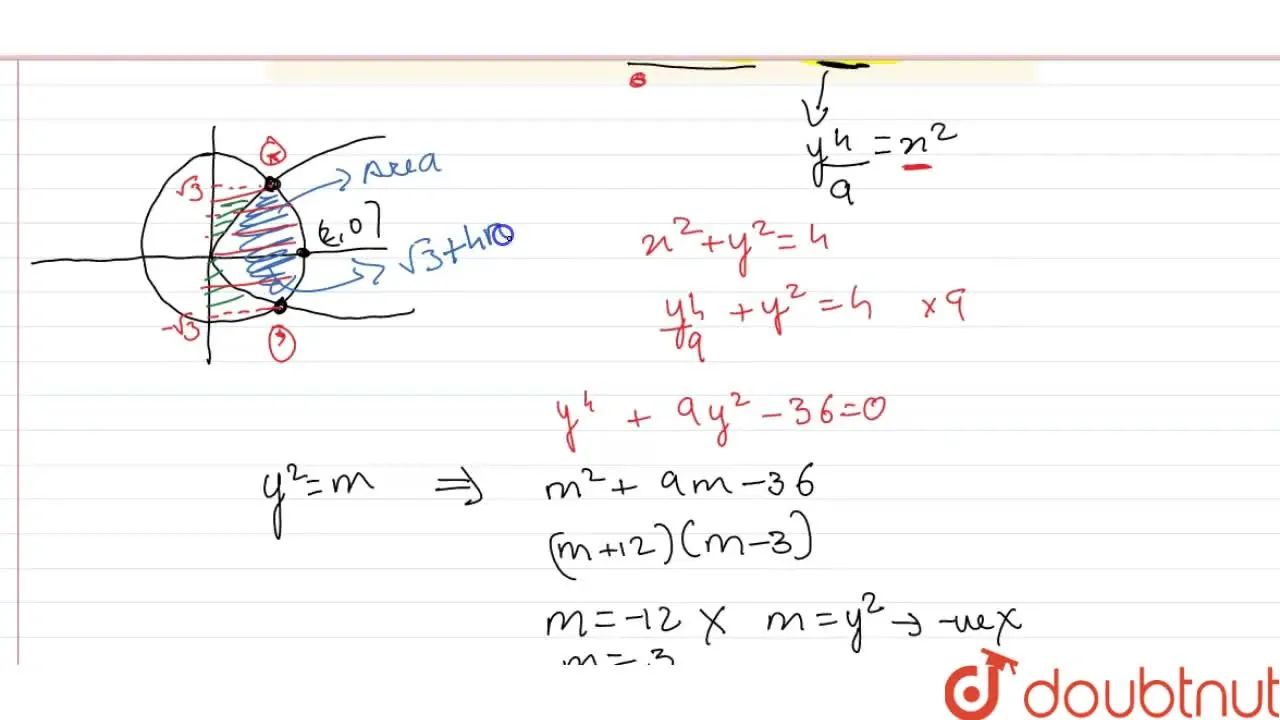
Using method of integration, find the area (in sq. units) of the smaller portion enclosed between the curves, x2+y2=4andy2=3x.
The correct Answer is:(√33+4π3) sq.unit
Step by step video, text & image solution for Using method of integration, find the area (in sq. units) of the smaller portion enclosed between the curves, x^2 + y^2 = 4 and y^2 = 3x. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ICSECHAPTER - MODEL TEST PAPER - 4EXERCISE - SECTION - C10videos
MODEL TEST PAPER - 4
- BOOK - ICSECHAPTER - MODEL TEST PAPER - 4EXERCISE - SECTION - C10videos
MODEL TEST PAPER - 4
- BOOK - ICSECHAPTER - MODEL TEST PAPER - 3 EXERCISE - SECTION - C10videos
MODEL TEST PAPER - 3
- BOOK - ICSECHAPTER - MODEL TEST PAPER - 7EXERCISE - Section - C5videos
MODEL TEST PAPER - 7
Similar Questions
The area enclosed between the curves y2=xandy=|x| is
View SolutionFind the area enclosed between the curves
y2=2x+6 and y=x−1View SolutionUsing the method of integration find the area bounded by the curve |x|+|y|=1.
View SolutionUsing definite integration, find the area of the smaller region bounded by the ellipse 9x2+16y2=144andx=2.
View SolutionUsing integration, find the area of the region enclosed between the two circles x2+y2=4 and (x−2)2+y2=4.
View SolutionFind the area enclosed between the curves
y=4x2 and y=x2+3View SolutionFind the area enclosed by the curves y=4x2 and y2=2x.
View SolutionFind the area enclosed between the curves
y2=2x,y=4x−1View SolutionThe area enclosed between the curves |x|+|y|≥2andy2=4(1−x29) is :
View SolutionShow that the area enclosed between the curves y = x and y=x3 is 12 sq unit.
View Solution