A plane intersects three co-ordinate axes at the points A, B, C. If (1, -2, 3) is the centroid of theΔABC then find the equation of the plane.
The correct Answer is:6x−3y+2z=18
To find the equation of the plane that intersects the coordinate axes at points A, B, and C, given that the centroid of triangle ABC is (1, -2, 3), we can follow these steps:
Step 1: Define the points of intersection
Let the points where the plane intersects the coordinate axes be:
- A = (x, 0, 0) (intersection with the x-axis)
- B = (0, y, 0) (intersection with the y-axis)
- C = (0, 0, z) (intersection with the z-axis)
Step 2: Write the formula for the centroid
The centroid (G) of triangle ABC is given by the formula:
G=(x1+x2+x33,y1+y2+y33,z1+z2+z33)
Substituting the coordinates of points A, B, and C, we have:
G=(x+0+03,0+y+03,0+0+z3)=(x3,y3,z3)
Step 3: Set the centroid equal to the given point
We know that the centroid G is (1, -2, 3). Therefore, we can set up the following equations:
x3=1(1)
y3=−2(2)
z3=3(3)
Step 4: Solve for x, y, and z
From equation (1):
x=3⋅1=3
From equation (2):
y=3⋅(−2)=−6
From equation (3):
z=3⋅3=9
Step 5: Write the intercepts
The intercepts of the plane on the axes are:
- x-intercept (A) = 3
- y-intercept (B) = -6
- z-intercept (C) = 9
Step 6: Write the equation of the plane
The general form of the equation of a plane that intersects the axes at points (a, 0, 0), (0, b, 0), and (0, 0, c) is given by:
xa+yb+zc=1
Substituting the values of a, b, and c:
x3+y−6+z9=1
Step 7: Clear the denominators
To eliminate the fractions, multiply through by the least common multiple (LCM) of the denominators (which is 18):
6x−3y+2z=18
Step 8: Rearranging the equation
Rearranging gives us:
6x−3y+2z−18=0
Final Equation
Thus, the equation of the plane is:
6x−3y+2z=18
Let the points where the plane intersects the coordinate axes be:
- A = (x, 0, 0) (intersection with the x-axis)
- B = (0, y, 0) (intersection with the y-axis)
- C = (0, 0, z) (intersection with the z-axis)
The centroid (G) of triangle ABC is given by the formula:
G=(x1+x2+x33,y1+y2+y33,z1+z2+z33)
Substituting the coordinates of points A, B, and C, we have:
G=(x+0+03,0+y+03,0+0+z3)=(x3,y3,z3)
We know that the centroid G is (1, -2, 3). Therefore, we can set up the following equations:
x3=1(1)
y3=−2(2)
z3=3(3)
From equation (1):
x=3⋅1=3
y=3⋅(−2)=−6
z=3⋅3=9
The intercepts of the plane on the axes are:
- x-intercept (A) = 3
- y-intercept (B) = -6
- z-intercept (C) = 9
The general form of the equation of a plane that intersects the axes at points (a, 0, 0), (0, b, 0), and (0, 0, c) is given by:
xa+yb+zc=1
Substituting the values of a, b, and c:
x3+y−6+z9=1
To eliminate the fractions, multiply through by the least common multiple (LCM) of the denominators (which is 18):
6x−3y+2z=18
Rearranging gives us:
6x−3y+2z−18=0
Thus, the equation of the plane is:
6x−3y+2z=18
Topper's Solved these Questions
- MODEL TEST PAPER - 3 Book:ICSEChapter:MODEL TEST PAPER - 3 Exercise:SECTION - C
Explore 10 Videos
- MODEL TEST PAPER - 3 Book:ICSEChapter:MODEL TEST PAPER - 3 Exercise:SECTION - C
Explore 10 Videos
- MODEL TEST PAPER - 2Book:ICSEChapter:MODEL TEST PAPER - 2Exercise:Section - C
Explore 10 Videos
- MODEL TEST PAPER - 4Book:ICSEChapter:MODEL TEST PAPER - 4Exercise:SECTION - C
Explore 10 Videos
Similar Questions
A plane cuts the co-ordinate axes at A, B and C respectively. If the centroid of ΔABC is (2,−3,4), find the equation of the plane.
View SolutionIf a plane meets the coordinates axes at A, Band C, in such a way that the centroid of ΔABC is at the point (1,2,3), the equation of the plane is
View SolutionA straight line passes through the points P(-1, 4) and Q(5,-2). It intersects the co-ordinate axes at points A and B. M is the midpoint of the segment AB. Find :
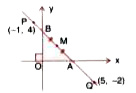
The equation of the line.
View SolutionA plane meets the coordinates axes in A, B, C such that the centroid of the triangle ABC is the point (a,a,a) .Then the equation of the plane is x+y+z=p, where p is ___
View SolutionA plane meets the coordinate axes at A, B, C such that the centroid of ΔABC is the point (a, b, c) . If the equation of the plane is xa+yb+zc=k, then k= 1 b. 2 c. 3 d. none of these
View SolutionA plane meets the coordinate axes at A,BandC respectively such that the centroid of triangle ABC is (1,−2,3). Find the equation of the plane.
View SolutionA plane meets the coordinate axes in A,B,C such that the centroid of triangle ABC is the point (p,q,r). Show that the equation of the plane is xp+yq+zr=3.
View SolutionA plane meets the coordinate axes in A,B,C such that the centroid of triangle ABC is the point (p,q,r). If the equation of the plane is xp+yq+zr=k then k=
View SolutionIf a plane meets the equations axes at A,BandC such that the centroid of the triangle is (1,2,4), then find the equation of the plane.
View SolutionIf a plane meets the equations axes at A,BandC such that the centroid of the triangle is (1,2,4), then find the equation of the plane.
View Solution

