Two sample sizes of 50 and 100 are given . The mean of these samples respectively are 56 and 50. Find the mean of the size 150 by combining the two samples.
The correct Answer is:52
To find the combined mean of two samples with given sizes and means, we can follow these steps:
Step 1: Identify the given data
- Sample A:
- Size (n₁) = 50
- Mean (Ā) = 56
- Sample B:
- Size (n₂) = 100
- Mean (B̄) = 50
Step 2: Calculate the total sums for each sample
The total sum for each sample can be calculated using the formula:
Total Sum=Mean×Size
For Sample A:
Total Sum of A=Aˉ×n₁=56×50=2800
For Sample B:
Total Sum of B=Bˉ×n₂=50×100=5000
Step 3: Calculate the combined total sum
Now, we can find the combined total sum of both samples:
Combined Total Sum=Total Sum of A+Total Sum of B
Combined Total Sum=2800+5000=7800
Step 4: Calculate the combined size
The combined size of the two samples is:
Combined Size=n₁+n₂=50+100=150
Step 5: Calculate the combined mean
The combined mean (C̄) can be calculated using the formula:
Cˉ=Combined Total SumCombined Size
Cˉ=7800150=52
Final Answer
The mean of the combined sample of size 150 is 52.
---
- Sample A:
- Size (n₁) = 50
- Mean (Ā) = 56
- Sample B:
- Size (n₂) = 100
- Mean (B̄) = 50
The total sum for each sample can be calculated using the formula:
Total Sum=Mean×Size
Total Sum of A=Aˉ×n₁=56×50=2800
Total Sum of B=Bˉ×n₂=50×100=5000
Now, we can find the combined total sum of both samples:
Combined Total Sum=Total Sum of A+Total Sum of B
Combined Total Sum=2800+5000=7800
The combined size of the two samples is:
Combined Size=n₁+n₂=50+100=150
The combined mean (C̄) can be calculated using the formula:
Cˉ=Combined Total SumCombined Size
Cˉ=7800150=52
The mean of the combined sample of size 150 is 52.
Topper's Solved these Questions
Similar Questions
Two samples of sizes 50 and 100 are given. The means of these samples respectively are 56 and 50 find the mean of size 150 by combining the two samples.
View SolutionOne set of 100 observations has the mean 15 and another set of 150 observation has the mean 16. Find the mean of 250 observation by combining the two sets of given observations.
View Solution
Knowledge Check
Two sample of sizes 50 and 100 given. The mean of these samples respectively are 56 and 50. Then the mean of size 150 combining is
A55B52C50DNone of these
The mean of two samples is 45. The mean of 1st samples and 2nd samples ware 30 and 50 respectively. Determine the ratio of the number of observations of the two samples.
View SolutionTwo samples of DNA, A and B have melting points 340K and 350 K respectively. This is because
View SolutionThe mean of two samples of sizes 200 and 300 were found to be 25, 10 respectively. Their standard deviations were 3 and 4 respectively. The variance of combined sample of size 500 is
View SolutionThe daily income of a sample of 50 employees are tabulated as follows.
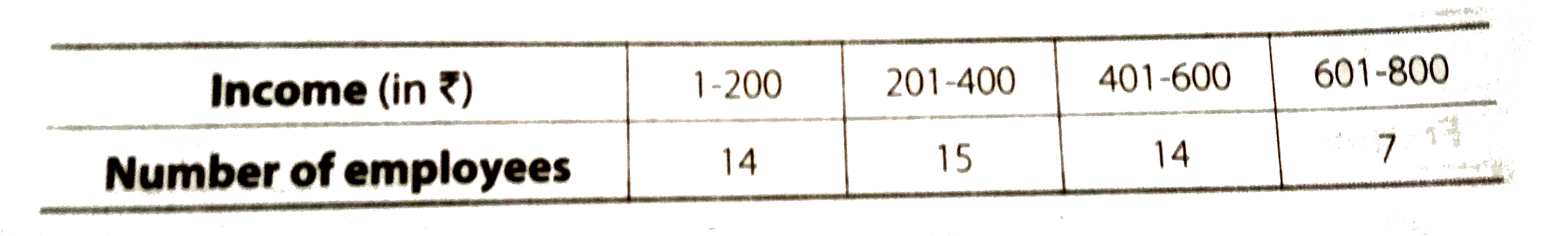
Find the mean daily income of employees.
View SolutionA dice is thrown two times.describe sample space
View SolutionFor two data sets each of size 5 the variances are given by 4 and 5 and the corresponding means are given to be 2 and 4 respectively. Then find the variance of the combined data.
View SolutionIn a mean life of a radioactive sample
View Solution
