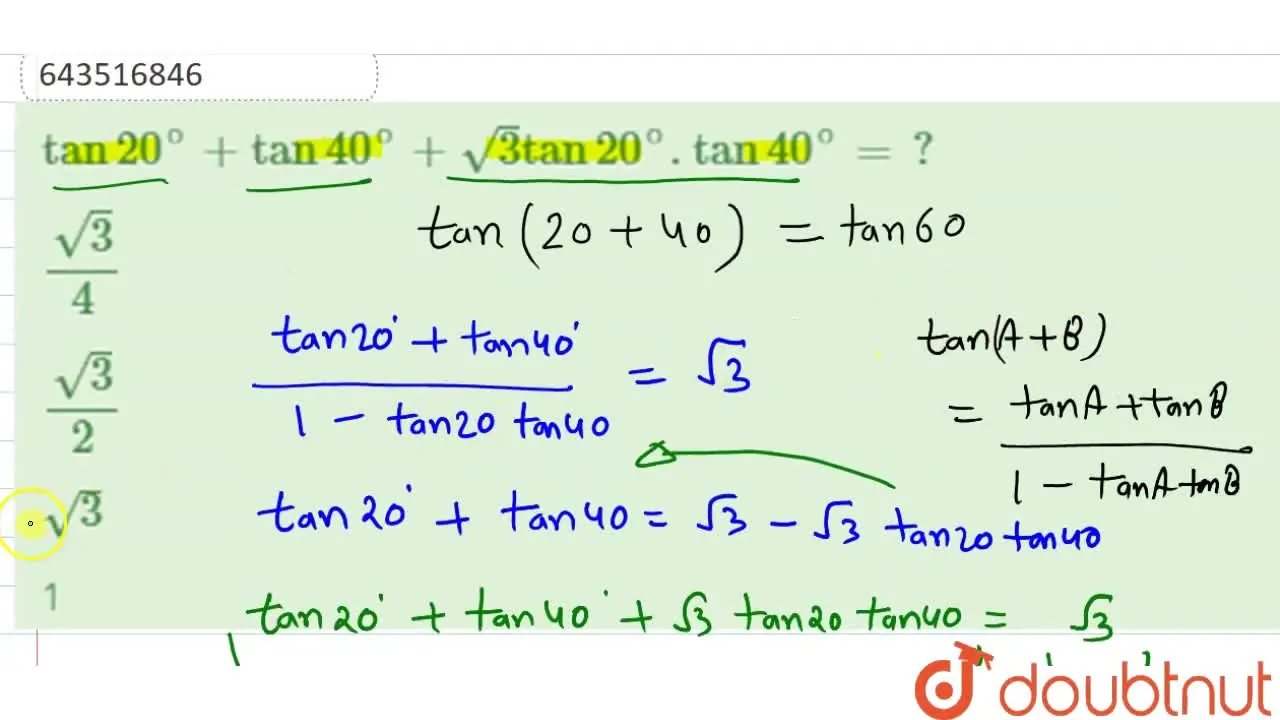
tan20∘+tan40∘+√3tan20∘.tan40∘=?
A
√34
B
√32
C
√3
D
1
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video, text & image solution for tan20^(@)+tan40^(@)+sqrt(3)tan20^(@).tan40^(@)=? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023
Related Playlists
Similar Questions
tan20∘−tan80∘+√3tan20∘tan80∘=
View Solutiontan20∘+tan40∘+√3.tan20∘.tan40∘=
View Solutiontan20∘−tan80∘+√3=
View Solutiontan20∘+2tan50∘=
View Solutiontan40∘+tan80∘−√3tan40∘tan80∘=
View Solutiontan200tan400tan600tan800
View Solutiontan20∘+tan40∘+√3+√3tan20∘tan40∘=
View SolutionThe value of tan40∘+tan20∘+√3tan20∘tan40∘ is
View Solutiontan20∘tan40∘tan80∘
View SolutionWithout using trigonometric tables , prove that :
(i) tan20∘tan40∘tan45∘tan50∘tan70∘=1
(ii) tan1∘tan2∘tan60∘tan88∘tan89∘=√3
(iii) cot5∘cot10∘cot30∘cot80∘cot85∘=√3
(iv) 4sin10∘sin20∘sin30∘sec70∘sec80∘=2View Solution