If Tn=sinnθ+cosnθ then T3−T5T1=?
sinθ.cosθ
sin2θ.cos2θ
sin2θ.cosθ
sinθ.cos2θ
The correct Answer is:B
To solve the problem, we need to evaluate the expression (T3−T5)/T1 where Tn=sinnθ+cosnθ.
1. Define Tn:
Tn=sinnθ+cosnθ
2. Calculate T3:
T3=sin3θ+cos3θ
3. Calculate T5:
T5=sin5θ+cos5θ
4. Calculate T1:
T1=sinθ+cosθ
5. Substitute into the expression:
T3−T5T1=(sin3θ+cos3θ)−(sin5θ+cos5θ)sinθ+cosθ
6. Factor the numerator:
- For T3−T5, we can factor out sin3θ and cos3θ:
T3−T5=sin3θ(1−sin2θ)+cos3θ(1−cos2θ)
- This simplifies to:
T3−T5=sin3θcos2θ+cos3θsin2θ
7. Rewrite the numerator:
T3−T5=sin2θcos2θ(sinθ+cosθ)
8. Substitute back into the expression:
T3−T5T1=sin2θcos2θ(sinθ+cosθ)sinθ+cosθ
9. Cancel out (sinθ+cosθ):
T3−T5T1=sin2θcos2θ
Final Result:
T3−T5T1=sin2θcos2θ
Tn=sinnθ+cosnθ
T3=sin3θ+cos3θ
T5=sin5θ+cos5θ
T1=sinθ+cosθ
T3−T5T1=(sin3θ+cos3θ)−(sin5θ+cos5θ)sinθ+cosθ
- For T3−T5, we can factor out sin3θ and cos3θ:
T3−T5=sin3θ(1−sin2θ)+cos3θ(1−cos2θ)
- This simplifies to:
T3−T5=sin3θcos2θ+cos3θsin2θ
T3−T5=sin2θcos2θ(sinθ+cosθ)
T3−T5T1=sin2θcos2θ(sinθ+cosθ)sinθ+cosθ
T3−T5T1=sin2θcos2θ
T3−T5T1=sin2θcos2θ
Topper's Solved these Questions
Similar Questions
Knowledge Check
If Tn=cosnθ+sinnθ,then2T6−3T4+1=
A2B3C0D1Suppose we consider friction between string and the pulley while still considering the string to be massless. So, in such a theoretical case, the tension in the string in contant with the pulley will not be constant and its variation is calculated as follows.
If the string is just on the verge of slipping on the pulley, then friction acting is limiting and if T2>T1 then friction will act towards T1 in tangential direction as shown.
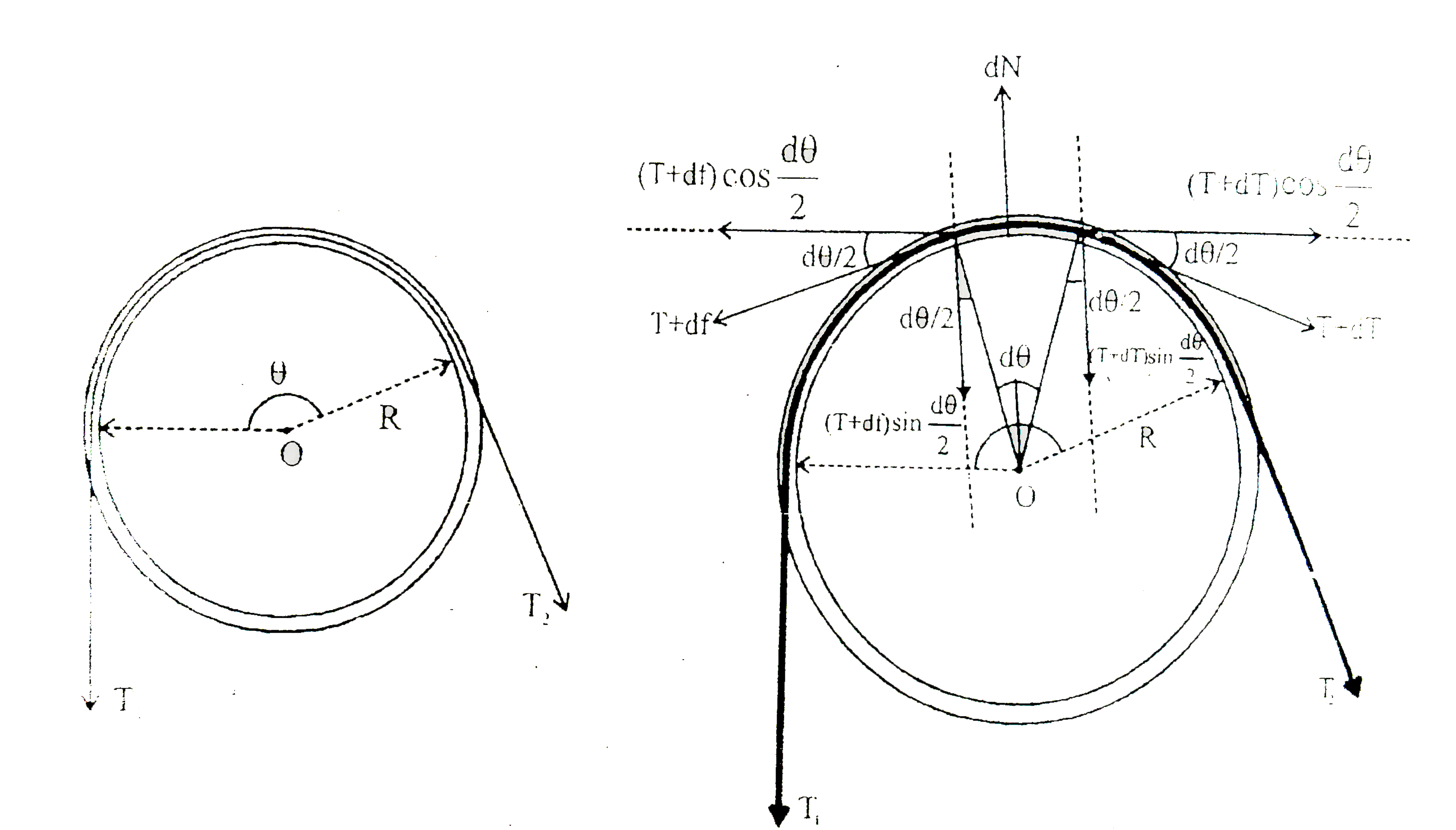
dN=(T+df)sin(dθ/2)+(T+dT)sin(dθ/2)
dN=Tsin(dθ/2)+dfsin(d∂/2)+Tsin(dθ/2)+dTsin(dθ/2)
dN≈2Tsin(dθ/2)
dN≈2Tdθ2
dN=Tdθ ........(1)
Alsso, (T+df)cos(dθ2)=(T+dT)cos(dθ2)
Tcos(dθ2)+dfcos(dθ2)=Tcos(dθ2)1dTcos(dθ2)
df=dT=μdN.........(2)
from (1) & (2)
dT=μTdθ
∫T2T1dTT=μ∫θ0dθ
ln(T2T1)=μθ
T2=T1eμθ, where θ is the angle of contact between the string and the pulley Based on above information, answer the following questions.
Two masses m1kg and m2kg passes over an atwood machine. Find the ratio of masses m1 and m2 so that string passing over the pulley will just start slipping over its surface. The friction coefficient between the string and pulley surface is 0.2.Ae0.2πBe0.2C0.2De0.4π
Prove the trigonometric identities: If Tn=sinnθ+cosnθ, prove that T3−T5T1=T5−T7T3
View SolutionIf Tn=sinnx+cosnx , prove that (i) 2T6−3T4+10=0
View SolutionIf Tn=sinnx+cosπx prove that T3−T5T1=T5−T7T3
View SolutionIf Tn=sinnθ+cosnθ then prove that (T5−T3):(T7−T5)=T1:T3.
View SolutionIf Tn=sinnθ+cosnθ, then T6−T4T6=m holds for values of m satisfying (A) m∈[−1,13] (B) m∈[0,13] (C) m∈[−1,0] (D) m∈[−1,−12]
View SolutionIf Tn=sinnx+cosnx, prove that 2T6−3T4+1=0
View Solution
