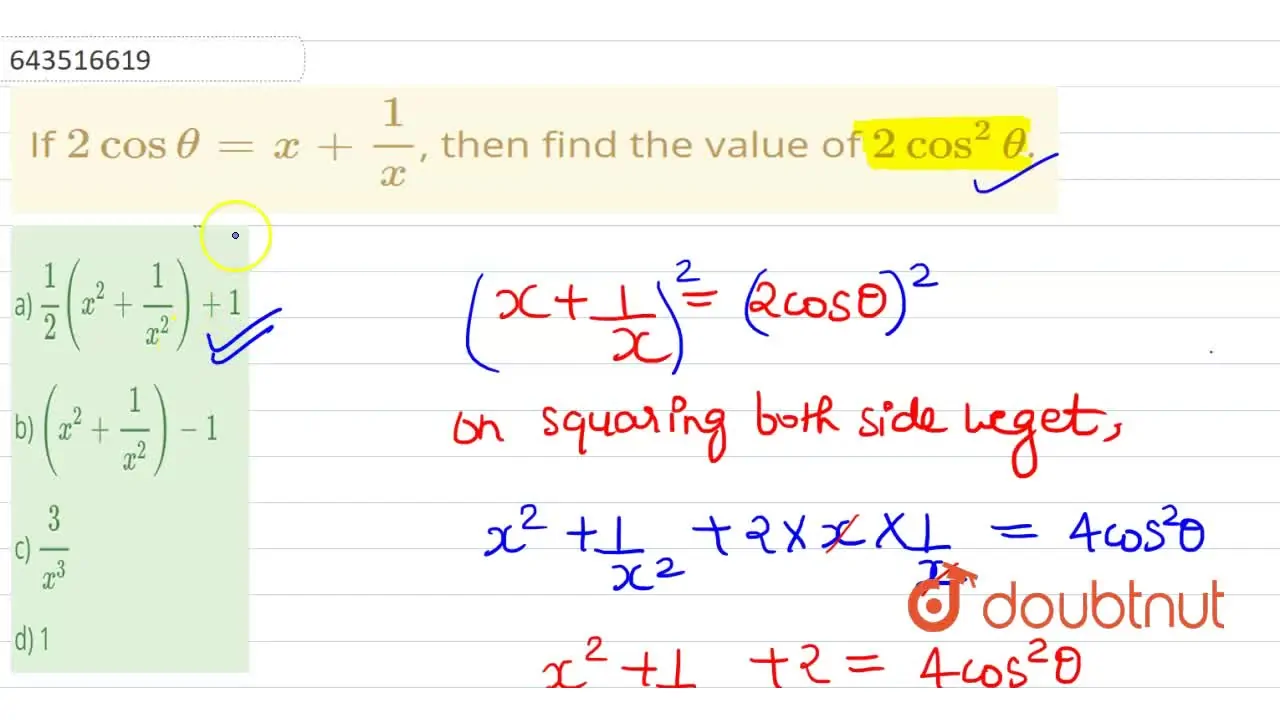
If 2cosθ=x+1x, then find the value of 2cos2θ.
A
a) 12(x2+1x2)+1
B
b) (x2+1x2)−1
C
c) 3x3
D
d) 1
Video Solution
Text Solution
The correct Answer is:A
|
Answer
Step by step video, text & image solution for If 2costheta=x+(1)/(x), then find the value of 2cos^(2)theta. by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023
Related Playlists
Similar Questions
If cosθ=1√2,then find the value of cos2θ.
View SolutionIf 2cosθ=x+1x, find the value of 2cos3θ
View SolutionIf cosθ=4x1+4x2 then find the value of sin θ
View SolutionIf cosθ=1√2,then find the value of (tan2θ+1).
View SolutionIf sinθ=x2−y2x2+y2 then find the values of cosθ and cotθ
View SolutionIf secθ=32,then find value of 2cosθ−tan2θsin2θ+1.
View SolutionIf cos θ=12(x+1x), what is the value of cos 2θ ?
View SolutionIf cosθ+cos2θ=1, then find the value of sin4θ+sin2θ.
View Solutionsinθ=1√2,then find the value of 3sin2θ−4sin3θ⋅cosθ.
View SolutionIf θ is an acute angle and sinθ=cosθ, then find the value of 2tan2θ+sin2θ−1.
View Solution