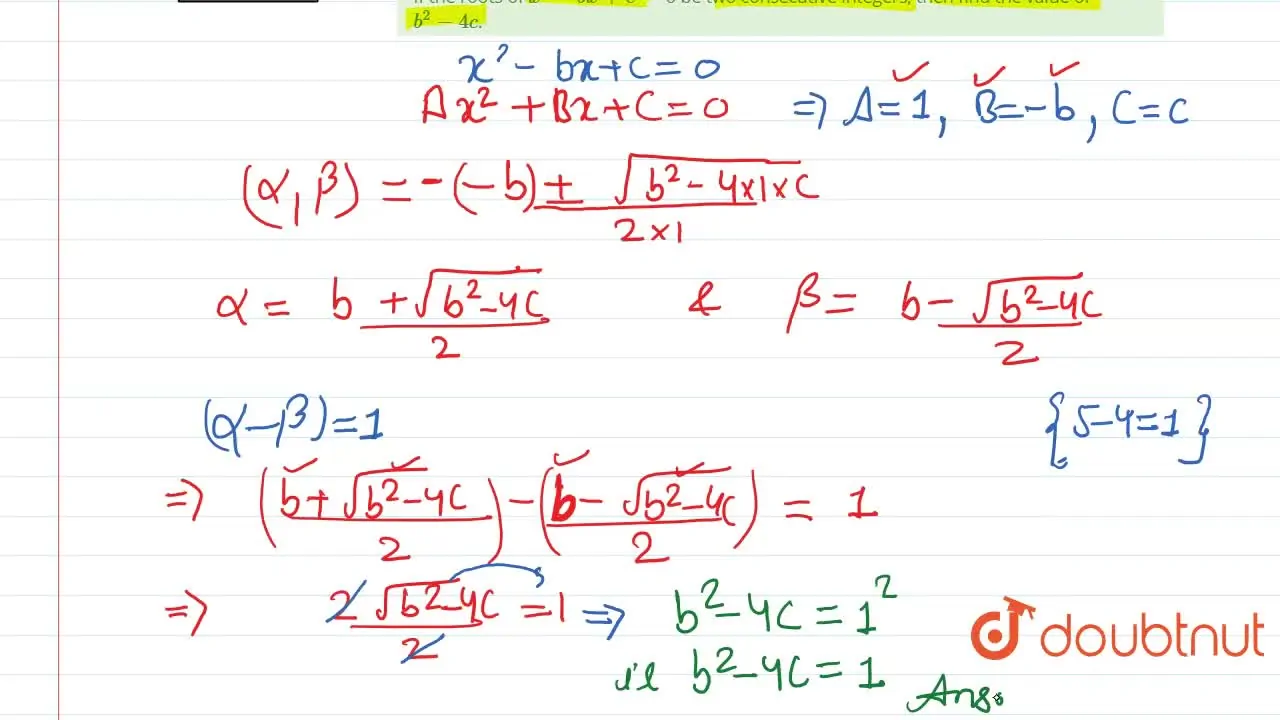
If the roots of x2−bx+c=0 be two consecutive integers, then find the value of b2−4c.
The correct Answer is:1
Step by step video, text & image solution for If the roots of x^(2)-bx+c=0 be two consecutive integers, then find the value of b^(2)-4c. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - ICSECHAPTER - QUADRATIC EQUATIONSEXERCISE - EXERCISE 10 (d)14videos
QUADRATIC EQUATIONS
- BOOK - ICSECHAPTER - QUADRATIC EQUATIONSEXERCISE - EXERCISE 10 (e)4videos
QUADRATIC EQUATIONS
- BOOK - ICSECHAPTER - QUADRATIC EQUATIONSEXERCISE - EXERCISE 10 (b)16videos
QUADRATIC EQUATIONS
- BOOK - ICSECHAPTER - PROPERTIES OF TRIANGLEEXERCISE - EXERCISE 738videos
PROPERTIES OF TRIANGLE
- BOOK - ICSECHAPTER - RELATION AND FUNCTIONSEXERCISE - MULTIPLE CHOICE QUESTIONS (Choose the correct answer from the given four options in questions)32videos
RELATION AND FUNCTIONS
Similar Questions
If the roots of x2−bx+c=0 are two consecutive integers then b2−4c=
View SolutionIf the roots of the equation x2−bx+c=0 are two consecutive integers, then find the value of b2−4c
View SolutionIf the roots of x2−bx+c=0 are two consecutive integers, then b2−4c is (a)0 (b) 1 (c) 2 (d) none of these
View SolutionIf the roots of the cubic x3+ax2+bx+c=0 are three consecutive positive integers, then the value of a2b+1=
View SolutionIf the roots of the cubic, x3+ax2+bx+c=0 are three consecutive positive integers, then the value of a2b+1 is equal to __________.
View SolutionIf the roots of the cubic equation, x3+ax2+bx+c=0 are three consecutive positive integers, then the value of (a2/b+1) is equal to?
View SolutionIf sinθandcosθ are the roots of the quadratic equation ax2+bx+c=0(a≠0). Then find the value of b2−a2ac.
View SolutionLet f(x)=x3+ax2+bx+c and g(x)=x3+bx2+cx+a, where a, b, c are integers. Suppose that the following conditions hold-
(a) f(1)=0,
(b) the roots of g(x) = 0 are the squares of the roots of f(x) = 0. Find the value of: a2013+b2013+c2013View SolutionIf a, b and c are consecutive integers, what is a+b+c?
View SolutionAll the roots of x3+ax2+bx+c are positive integers greater than 2, and the coefficients satisfy a+b+c+1=−385. Find the value of √a+51
View Solution