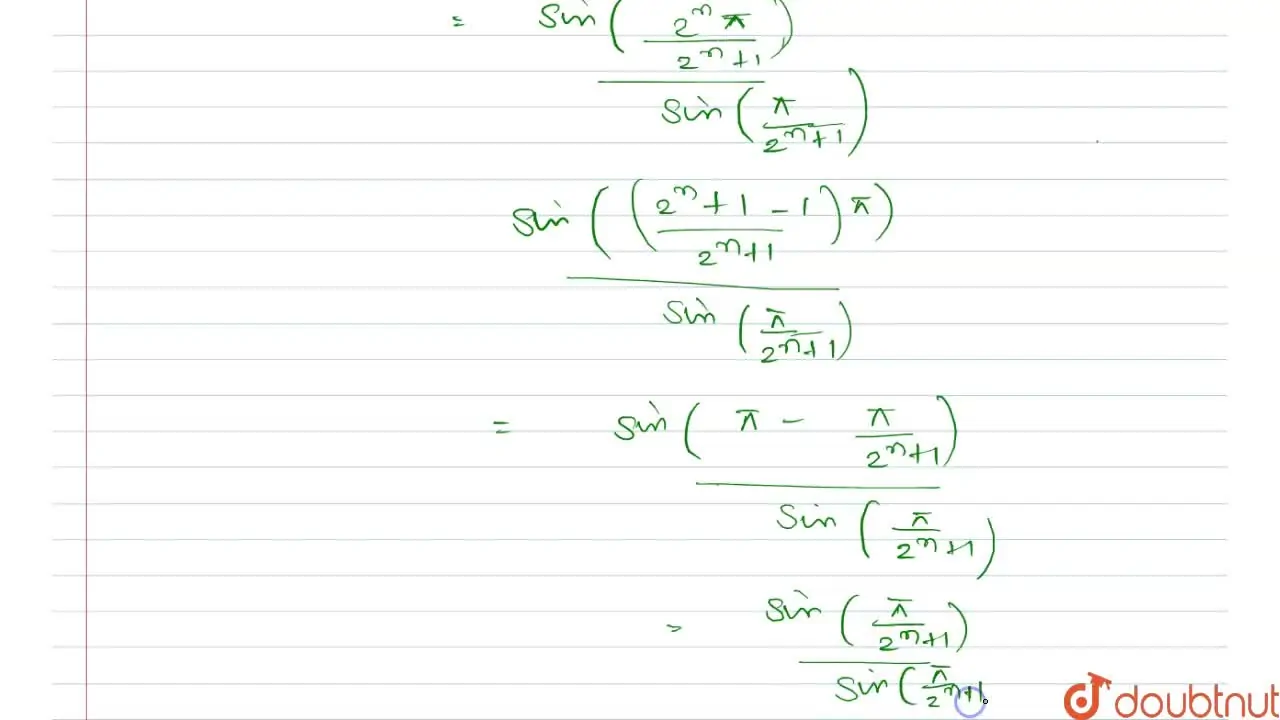If θ=π2n+1 prove that 2ncosθcos2θcos22θ….Cos2n−1θ=1
Step by step video & image solution for If theta = (pi)/(2 ^(n) +1) prove that 2 ^(n) cos theta cos 2 theta cos 2 ^(2) theta…. Cos 2 ^(n -1) theta =1 by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - ICSECHAPTER - COMPOUND AND MULTIPLE ANGLES EXERCISE - CHEPTER TEST23videos
COMPOUND AND MULTIPLE ANGLES
- BOOK - ICSECHAPTER - COMPOUND AND MULTIPLE ANGLES EXERCISE - EXERCISE 5 (C )30videos
COMPOUND AND MULTIPLE ANGLES
- BOOK - ICSECHAPTER - COMPLEX NUMBERSEXERCISE - Chapter Test25videos
COMPLEX NUMBERS
- BOOK - ICSECHAPTER - CONIC SECTIONS EXERCISE - Multiple Choice Questions33videos
CONIC SECTIONS
Similar Questions
If θ=π2n+1 , prove that: 2ncosθcos2θcos22cos2n−1θ=1.
View SolutionIf (2n+1)θ=π then 2ncosθcos2θcos22θ.......cos2n−1θ=
View SolutionFor all n∈N,cosθcos2θcos4θ....cos2n−1θ equals to
View SolutionProve that 1−cos2θsin2θ=tanθ.
View SolutionFind the value of cosθ.cos2θ.cos22θ.cos23θ…….cos2n−1θ is
View SolutionProve that : 2cos2nθ+12cosθ+1=(2cosθ−1)(2cos2θ−1)(2cos22θ−1)…(2cos2n−1θ−1)
View SolutionProve that : sin2θcosθ+cosθ=secθ
View SolutionProve that cos6θ+sin6θ=1−3sin2θcos2θ
View SolutionProve: tan2θcos2θ=1−cos2θ
View SolutionIf θ=π7, show that cosθcos2θcos3θ=18.
View Solution