If a triangle is inscribed in a regular decagon such that all the three vertices of the triangle are the vertices of the decagon, but none of the triangle's sides is the side of the decagon.How many such triangles can be formed?
40
50
60
70
The correct Answer is:B
Step by step video, text & image solution for If a triangle is inscribed in a regular decagon such that all the three vertices of the triangle are the vertices of the decagon, but none of the triangle's sides is the side of the decagon.How many such triangles can be formed? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The number of triangles whose vertices are at the vertices of a octagon but none of the sides of such triangles are taken from the sides of the octagon.
A8B15C16Dnone of these - Question 2 - Select One
The number of triangles whose vertices are at the vertices of a octagon but none of the sides of such triangles are taken from the sides of the octagon.
A8B15C16Dnone of these - Question 3 - Select One
The ratio of area of triangle inscribed in a parabola to the area of the triangle formed by the tangents at the vertices of the triangle is
A1B2C1/2Dnone
Triangles are formed by joining vertices of a octagon then numbr of triangle
View SolutionName the vertices and sides of the given triangle.
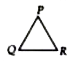 View Solution
View SolutionName the sides, vertices and angles of the given triangle.
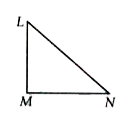 View Solution
View SolutionThe number of triangles whose vertices are at the vertices of an octagon but none of whose sides happen to come from the sides of the octagon is:
View SolutionThe number of triangles whose vertices are at the vertices of an octagon but none of whose sides happen to come from the sides of the octagon is:
View Solution
