Solve the following problems independently of each other
Find the value of m, if there are maximum 28 triangles formed by joining total 9 points on a plane, out of which m points are collinear
3
4
1
8
The correct Answer is:D
To solve the problem of finding the value of m such that there are a maximum of 28 triangles formed by joining a total of 9 points on a plane, out of which m points are collinear, we can follow these steps:
Step 1: Understand the Problem
We have 9 points in total, and m of these points are collinear. The maximum number of triangles that can be formed by any 3 points from these 9 points is given by the combination formula (nr), where n is the total number of points and r is the number of points to choose (in this case, 3).
Step 2: Calculate Total Triangles Without Collinearity
The total number of triangles that can be formed from 9 points is:
(93)=9!3!(9−3)!=9×8×73×2×1=84
Step 3: Calculate the Effect of Collinear Points
When m points are collinear, they cannot form a triangle. The number of triangles that can be formed from the m collinear points is:
(m3)
Thus, the maximum number of triangles that can be formed with the remaining points is:
Maximum triangles=(93)−(m3)
Step 4: Set Up the Equation
According to the problem, the maximum number of triangles formed is 28. Therefore, we can set up the equation:
84−(m3)=28
Step 5: Solve for (m3)
Rearranging the equation gives:
(m3)=84−28=56
Step 6: Find Possible Values of m
Now, we need to find m such that:
(m3)=56
This can be expressed as:
m(m−1)(m−2)6=56
Multiplying both sides by 6 gives:
m(m−1)(m−2)=336
Step 7: Test Possible Values of m
We can test integer values for m:
- For m=7:
7×6×5=210(not 336)
- For m=8:
8×7×6=336(correct!)
- For m=9:
9×8×7=504(too high)
Conclusion
The only value of m that satisfies the equation is m=8.
Final Answer
Thus, the value of m is 8.
We have 9 points in total, and m of these points are collinear. The maximum number of triangles that can be formed by any 3 points from these 9 points is given by the combination formula (nr), where n is the total number of points and r is the number of points to choose (in this case, 3).
The total number of triangles that can be formed from 9 points is:
(93)=9!3!(9−3)!=9×8×73×2×1=84
When m points are collinear, they cannot form a triangle. The number of triangles that can be formed from the m collinear points is:
(m3)
Thus, the maximum number of triangles that can be formed with the remaining points is:
Maximum triangles=(93)−(m3)
According to the problem, the maximum number of triangles formed is 28. Therefore, we can set up the equation:
84−(m3)=28
Rearranging the equation gives:
(m3)=84−28=56
Now, we need to find m such that:
(m3)=56
This can be expressed as:
m(m−1)(m−2)6=56
Multiplying both sides by 6 gives:
m(m−1)(m−2)=336
We can test integer values for m:
7×6×5=210(not 336)
- For m=8:
8×7×6=336(correct!)
9×8×7=504(too high)
The only value of m that satisfies the equation is m=8.
Thus, the value of m is 8.
Topper's Solved these Questions
Similar Questions
Solve the following problems independently of each other.
Find the total number of the shortest routes from one corner to its opposite corner in the following diagram.
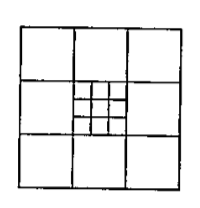
View SolutionSolve the following problems independently of each other.
Find the total number of the shortest routes from one corner to its opposite corner in the following diagram.
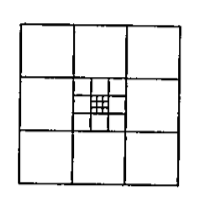
View Solution
Knowledge Check
Solve the following problems independently of each other
Find the number of diagonals in a heptagonA14B21C12D16Find the number of diagonals in an n-sided polygon.
A^nC2−nB^nC2C^nC2+nDn(n−3)2A polygon has 54 diagonals . Find the number of its sides.
A18B12C14D9
Solve the following problems independently of each other.
Find the total number of the shortest routes from one corner to its opposite corner in the given diagram.

View SolutionSolve the following problems independently of each other.
Find the total number of triangles in a pentagon when all its vertices are joined with each other
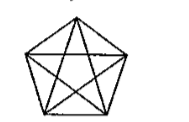
View SolutionFind the number of straight lines formed by joining 6 different points on a plane, no three of them being collinear.
View SolutionFind the number of different straight lines obtained by joining n points on a plane, no three of which are collinear.
View SolutionFind the number if triangle that can be formed by joining the 6 non - collinear points on a plane .
View Solution
