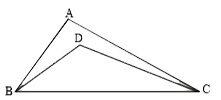
In a triangle ABC, the median BD yields ∠BDC=45∘ and ∠ABD=∠BCD=x∘. Find the value of x.
45∘
30∘
60∘
none of these
The correct Answer is:A
To solve the problem, we need to find the value of x in triangle ABC given the conditions about the angles.
1. Identify the Angles:
- We are given that ∠BDC=45∘.
- We also know that ∠ABD=∠BCD=x∘.
2. Use the Triangle Angle Sum Property:
- In triangle ABD, the sum of the angles is 180∘.
- Therefore, we can write the equation for the angles in triangle ABD:
∠ABD+∠ADB+∠DAB=180∘
- Substituting the known angles, we have:
x+∠ADB+∠DAB=180∘
3. Express ∠ADB and ∠DAB:
- Since BD is a median, D divides AC into two equal parts.
- This means that ∠ADB and ∠DAB can be expressed in terms of x and 45∘.
- Specifically, ∠ADB=45∘ and ∠DAB=x.
4. Set Up the Equation:
- Now substituting back into the angle sum equation:
x+45∘+x=180∘
- Simplifying this gives:
2x+45∘=180∘
5. Solve for x:
- Rearranging the equation:
2x=180∘−45∘
2x=135∘
- Dividing both sides by 2:
x=135∘2=67.5∘
Conclusion:
The value of x is 67.5∘.
- We are given that ∠BDC=45∘.
- We also know that ∠ABD=∠BCD=x∘.
- In triangle ABD, the sum of the angles is 180∘.
- Therefore, we can write the equation for the angles in triangle ABD:
∠ABD+∠ADB+∠DAB=180∘
- Substituting the known angles, we have:
x+∠ADB+∠DAB=180∘
- Since BD is a median, D divides AC into two equal parts.
- This means that ∠ADB and ∠DAB can be expressed in terms of x and 45∘.
- Specifically, ∠ADB=45∘ and ∠DAB=x.
- Now substituting back into the angle sum equation:
x+45∘+x=180∘
- Simplifying this gives:
2x+45∘=180∘
- Rearranging the equation:
2x=180∘−45∘
2x=135∘
- Dividing both sides by 2:
x=135∘2=67.5∘
The value of x is 67.5∘.
Topper's Solved these Questions
Similar Questions
Knowledge Check
In a triagle Δ ABC, the angle bisectors of ∠ABC and ∠ACB meet at point D and angle ∠BDC is 135∘ . Find the value of ∠BAC.
A45∘B75∘C60∘D90∘In a ΔABC,∠A+∠B=118∘
∠A+∠C=96∘ . Find the value of ∠AA36∘B40∘C30∘D34∘If O is the incentre of a triangle ABC and ∠AOC=124∘, find the ∠ABC.
A56B112C68Dcan't be determined
In △ABC,BD⊥AC, at D, E is a point on BC such that ∠BEA=x∘,If ∠EAC=46∘ and ∠EBD=60∘, then the value of x is:
त्रिभुज ABC में, BD⊥AC, E, BC पर एक बिंदु इस प्रकार है की ∠BEA=x∘ , यदि ∠EAC=46∘ और ∠EBD=60∘, तो x का मान ज्ञात करे |
View SolutionIn the adjoining figure ∠BAD=a,∠ABC=band∠BCD=c and ∠ADC=d, find the value of ∠ABC in terms of a, c and d:
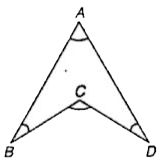
View SolutionIn △ABC,BD⊥AC, at D,E is a point on BC such that ∠BEA=x∘. If ∠EAC=62∘ and ∠EBD=60∘, then the value of x is :
त्रिभुज ABC में, BD⊥AC पर D, E, BC पर एक बिंदु है जेसे कि ∠BEA=x∘ यदि ∠EAC=62∘ और ∠EBD=60∘ है, तो x का मान है :
View SolutionIn △ABC ,BD is perpendicular to AC. E is a point on BC such that ∠BEA=x∘. If ∠EAC=38∘ and ∠EBD=40∘, then the value of x is:
त्रिभुज ABC में,BD, AC पर लम्ब है | E, BC पर स्थित ऐसा बिंदु है कि ∠BEA=x∘ है | यदि ∠EAC=38∘ और ∠EBD=40∘ है, तो x का मान क्या होगा ?
View SolutionIn a triangle aBC ,∠B=2∠C.AD and BE bisectors of ∠BACand∠ABC. If AB=CD . Find ∠BAC
View Solution