Question

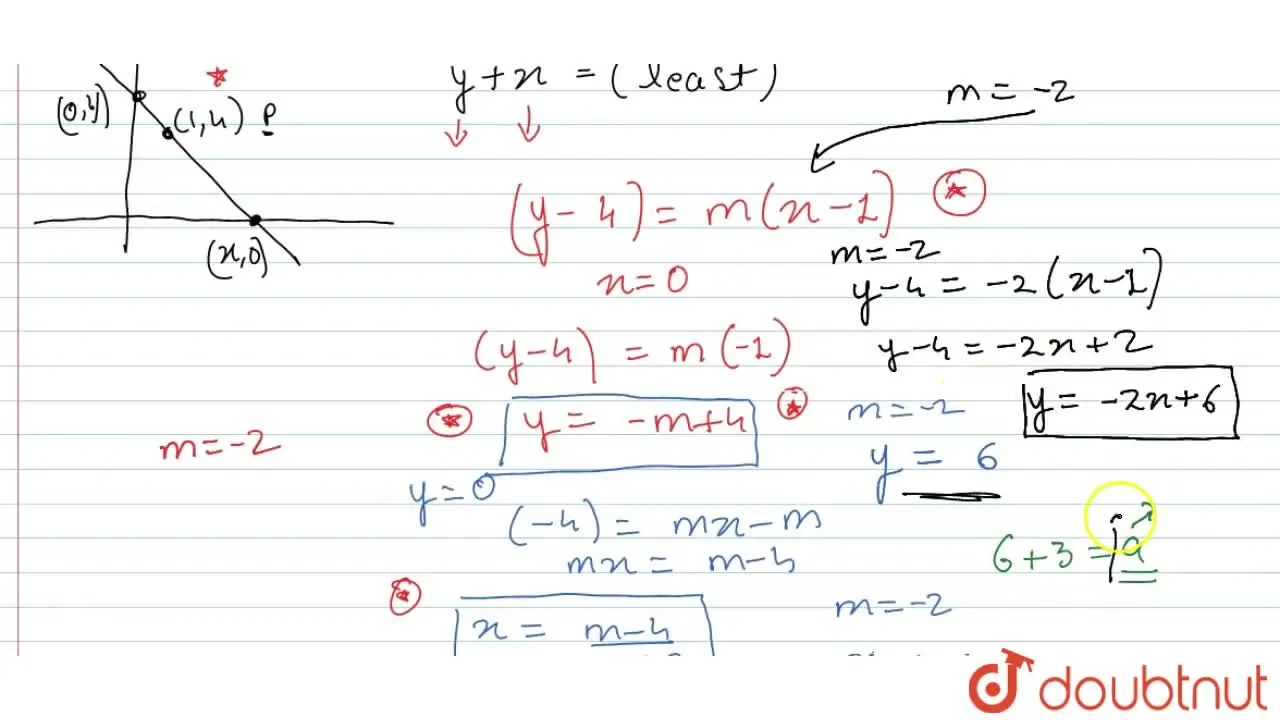
A straight line is drawn through the point P(1,4) Find the least value of the sum of intercepts made by line on the coordinates axes. Also find the equation of the line.

Answer

Least value of the sum of intercepts = 9: Equations of the line 2x+ y =6

Explanation

To solve the problem step by step, we will follow the procedure outlined in the video transcript.

Step 1: Understand the Problem
We need to find the least value of the sum of the intercepts made by a line passing through the point \( P(1, 4) \) on the coordinate axes. The intercepts on the y-axis and x-axis will be denoted as \( b \) and \( a \) respectively. We need to minimize the sum \( S = a + b \).

Step 2: Equation of the Line
The equation of a line in point-slope form through point \( P(1, 4) \) can be expressed as:
\( y - 4 = m(x - 1) \)
where \( m \) is the slope of the line.

Step 3: Find the Intercepts
To find the y-intercept (where \( x = 0 \)):
\( y - 4 = m(0 - 1) \implies y - 4 = -m \implies y = -m + 4 \)
Thus, the y-intercept \( b = -m + 4 \).

To find the x-intercept (where \( y = 0 \)):
\( 0 - 4 = m(x - 1) \implies -4 = mx - m \implies mx = m - 4 \implies x = \frac{m - 4}{m} \)
Thus, the x-intercept \( a = \frac{m - 4}{m} \).

Step 4: Sum of the Intercepts
Now we can express the sum of the intercepts:
\( S = a + b = \left(\frac{m - 4}{m}\right) + (-m + 4) \)
Simplifying this:
\( S = \frac{m - 4}{m} - m + 4 = \frac{m - 4 - m^2 + 4m}{m} = \frac{-m^2 + 5m - 4}{m} \)
This simplifies to:
\( S = -m + 5 - \frac{4}{m} \)

Step 5: Differentiate to Find Minimum
To find the minimum value of \( S \), we differentiate with respect to \( m \):
\( \frac{dS}{dm} = -1 + \frac{4}{m^2} \)
Setting the derivative to zero for minimization:
\( -1 + \frac{4}{m^2} = 0 \implies \frac{4}{m^2} = 1 \implies m^2 = 4 \implies m = \pm 2 \)

Step 6: Determine Minimum Value
To determine which value gives a minimum, we can use the second derivative test:
\( \frac{d^2S}{dm^2} = -\frac{8}{m^3} \)
- For \( m = 2 \):
\( \frac{d^2S}{dm^2} = -\frac{8}{2^3} = -1 \quad (\text{maximum}) \)
- For \( m = -2 \):
\( \frac{d^2S}{dm^2} = -\frac{8}{(-2)^3} = 1 \quad (\text{minimum}) \)

Thus, the value of \( m \) that minimizes \( S \) is \( m = -2 \).

Step 7: Calculate the Intercepts
Substituting \( m = -2 \) back into the intercept formulas:
- For y-intercept:
\( b = -(-2) + 4 = 2 + 4 = 6 \)
- For x-intercept:
\( a = \frac{-2 - 4}{-2} = \frac{-6}{-2} = 3 \)

Step 8: Calculate the Minimum Sum
Now, we find the minimum sum:
\( S = a + b = 3 + 6 = 9 \)

Step 9: Equation of the Line
Finally, substituting \( m = -2 \) into the line equation:
\( y - 4 = -2(x - 1) \implies y - 4 = -2x + 2 \implies y = -2x + 6 \)

Final Answers
- The least value of the sum of intercepts is \( 9 \).
- The equation of the line is \( y = -2x + 6 \).