A weighted glass tube is floated in a liquid with 20 cm of its length immersed. It is pushed down through a certain distance and then released. Compute the time period of its vibration.
Height to which the tube is immersed in the liquid
= h=20 cm
Let the mass of the tube bem and the density of the liquid bep. The tube floats when the weight of the tube equals the upthrust (see Fig.)
mg=20×A×ρg
m=20×Aρ where A is the area of cross-section of the tube.
Let y be the depth through which the tube is pushed into the liquid.
Restoring force on the tube = yAρg
Acceleration of the tube =yAρg20Aρ
= yg20
The acceleration is proportional to the displacement. So the motion of the tube is shm. The period of oscillation is given by
T=2π√DisplacementAcceleration
=2π√yyg/20
y=20cm0.20m
T=2π√0.29.8
=2×3.14√0.29.8=0.897s
Topper's Solved these Questions
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - ADDITIONAL SOLVED PROBLEMS11videos
OSCILLATIONS
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SHORT ANSWER QUESTIONS WITH ANSWER20videos
OSCILLATIONS
- BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS (FROM POISEUILLE.S FORMULA)19videos
MOTION IN FLUIDS
- BOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 4 ( TEMPERATURE ) UNSOLVED PROBLEMS12videos
PROPERTIES OF MATTER
Similar Questions
A pole is floating in a liquid with 80 cm of its length immersed. It is pushed doun a certain distance and then released. Time period of vertical oscillation is
View SolutionA spring elongated by length 'L' when a mass 'M' is suspended to it. Now a tiny mass 'm' is attached and then released, its time period of oscillation is -
View SolutionA cuboidal piece of wood has dimensions a, b and c. its relatively density is d. it is floating in a large body of water such that side a is vertical. It is pushed down a bit and released. The time period of SHM executed by it is
View SolutionA test tube of length l and area of cross-section A has some iron fillings of mass M. The test tube floats normally in a liquid of density ρ with length x dipped in the liquid. A disturbing force makes the tube oscillate in the liquid. The time period of oscillation is given by (neglect the mass of the test tube)
View SolutionMercury fills a glass tube Fig. so that the total column is 20 cm long. The tube is then rocked, so that the mercury begins to oscillate. Find the frequency and the period of the vibrations.
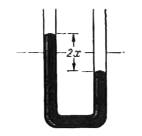View SolutionA cylindrical object of outer diameter 20 cm and mass 2 kg floats in water with its axis vertical. If it is slightly depressed and then released, find the time period of the resulting simple harmonic motion of the object.
View SolutionA solid cylinder of denisty ρ0, cross-section area A and length l floats in a liquid ρ(>ρ0 with its axis vertical, . If it is slightly displaced downward and released, the time period will be :
View SolutionA cylindrical of wood (density =600kgm−3) of base area 30cm2 and height 54cm, floats in a liquid of density 900kg−3 The block is deapressed slightly and then released. The time period of the resulting oscillations of the block would be equal to that of a simple pendulum of length (nearly) :
View SolutionA uniform disc of radius 5.0 cm and mass 200g is fixed at its centre to a metal wire, the other end of which is fixed with a clamp. The hanging disc is rotated about the wire through an angle and is released. If the disc makes torsional oscillations with time period 0.20s, find the torsional constant of the wire.
View SolutionA block of wood of volume 30cm3 floats it water with 20cm3 of its volume immersed.
Calculate the density.View Solution