In an experiment to determine the thermal conductivity of copper with Searle's apparatus, 100 gm of water flowed in 4.2 minutes past the cold end of the copper bar, its initial and final temperatures being 25°C and 52°C respectively. If the temperature difference between two points in the bar 10 cm apart is 23°C and the area of cross-section of the bar is 5 cm2, calculate the coefficient of thermal conductivity of copper. Specific heat capacity of water 4,200J kg−1K−1
To calculate the coefficient of thermal conductivity of copper using the given data, we will follow these steps:
Step 1: Calculate the heat gained by water (Q)
The formula to calculate the heat gained by water is:
Q=mcΔT
Where:
- m = mass of water (in kg)
- c = specific heat capacity of water (in J/kg·K)
- ΔT = change in temperature (in K)
Given:
- Mass of water, m=100g=0.1kg
- Specific heat capacity of water, c=4200J/kg⋅K
- Initial temperature, Ti=25∘C
- Final temperature, Tf=52∘C
Now, calculate ΔT:
ΔT=Tf−Ti=52−25=27K
Now substitute the values into the equation for Q:
Q=0.1kg×4200J/kg⋅K×27K
Calculating Q:
Q=0.1×4200×27=11340J
Step 2: Convert the area of cross-section to square meters
Given:
- Area of cross-section, A=5cm2
Convert A to square meters:
A=5cm2=5×10−4m2
Step 3: Identify the temperature difference and distance
Given:
- Temperature difference, θ1−θ2=23∘C (which is equal to 23 K)
- Distance between the two points, d=10cm=0.1m
Step 4: Convert time to seconds
Given:
- Time, t=4.2minutes
Convert time to seconds:
t=4.2minutes×60seconds/minute=252seconds
Step 5: Use the formula for thermal conductivity (K)
The formula for thermal conductivity is given by:
K=QdA(θ1−θ2)t
Substituting the values we have:
K=11340J×0.1m(5×10−4m2)×23K×252s
Calculating the denominator:
=(5×10−4)×23×252=0.0028956m2⋅K⋅s
Now substituting back into the equation for K:
K=11340.0028956
Calculating K:
K≈292.3W/m⋅K
Final Answer:
The coefficient of thermal conductivity of copper is approximately 292.3W/m⋅K.
---
- m = mass of water (in kg)
- c = specific heat capacity of water (in J/kg·K)
- ΔT = change in temperature (in K)
- Mass of water, m=100g=0.1kg
- Specific heat capacity of water, c=4200J/kg⋅K
- Initial temperature, Ti=25∘C
- Final temperature, Tf=52∘C
- Area of cross-section, A=5cm2
- Temperature difference, θ1−θ2=23∘C (which is equal to 23 K)
- Distance between the two points, d=10cm=0.1m
- Time, t=4.2minutes
The coefficient of thermal conductivity of copper is approximately 292.3W/m⋅K.
Topper's Solved these Questions
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - CONCEPTUAL SHORT ANSWER QUESTIONS WITH ANSWERS
8 Videos
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - LONG ANSWER QUESTIONS
5 Videos
- SELF ASSESSMENT PAPER 5BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 5EXERCISE - Section-D
6 Videos
- THERMAL RADIATION BOOK - ICSECHAPTER - THERMAL RADIATION EXERCISE - SELECTED PROBLEMS (from WIEN.S DISPLACEMENT LAW)
14 Videos
Similar Questions
Describe an experiment to determine the coefficient of thermal conductivity of a good conductor by Searle's method.
View SolutionIf the temperature difference on the two sides of a wall increases from 100∘C to 200∘C, its thermal conductivity
View SolutionThe temperature difference between the ends of a rod of aluminium of length 1.0 m and area of cross section 5.0 cm2 is 200∘C. How much heat will flow through the rod in 5 minutes ? The coefficient of thermal conductivity of aluminium is 0.2 kJ s−1 m−1 ∘C−1.
View Solution0.5N solution of a salt placed between two Pt electrodes 2.0 cm apart and having area of cross-section 2.5 cm2 has resistance of 25 ohms. Calculate the conductance and cell constant.
View SolutionThe thickness of a metallic plate is 0.4 cm. The temperature between its two surfaces is 20∘C. The quantity of heat flowing per second is 50 calories from 5cm2 area. In CGS system, the coefficient of thermal conductivity will be
View SolutionThe thickness of a metallic plate is 0.4 cm. The temperature between its two surfaces is 20∘C. The quantity of heat flowing per second is 50 calories from 5cm2 area. In CGS system, the coefficient of thermal conductivity will be
View SolutionIn an experiment to find the thermal conductivity of rubber, a tube of length 10 cm with external radius 0.5 cm and internal radius 0.3 cm is immersed in 0.28 g of water at 30°C contained in a copper calorimeter of mass 0.20 kg and specific heat capacity 385JKg−1K−1. Through the tube, steam is passed for 10 minutes and the final maximum temperature of water and calorimeter is 42∘C. Calculate the thermal conductivity of rubber. Specific heat capacity of water =4200JKg−1K−1.
View Solution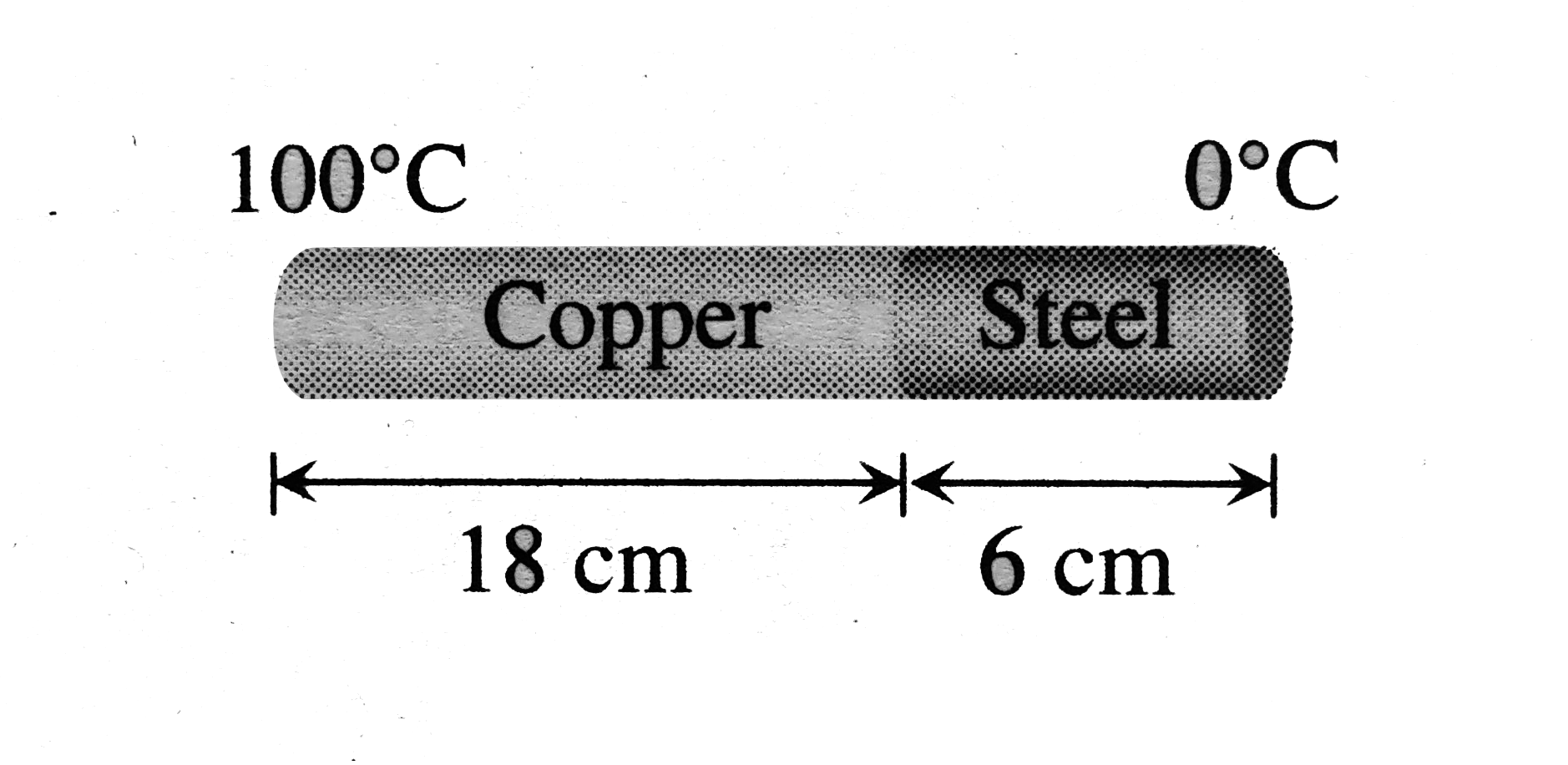
The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?
View SolutionThree bars of equal lengths and equal area of cross-section are connected in series fig. their thermal conducitives are in the ratio 2:3:4. If at the steady state the open ends of the first and the last bars are at temperature 200∘C and 20∘C respectively, find the temperature of both the junctions.
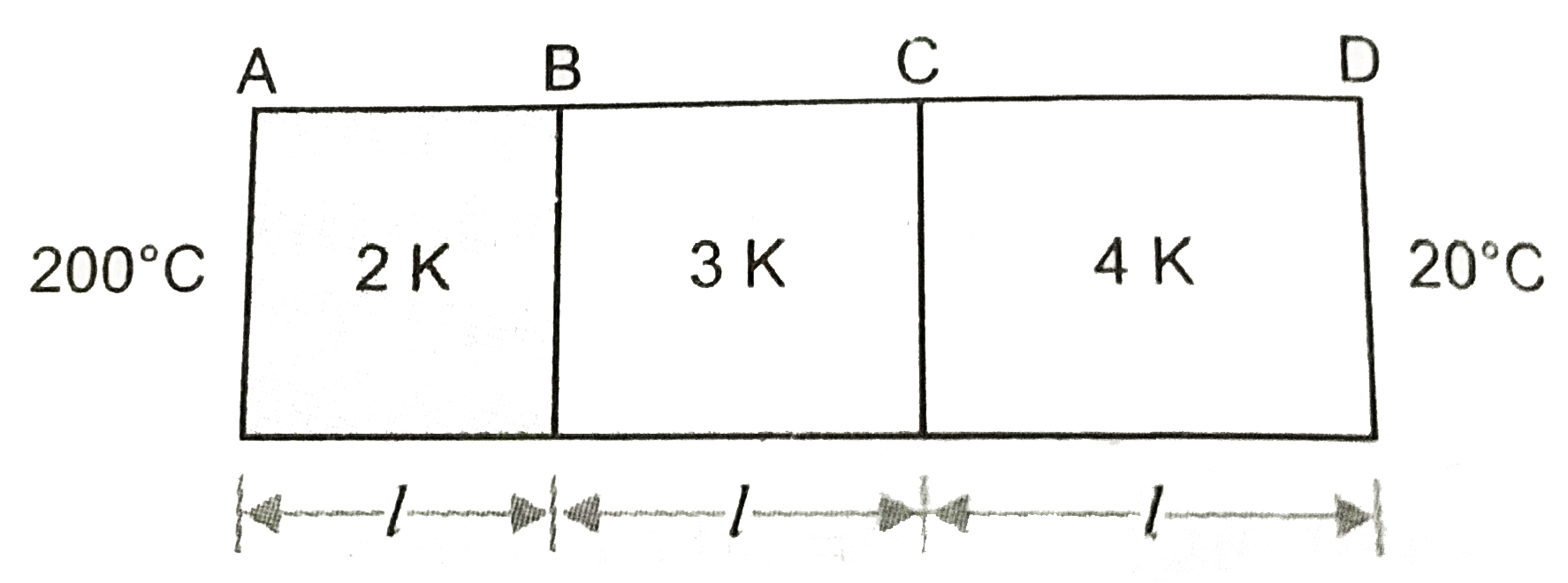 .
.
View SolutionIn a steady state of thermal conduction, temperature of the ends A and B of a 20 cm long rod are 100∘C and 0∘C respectively. What will be the temperature of the rod at a point at a distance of 9 cm from the end A of the rod
View Solution

