A rhombus is symmetrical about
each of its two diagonals
each of its two lines joining the mid-points of opposite sides
each of the perpendicular bisector of its sides
none of these
The correct Answer is:a
To solve the question "A rhombus is symmetrical about", we will analyze the properties of a rhombus and determine its lines of symmetry step by step.
1. Understanding the Shape of a Rhombus:
- A rhombus is a type of quadrilateral where all four sides are of equal length.
- It has opposite angles that are equal, and adjacent angles are supplementary (they add up to 180 degrees).
Hint: Remember that in a rhombus, all sides are equal, which is a key property.
2. Drawing the Rhombus:
- Let's draw a rhombus and label its vertices as A, B, C, and D in a clockwise manner.
Hint: Label the vertices clearly to help visualize the diagonals.
3. Identifying the Diagonals:
- The diagonals of a rhombus are the lines that connect opposite vertices. In our rhombus, the diagonals are AC and BD.
Hint: Diagonals are important as they help us find the lines of symmetry.
4. Properties of the Diagonals:
- The diagonals of a rhombus bisect each other at right angles (90 degrees).
- Each diagonal divides the rhombus into two congruent triangles, meaning they are mirror images of each other.
Hint: Remember that bisecting means dividing into two equal parts.
5. Finding Lines of Symmetry:
- Since each diagonal divides the rhombus into two symmetric parts, this means that the rhombus has two lines of symmetry: one along diagonal AC and another along diagonal BD.
Hint: Lines of symmetry are lines where one side is a mirror image of the other.
6. Conclusion:
- Therefore, a rhombus is symmetrical about each of its two diagonals.
Hint: The answer to the question is that the rhombus has two lines of symmetry, which are its diagonals.
Final Answer:
A rhombus is symmetrical about each of its two diagonals.
- A rhombus is a type of quadrilateral where all four sides are of equal length.
- It has opposite angles that are equal, and adjacent angles are supplementary (they add up to 180 degrees).
- Let's draw a rhombus and label its vertices as A, B, C, and D in a clockwise manner.
- The diagonals of a rhombus are the lines that connect opposite vertices. In our rhombus, the diagonals are AC and BD.
- The diagonals of a rhombus bisect each other at right angles (90 degrees).
- Each diagonal divides the rhombus into two congruent triangles, meaning they are mirror images of each other.
- Since each diagonal divides the rhombus into two symmetric parts, this means that the rhombus has two lines of symmetry: one along diagonal AC and another along diagonal BD.
- Therefore, a rhombus is symmetrical about each of its two diagonals.
A rhombus is symmetrical about each of its two diagonals.
Similar Questions
Knowledge Check
- Question 1 - Select One
A rhombus has :
Aone line of symmetryBtwo lines of symmetry eachCfour lines of symmetryDno line of symmetry - Question 2 - Select One
Which of the following graphs is symmetric about the origin ?
A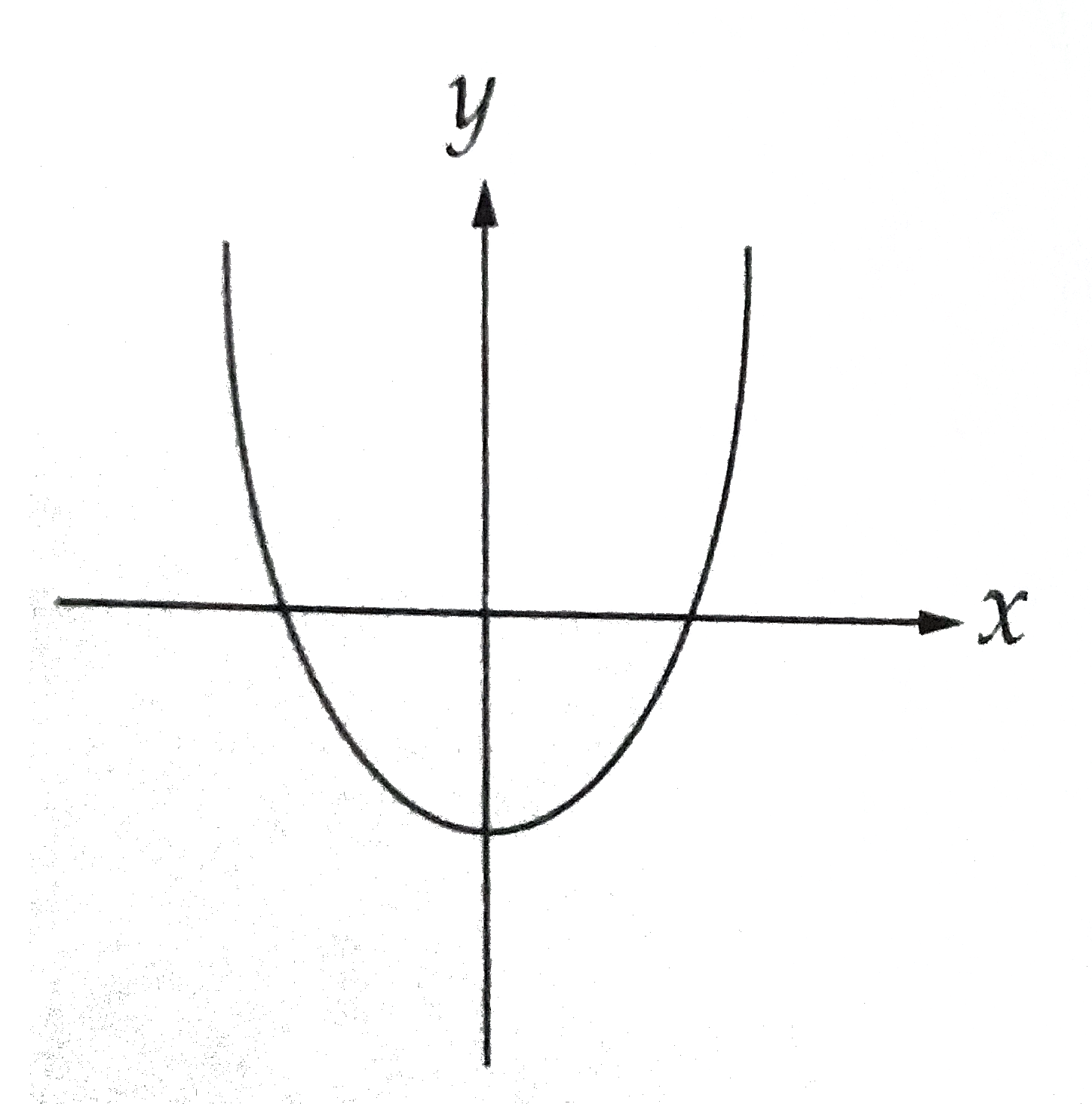 B
B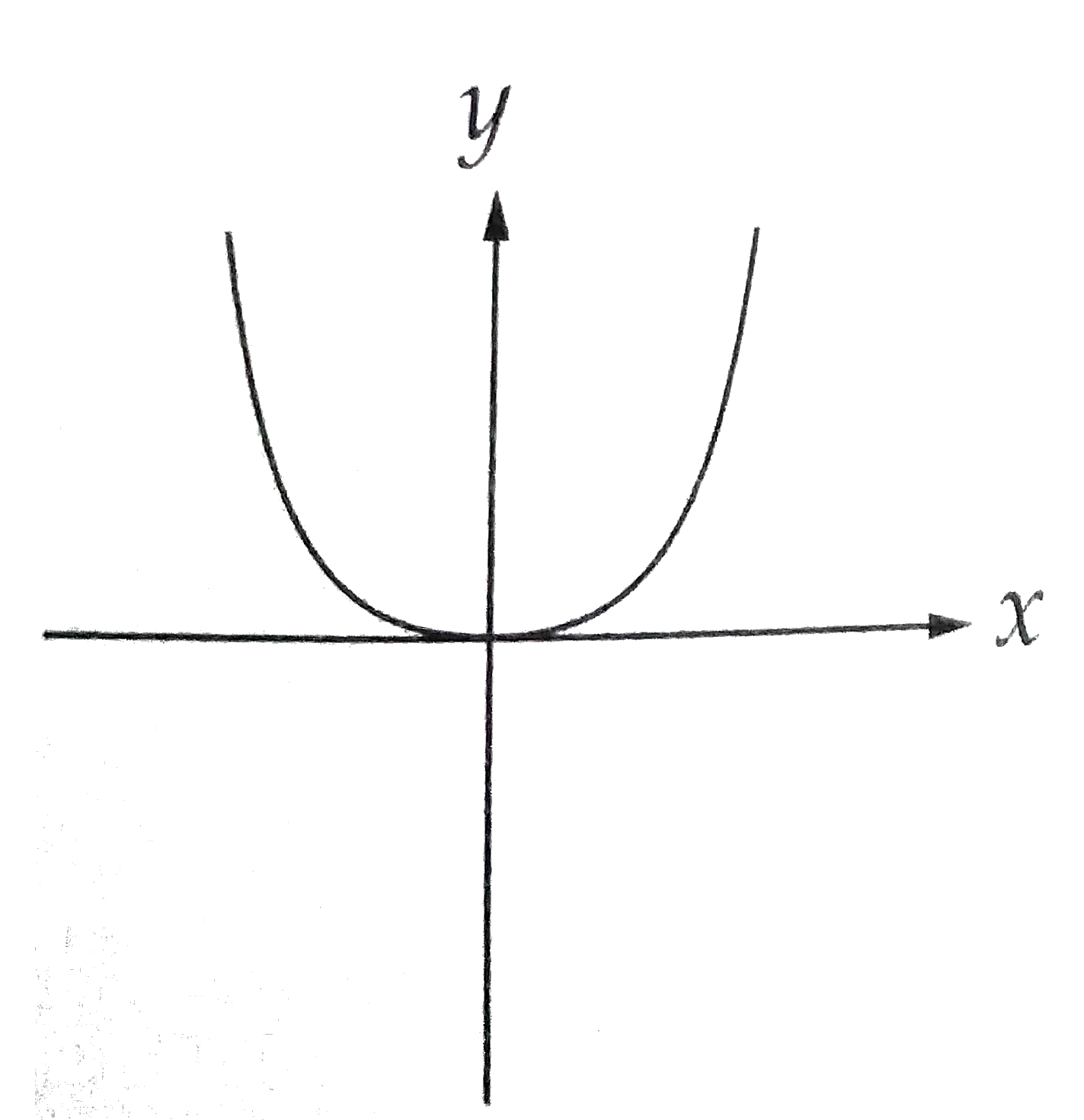 C
C D
D
- Question 3 - Select One
Which of the following shapes is not symmetrical about the horizontal line ?
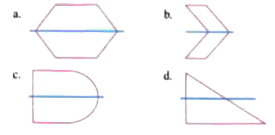 .AABBCCDD
.AABBCCDD
Statement I The graph of y=sec2x is symmetrical about the Y-axis.
Statement II The graph of y=tax is symmetrical about the origin.View SolutionStatement I The curve y=x22+x+1 is symmetric with respect to the line x=1. because Statement II A parabola is symmetric about its axis.
View SolutionIf the graph of a function f(x) is symmetrical about the line x = a, then
View SolutionIf the graph of the function y = f(x) is symmetrical about the line x = 2, then
View SolutionWhich of the following shapes is not symmetrical about the vertical line ?
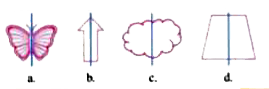 View Solution
View Solution
