A cubical block of metal weighs 6 pounds. How much will another cube of the same metal weigh if its sides are twice as long?
A) 48
B) 12
C) 36
D) 60
The correct Answer is:5x−9x=−3
To solve the problem of how much a cubical block of metal will weigh if its sides are twice as long, we can follow these steps:
Step 1: Understand the relationship between the side length and volume of a cube.
The volume V of a cube is given by the formula:
V=a3
where a is the length of a side of the cube.
Step 2: Calculate the volume of the original cube.
Let the side length of the original cube be a. The volume of the original cube is:
Voriginal=a3
Step 3: Determine the side length of the new cube.
If the sides of the new cube are twice as long, then the side length of the new cube is:
2a
Step 4: Calculate the volume of the new cube.
The volume of the new cube is:
Vnew=(2a)3=8a3
Step 5: Relate the weights of the cubes.
Since the weight of an object is proportional to its volume (assuming the same material and density), we can set up the following relationship:
Weight of new cubeWeight of original cube=VnewVoriginal
Substituting the volumes from steps 2 and 4:
Weight of new cube6 pounds=8a3a3
Step 6: Simplify the equation.
This simplifies to:
Weight of new cube6=8
Step 7: Solve for the weight of the new cube.
Multiplying both sides by 6 gives:
Weight of new cube=6×8=48 pounds
Final Answer:
The weight of the new cube is 48 pounds.
---
The volume V of a cube is given by the formula:
V=a3
where a is the length of a side of the cube.
Let the side length of the original cube be a. The volume of the original cube is:
Voriginal=a3
If the sides of the new cube are twice as long, then the side length of the new cube is:
2a
The volume of the new cube is:
Vnew=(2a)3=8a3
Since the weight of an object is proportional to its volume (assuming the same material and density), we can set up the following relationship:
Weight of new cubeWeight of original cube=VnewVoriginal
Substituting the volumes from steps 2 and 4:
Weight of new cube6 pounds=8a3a3
This simplifies to:
Weight of new cube6=8
Multiplying both sides by 6 gives:
Weight of new cube=6×8=48 pounds
The weight of the new cube is 48 pounds.
Topper's Solved these Questions
Similar Questions
If a metallic cuboid weighs 16 kg, how much would a miniature cuboid of metal weigh, if all dimensions are reduced to one-fourth of the original? (a) 0.25 kg (b) 0.50 kg (c) 0.75 kg (d) 1 kg
View SolutionA cube of side length 3 cm weighs 12 kg. What is the weight of similar cube of same material whose side length is 12 cm?
View Solution
Knowledge Check
A cubical block of metal weights 6 pounds. How much will another cube of the same metal weight if its sides are twice as long?
A48B12C36DData InadequateWhen a metal sphere is suspended at the end of a metal wire, its extension is 0.4 mm. If another metal sphere of the same material with its radius is half of the previous, is suspended then extension would be
A0.05 mmB0.02 mmC0.01 mmD0.32 mmA metal ball immersed in alcohol weighs w1 and0∘C andw2 at 59∘C. The coefficient of cubical expansion of the metal is less than that of alcohol. Assuming that the density of the metal is large compared to that of alcohol, it can be shown that
Aw1>w2Bw1=w2Cw1<w2Dw1=(w1/2)
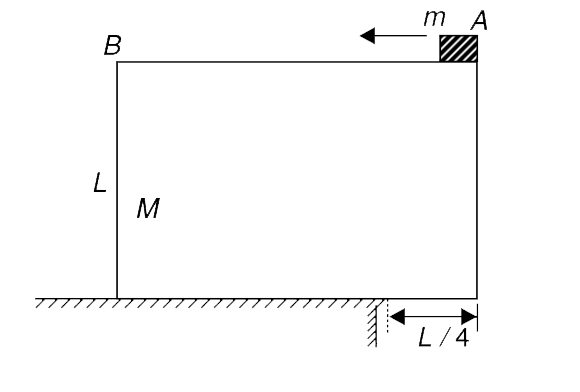
A uniform cubical block of mass M and side length L is lying on the edge of a rough table with 14th of its edge overhanging. When a small block of mass m is placed on its top surface at the right edge (see fig.), the cube is on verge of toppling. The block of mass m is given a sharp horizontal impulse so that it acquires a velocity towards B. The small block moves on the top surface and falls on the other side. What is maximum coefficient of friction between the small block and the cube so that the cube does not rotate as the block moves over it. Assume that the friction between the cube and the table is large enough to prevent sliding of the cube on the table.
View SolutionTwo positions of a cubical block are given below, each face having a number of small triangles. In another position of the cube, if there is one triangle at the bottom, how many triangles will be there on the top face?

View SolutionAn irregular piece of metal weighs 10.00g in air and 8.00g whaen submerged in water
(a) Find the volume of the metal and its density.
(b) If the same piece of metal weighs 8.50g when immersed in a particular oil. What is the density of the oil?
View SolutionA bullet of mass 4 g is fired with a velocity of 600 ms−1 into a block of metal weighing 9.996kg. The block is free to move. Calculate the common velocity of the bullet and block after the impact.
View SolutionA solid cube of each side 10 cm, has been painted red, blue and black on pairs of opposite faces. It is then cut into cubical blocks of each side 2 cm. Answer the following questions.
How many cubes have two faces painted red and black and all other faces unpainted?
View Solution
