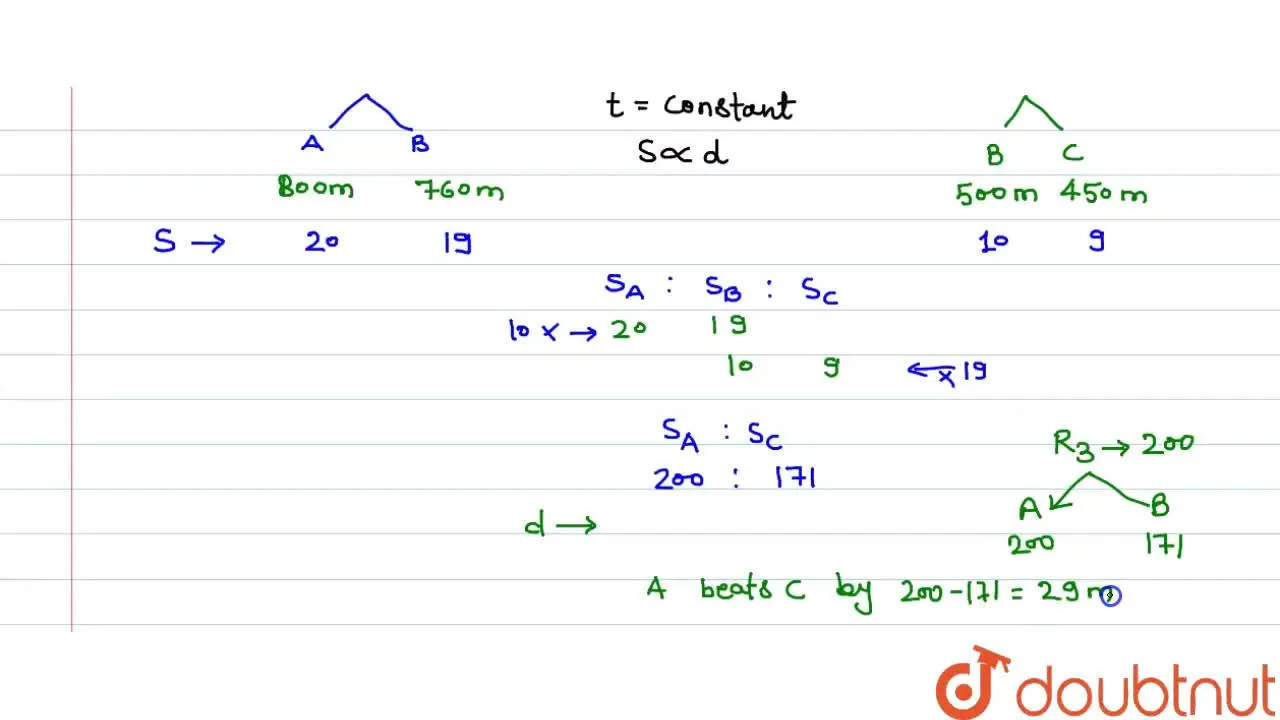
In a race of 800 m, A can beat B by 40 m. In a race of 500 m, B can beat C by 50 m. In a race of 200 m, By what margin will A beat C.
The correct Answer is:11.9m
Step by step video, text & image solution for In a race of 800 m, A can beat B by 40 m. In a race of 500 m, B can beat C by 50 m. In a race of 200 m, By what margin will A beat C. by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
In a race of 1000 m, A can beat B by 100 m. In a race of 400 m, B beat C by 40 m. In a race of 500 m, By what margin will A beat C.
View SolutionIn a race of 800 m, A can beat B by 40 m. In a race of 500 m, B can beat C by 5 m. In a race of 200 m, A will beat C by
View SolutionIn a race of 1000 m, A can beat B by 100 m. In a race of 400 m, B beat C by 40 m. In a race of 500 m, By what margin will A beat C.
View SolutionIn a race of 1km A beats B by 100m in a race of 300m B beats C by 50m. Then in a race of 1 km by what margin will A beat C?
View SolutionIn a race of 600 m, A can beat B by 60 m and in a race of 500 m, B can beat C by 50 m. By how many metres will A beat C in a race of 400 m ?
View SolutionIn a race of 600 m, A can beat B by 60 m and in a race of 500 m, B can beat C by 50 m. By how many metres will A beat C in a race of 400 m ?
View SolutionIn a race of 1200m, A can beat B by 120 m and in a race of 500 m, B can beat C by 100 m. By how many metres will A beat C in a race of 800 m?
View SolutionIn a race of 600 m, A can beat B by 60 m and in a race of 500 m, B can beat C by 25m . By how many metres will A beat C in a 400 m race?
View SolutionIn a race of 600 m ,A can beat B by 30 m and in a race of 500 m, B can beat C by 25m .By how many metres will A beat C in a race of 400 m?
View SolutionIn a 1000 m race, A can beat B by 100 m. In a race of 400 m, B can beat C by 40 m. By how many metres will A beat C in a race of 500 m? (a) 85 m (b) 95 m (c) 105 m (d) 115 m
View Solution