A simple cubic lattice consists of eight identical spheres of radius R in contact, placed at the corners of a cube. What is the volume of the cubical box that will just enclose these eight spheres and what fraction of this volume is actually occupied by the spheres?
The correct Answer is:64R3,52.36%
To solve the problem, we need to determine two things: the volume of the cubical box that encloses the eight spheres and the fraction of this volume that is occupied by the spheres.
Step 1: Determine the side length of the cube
In a simple cubic lattice, the spheres are placed at the corners of the cube. Each corner sphere touches the adjacent corner spheres. The distance between two opposite corners of the cube is equal to four times the radius of the spheres (since there are two radii from each sphere at the corners).
Given that the radius of each sphere is R, the relationship between the side length a of the cube and the radius R is:
a=2R
Step 2: Calculate the volume of the cube
The volume V of a cube is given by the formula:
V=a3
Substituting a=2R:
V=(2R)3=8R3
Step 3: Calculate the volume occupied by the spheres
Each sphere has a volume given by the formula:
Volume of one sphere=43πR3
Since there are 8 spheres, the total volume occupied by the spheres is:
Total volume occupied=8×43πR3=323πR3
Step 4: Calculate the fraction of the volume occupied by the spheres
The fraction of the volume of the cube that is occupied by the spheres can be calculated as:
Fraction occupied=Volume occupied by spheresVolume of the cube=323πR38R3
Simplifying this gives:
Fraction occupied=32π3×8=4π3
Calculating the numerical value:
4π3≈0.4189
Final Results
1. Volume of the cubical box: 8R3
2. Fraction of the volume occupied by the spheres: 4π3≈0.4189
a=2R
V=a3
Substituting a=2R:
V=(2R)3=8R3
Volume of one sphere=43πR3
Since there are 8 spheres, the total volume occupied by the spheres is:
Total volume occupied=8×43πR3=323πR3
Fraction occupied=Volume occupied by spheresVolume of the cube=323πR38R3
Simplifying this gives:
Fraction occupied=32π3×8=4π3
Calculating the numerical value:
4π3≈0.4189
2. Fraction of the volume occupied by the spheres: 4π3≈0.4189
Topper's Solved these Questions
- SOLID AND LIQUID STATES BOOK - RC MUKHERJEECHAPTER - SOLID AND LIQUID STATES EXERCISE - OBJECTIVE PROBLEMS
51 Videos
- SOLID AND LIQUID STATES BOOK - RC MUKHERJEECHAPTER - SOLID AND LIQUID STATES EXERCISE - OBJECTIVE PROBLEMS
51 Videos
- PROPERTIES OF GASESBOOK - RC MUKHERJEECHAPTER - PROPERTIES OF GASESEXERCISE - OBJECTIVE PROBLEMS
5 Videos
- VOLUMETRIC CALCULATIONS BOOK - RC MUKHERJEECHAPTER - VOLUMETRIC CALCULATIONS EXERCISE - OBJECTIVE PROBLEMS
37 Videos
Similar Questions
Knowledge Check
A solid metallic sphere of radius 4 cm is melted and recast into '4' identical cubes. What is the side of the cube?
A7√π3 cmB53√π3cmC63√π3cmD43√π3 cmA solid metallic sphere of radius 4 cm is melted and recast into '4' identical cubes. What is the side of the cube?
A73√π3cmB53√π3cmC63√π3cmD43√π3cmA solid metallic sphere of radius 4 cm is melted and recast into '4' identical cubes. What is the side of the cube?
A7 3√π3cmB5 3√π3cmC6 3√π3cmD4 3√π3cm
If the radius of a sphere is doubled, what is the ratio of the volume of the first sphere to that of the second sphere?
View SolutionThe error in the measurement of radius of a shpere if 2%. What would be the error in the volume of the sphere?
View SolutionWhat are the dimensions of :
a. volume of a cube of edge a,
b. volume of a sphere of radius a,
c. the ratio of the volume of a cube of edge a to the volume of a sphere of radius a ?
View SolutionSimple cubic structure represented below .
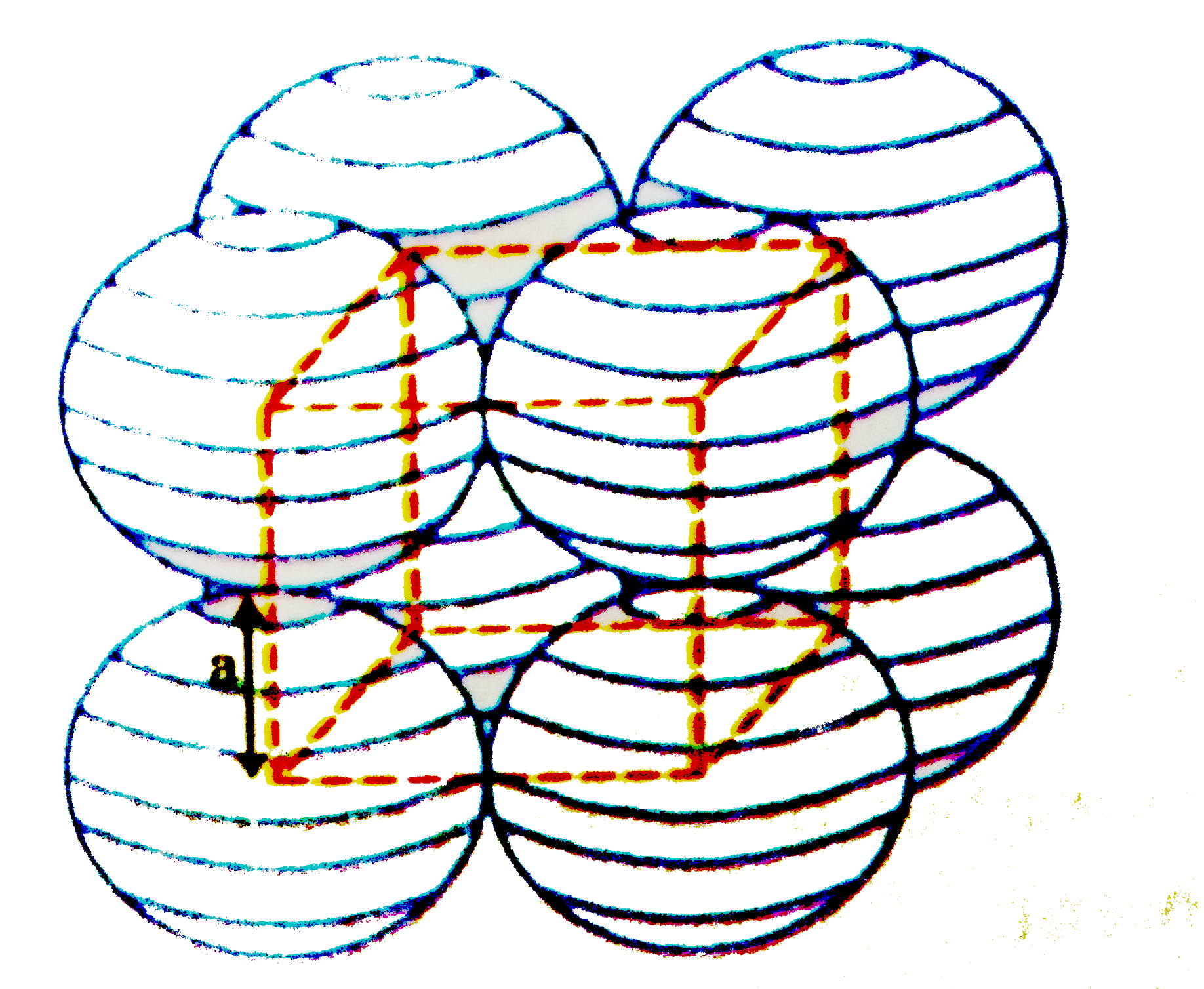
What is the volume of the entire cube ?
View SolutionTwo identical spheres are placed in contact with each other. The force of gravitation between the spheres will be proportional to ( R = radius of each sphere)
View Solution
