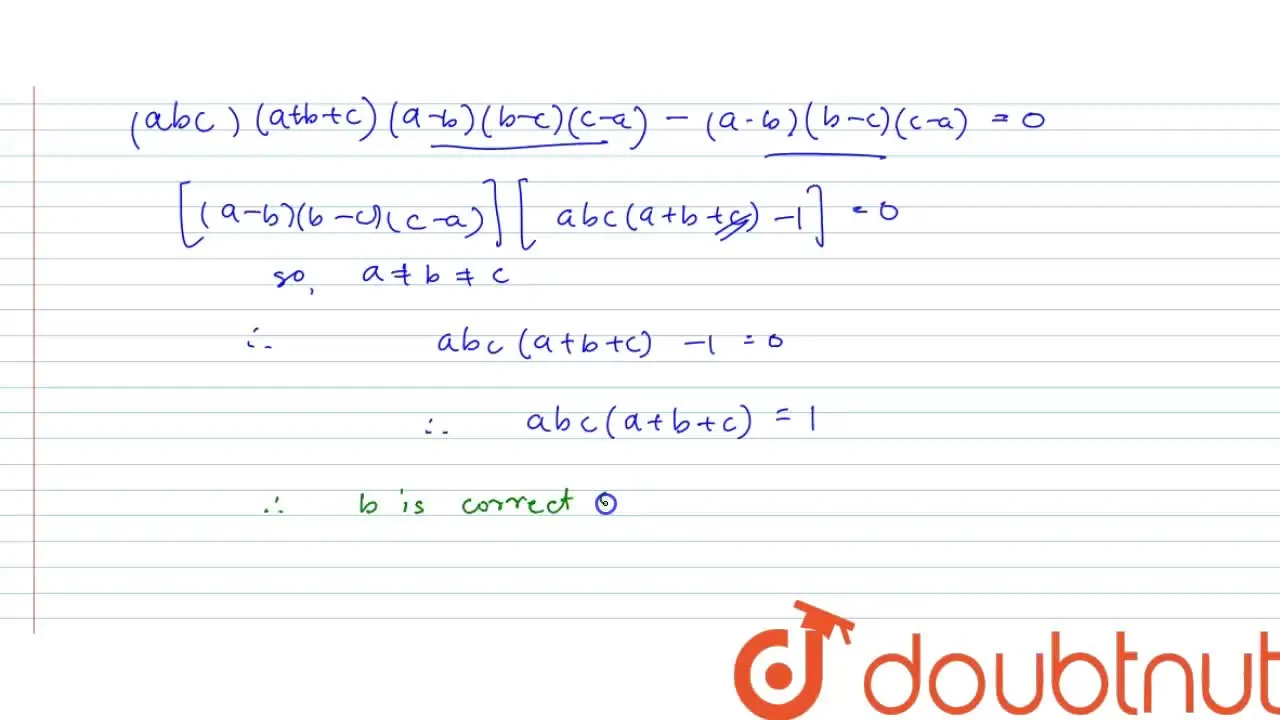
If a,b,c are distinct real numbers and ∣∣
∣
∣∣aa2a4−1bb2b4−1cc2c4−1∣∣
∣
∣∣=0 then
A)
a
b
c
=
1
B
)
a
b
c
(
a
+
b
+
c
)
=
1
C
)
a
b
c
(
a
+
b
+
c
)
=
a
b
+
b
c
+
c
a
D) none of these
A)abc=1
B)abc(a+b+c)=1
C)abc(a+b+c)=ab+bc+ca
D) none of these
The correct Answer is:B
Step by step video, text & image solution for If a,b,c are distinct real numbers and |{:(a,a^(2),a^(4)-1),(b,b^(2),b^(4)-1),(c,c^(2),c^(4)-1):}|=0 then A) a b c = 1 B ) a b c ( a + b + c ) = 1 C ) a b c ( a + b + c ) = a b + b c + c a D) none of these by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ICSECHAPTER - DETERMINANTS EXERCISE - Multiple Choice Questions37videos
DETERMINANTS
- BOOK - ICSECHAPTER - CONTINUITY AND DIFFERENTIABILITY EXERCISE - MULTIPLE CHOICE QUESTIONS56videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - ICSECHAPTER - GEOMETERYEXERCISE - Multiple choice questions (Assertion and Reason based questions)2videos
GEOMETERY
Similar Questions
If a,b,c are distinct real numbers and ∣∣ ∣ ∣∣aa2a3−1bb2b3−1cc2c3−1∣∣ ∣ ∣∣=0 then
View SolutionIf a,b,c are all distinct and ∣∣ ∣ ∣∣aa3a4−1bb3b4−1cc3c4−1∣∣ ∣ ∣∣ =0, show that abc(ab+bc+ac) = a+b+c
View SolutionIf a,b,c are all distinct and ∣∣ ∣ ∣∣aa3a4−1bb3b4−1cc3c4−1∣∣ ∣ ∣∣ =0, show that abc(ab+bc+ac) = a+b+c
View SolutionIf a,b,c are all distinct and ∣∣ ∣ ∣∣aa3a4−1bb3b4−1cc3c4−1∣∣ ∣ ∣∣ =0, show that abc(ab+bc+ac) = a+b+c
View SolutionIf a,b,c are all different and ∣∣ ∣ ∣∣aa3a4−1bb3b4−1cc3c4−1∣∣ ∣ ∣∣=0, show that abc(ab+bc+ca)=a+b+c
View SolutionIf a, b, c are distinct positive real numbers such that a+1b=4,b+1c=1,c+1d=4 and d+1a=1, then
View SolutionIf a, b, c are three distinct positive real numbers, then the least value of
(1+a+a2)(1+b+b2)(1+c+c2)abc, isView SolutionIf a,b,c are real numbers such that 3(a2+b2+c2+1)=2(a+b+c+ab+bc+ca), than a,b,care in
View SolutionIf a,b,c are positive real numbers and 2a+b+3c=1, then the maximum value of a4b2c2 is equal to
View SolutionProve that: a+b+ca−1 b−1+b−1 c−1+c−1a−1=abc
View Solution