The side of a triangle are in the ratio 1:√3:2, then the angles of the triangle are in the ratioSolution in Telugu
1:3:5
2:3:4
3:2:1
1:2:3
The correct Answer is:D
Step by step video, text & image solution for The side of a triangle are in the ratio 1 : sqrt3 : 2, then the angles of the triangle are in the ratio by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - LECTURE SHEET EXERCISE - I (More than One correct answer Type Questions)
4 Videos
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - LECTURE SHEET EXERCISE - I (Matrix Matching Type Questions)
1 Videos
- PROPERTIES OF TRIANGLESBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF TRIANGLESEXERCISE - PRACTICE EXERCISE
132 Videos
- PLANESBOOK - AAKASH SERIESCHAPTER - PLANESEXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS
30 Videos
- PROPERTIES OF VECTORSBOOK - AAKASH SERIESCHAPTER - PROPERTIES OF VECTORSEXERCISE - PRACTICE EXERCISES
55 Videos
Similar Questions
If the sines of the angle A and B of a triangle ABC satisfy the equation c2x2−c(a+b)x+ab=0 . Then the triangle
View SolutionIn a ΔABC,BC=2,CA=1+√3 and ∠C=60∘. Feet of the perpendicular from A, B and on the opposite sides BC, CA and AB are D, E and F respectively and perpendiculars are concurrerant at P.
Now match the entries of Column-I with respective entries of column-II.
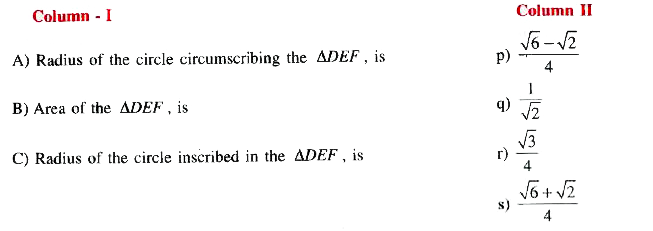
View SolutionIf a = 3, b =4 , sin A = 3/4 then ∠B=
View SolutionThe plane 2x + 3y + kz - 7 = 0 is parallel to the line whose d.r's are (2,-3,1) then k=
View SolutionIf ¯¯¯¯¯¯OA=¯i+2¯j−3¯k,¯¯¯¯¯¯OB=3¯i−2¯j+5¯kand2¯¯¯¯¯¯AC=3¯¯¯¯¯¯AB then ¯¯¯¯¯¯AC=
View SolutionIf the angle opf a triangle are in the ratio 1:2:3, then show that the sides opposite to the respective angle are in the ratio 1:√3:2.
View Solutionत्रिभुज ABC की भुजाओं का अनुपात 1:√3:2 है तब A : B : C का अनुपात है|
View SolutionThe ratio of the sides of a triangle ABC is 1:√3:2. Then the ratio A:B:C is-
View SolutionIf the sides of a triangle are in the ratio 1 : √3 : 2, then the angles of the triangle are in the ratio
View SolutionThe side of a triangle are in the ratio 1:√3:2, then the angles of the triangle are in the ratio
View SolutionIf the three angles in a triangle are in the ratio 1:2:3, then prove that the corresponding sides are in the ratio 1:√3:2.
View SolutionThe sides of a triangle are in the ratio 1:√3:2. Then the angles are in the ratio
View SolutionThe ratio of the sides of a triangle ABC is 1:√3:2. The ratioA:B:Cis
View SolutionThe sides of a triangle are in the ratio 1:√3:2 then the angles of the triangle are in the ratio
View SolutionIf the angle opf a triangle are in the ratio 1:2:3, then show that the sides opposite to the respective angle are in the ratio 1:√3:2.
View Solution
