Question

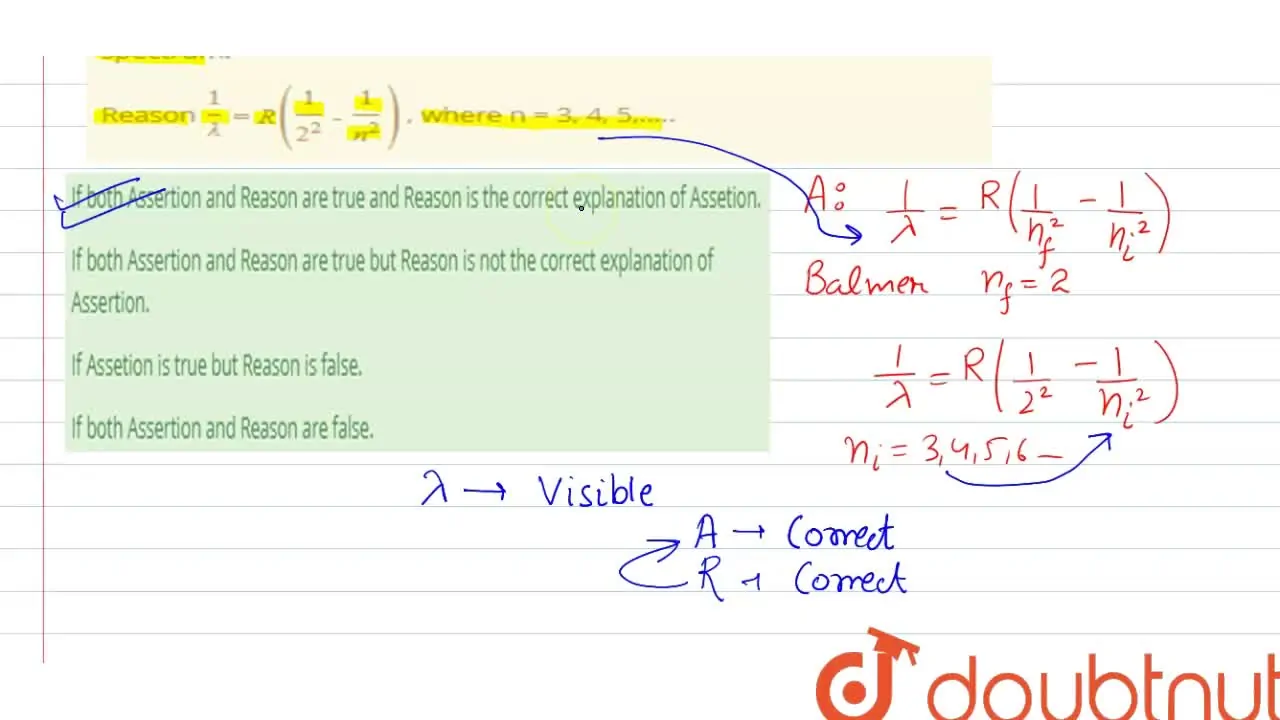
Assertion Balmer series lies in the visible region of electromagnetic spectrum.
Reason $\frac{1}{\lambda }=R(\frac{1}{2^2}-\frac{1}{n^2})$ , where n = 3, 4, 5,.....

Options

A. If both Assertion and Reason are true and Reason is the correct explanation of Assetion.
B. If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
C. If Assetion is true but Reason is false.
D. If both Assertion and Reason are false.

Answer

A

Explanation

To solve the question, we will analyze both the assertion and the reason provided.

Step 1: Understanding the Assertion
The assertion states that the Balmer series lies in the visible region of the electromagnetic spectrum. The Balmer series corresponds to the transitions of electrons in a hydrogen atom where the final energy level (nf) is 2.

Step 2: Understanding the Reason
The reason provided is the formula for the wavelength of the emitted radiation during the transitions in the hydrogen atom:
\( \frac{1}{\lambda} = R \left( \frac{1}{2^2} - \frac{1}{n^2} \right) \)
where \( n = 3, 4, 5, \ldots \). Here, \( R \) is the Rydberg constant.

Step 3: Applying the Formula
For the Balmer series, we set \( nf = 2 \). The initial energy levels (ni) can take values starting from 3 (n = 3, 4, 5, ...). We can calculate the wavelengths for these transitions:

1. For \( n = 3 \):
\( \frac{1}{\lambda} = R \left( \frac{1}{2^2} - \frac{1}{3^2} \right) = R \left( \frac{1}{4} - \frac{1}{9} \right) \)
Calculate \( \frac{1}{4} - \frac{1}{9} \):
\( \frac{1}{4} = \frac{9}{36}, \quad \frac{1}{9} = \frac{4}{36} \implies \frac{1}{4} - \frac{1}{9} = \frac{9 - 4}{36} = \frac{5}{36} \)
So,
\( \frac{1}{\lambda} = R \cdot \frac{5}{36} \)

2. For \( n = 4 \):
\( \frac{1}{\lambda} = R \left( \frac{1}{2^2} - \frac{1}{4^2} \right) = R \left( \frac{1}{4} - \frac{1}{16} \right) \)
Calculate \( \frac{1}{4} - \frac{1}{16} \):
\( \frac{1}{4} = \frac{4}{16} \implies \frac{1}{4} - \frac{1}{16} = \frac{4 - 1}{16} = \frac{3}{16} \)
So,
\( \frac{1}{\lambda} = R \cdot \frac{3}{16} \)

3. For \( n = 5 \):
\( \frac{1}{\lambda} = R \left( \frac{1}{2^2} - \frac{1}{5^2} \right) = R \left( \frac{1}{4} - \frac{1}{25} \right) \)
Calculate \( \frac{1}{4} - \frac{1}{25} \):
\( \frac{1}{4} = \frac{25}{100}, \quad \frac{1}{25} = \frac{4}{100} \implies \frac{1}{4} - \frac{1}{25} = \frac{25 - 4}{100} = \frac{21}{100} \)
So,
\( \frac{1}{\lambda} = R \cdot \frac{21}{100} \)

Step 4: Conclusion
From the calculations, we can see that for \( n = 3, 4, 5 \), the wavelengths calculated will fall within the visible spectrum (approximately 400 nm to 700 nm). Therefore, the assertion is correct.

Final Answer
Both the assertion and reason are true, and the reason correctly explains the assertion.

---