Atomic number of of silver metal is 47. calculate the speed at which a beam of protons has to be fired at a sheet of silver foil if the protons were able to approach to whithin 2.5×10−14 m of the silver nucleus?
To solve the problem of calculating the speed at which a beam of protons has to be fired at a sheet of silver foil, we will use the principles of electrostatics and conservation of energy. Here’s a step-by-step solution:
Step 1: Identify the Given Data
- Atomic number of silver (Z) = 47
- Distance from the nucleus (r) = 2.5×10−14 m
- Mass of a proton (m) = 1.67×10−27 kg
- Charge of a proton (e) = 1.6×10−19 C
Step 2: Calculate the Charge of the Silver Nucleus
The total charge (Q) of the silver nucleus can be calculated using the formula:
Q=Z⋅e
Substituting the values:
Q=47⋅(1.6×10−19)=7.52×10−18 C
Step 3: Use the Conservation of Energy Principle
The kinetic energy (KE) of the protons must equal the electrostatic potential energy (PE) at the distance r:
KE=PE
12mv2=k⋅Q⋅er
Where k is Coulomb's constant, approximately 9×109N m2/C2.
Step 4: Rearranging the Equation
Rearranging the equation to solve for v:
v2=2k⋅Q⋅em⋅r
v=√2k⋅Q⋅em⋅r
Step 5: Substitute the Values
Substituting the known values into the equation:
v=
⎷2⋅(9×109)⋅(7.52×10−18)⋅(1.6×10−19)(1.67×10−27)⋅(2.5×10−14)
Step 6: Calculate the Velocity
Calculating the numerator:
2⋅(9×109)⋅(7.52×10−18)⋅(1.6×10−19)=2.29×10−26
Calculating the denominator:
(1.67×10−27)⋅(2.5×10−14)=4.175×10−41
Now substituting these values back into the equation:
v=√2.29×10−264.175×10−41
Calculating the fraction:
2.29×10−264.175×10−41≈5.49×1014
Finally, taking the square root:
v≈√5.49×1014≈2.34×107 m/s
Final Answer
The speed at which a beam of protons has to be fired at a sheet of silver foil is approximately:
v≈2.34×107 m/s
- Atomic number of silver (Z) = 47
- Distance from the nucleus (r) = 2.5×10−14 m
- Mass of a proton (m) = 1.67×10−27 kg
- Charge of a proton (e) = 1.6×10−19 C
The total charge (Q) of the silver nucleus can be calculated using the formula:
Q=Z⋅e
Substituting the values:
Q=47⋅(1.6×10−19)=7.52×10−18 C
The kinetic energy (KE) of the protons must equal the electrostatic potential energy (PE) at the distance r:
KE=PE
12mv2=k⋅Q⋅er
Where k is Coulomb's constant, approximately 9×109N m2/C2.
Rearranging the equation to solve for v:
v2=2k⋅Q⋅em⋅r
v=√2k⋅Q⋅em⋅r
Substituting the known values into the equation:
v=
⎷2⋅(9×109)⋅(7.52×10−18)⋅(1.6×10−19)(1.67×10−27)⋅(2.5×10−14)
Calculating the numerator:
2⋅(9×109)⋅(7.52×10−18)⋅(1.6×10−19)=2.29×10−26
Calculating the denominator:
(1.67×10−27)⋅(2.5×10−14)=4.175×10−41
Now substituting these values back into the equation:
v=√2.29×10−264.175×10−41
Calculating the fraction:
2.29×10−264.175×10−41≈5.49×1014
Finally, taking the square root:
v≈√5.49×1014≈2.34×107 m/s
The speed at which a beam of protons has to be fired at a sheet of silver foil is approximately:
v≈2.34×107 m/s
Topper's Solved these Questions
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - Check point 12.1
14 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - Check point 12.2
16 Videos
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - JEE MAIN
62 Videos
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Video
Similar Questions
Calculate the speed with which wafer emerges from a hole in a tank at which the gauge pressure is 3×105N/m2 ?
View SolutionCalculate the wavelength (in nanometer) associated with a proton moving at 1.0×103ms−1 (Mass of proton =1.67×10−27kgandh=6.63×10−34Js)
View SolutionIn the Bohr model of hydrogen atom, the electron is treated as a particle going in a circle with the centre at the proton. The proton itself is assumed to be fixed in an inertial frame. The centripetal force is provided by the Coloumb attraction. In the ground state, the electron goes round the proton in a circle of radius 5.3×10−11m. Find the speed of the electron in the ground state. Mass of the electron =9.1×10−31kg and charge of the electron =1.6×10−19C.
View SolutionA monochromatic beam of bright of light of wavelength 5000Å is used in Young's double slit experiment. If one of the slits is covered by a transparent sheet of thikness 1.4×10−5m, having refractive index of its medium 1.25. Then the number of fringes shifted is
View SolutionRutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
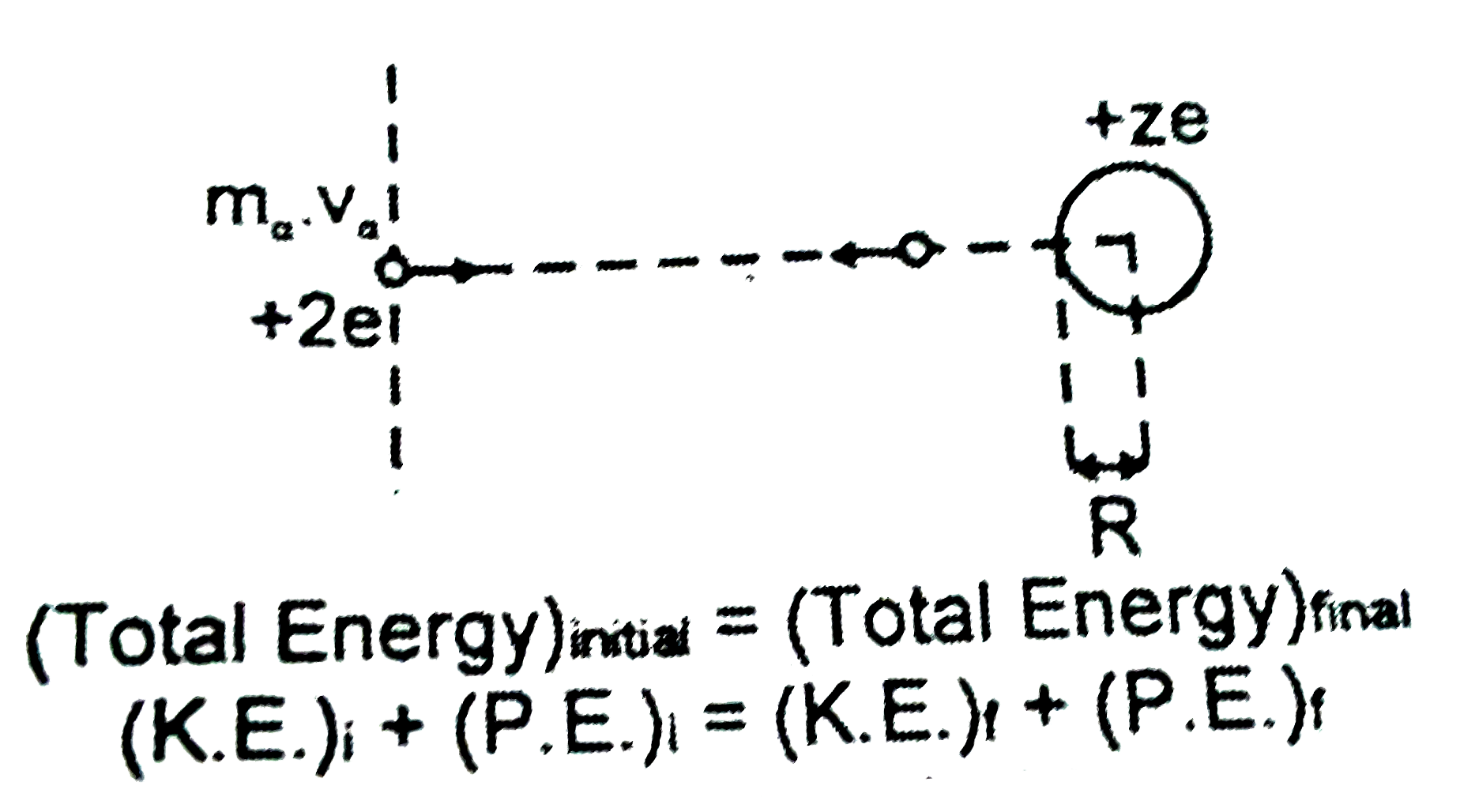
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
Radius of a particular nucleus is calculated by the projection of α-particle from infinity at a particular speed. Let this radius is the true radius . If the radius calculation for the same nucleus is made by another α-particle with half of the earlier speed, then the percentage error involved in the radius calculation is :
View SolutionAn accelration produces a narrow beam of protons, each having an initial speed of v0. The beam is directed towards an initially uncharges distant metal sphere of radius R and centered at point O. The initial path of the beam is parallel to the axis of the sphere at a distance of (R/2) from the axis, as indicated in the diagram.

The protons in the beam that collide with the sphere will cause it to becomes charged. The subsequentpotential field at the accelerator due to the sphere can be neglected. The angular momentum of a particle is defined in a similar way to the moment of a force. It is defined as the moment of its linear momentum, linear replacing the force. We may assume the angular momentum of a proton about point O to be conserved. Assume the mass of the proton as mP and the charge on it as e. Given that the potential of the sphere increases with time and eventually reaches a constant velue.
The total energy (E) of a proton in the beam travelling with seed v at a distance of r(r≥R) from point O. Assuming that the sphere has acquired an electrostatic charge Q is
View SolutionThe average separation between the proton and the electron in a hydrogen atom in ground state is 5.3xx10^-11m. a. Calculate the Coulomb force between tehm at this separation. b. When the atom goes into its first excited state the average separation between the roton and the electron increases to four times its value in the ground state.What is th Coulomb force in this state?
View SolutionSilver crystallises in a face centred cubic lattice with all the atoms at the lattice points. The length of the edge of the unit cell as determined by X-ray diffraction studies is found to be 4.077×10−8cm. The density of silver is 10.5g cm−3. Calculate the atomic mass of silver.
View SolutionA beam of specific kind of particles of velocity 2.1×107m/s is scattered by a gold (Z=79) nuclei. Find out specific charge (charge/mass) of this particle if the distance of closest approach is 2.5×10−14m.
View SolutionSuppose the exact charge neutrality does hold not in a world and the electron has a charge 1% less in magnitude than the proton. Calculate the Coulomb force acting between two blocks of iron each of mass 1 kg separated by a distance of 1m. Number of protons in an iron atom =26 and 58 kg of iron contains 6×1026 atoms.
View Solution
