Question

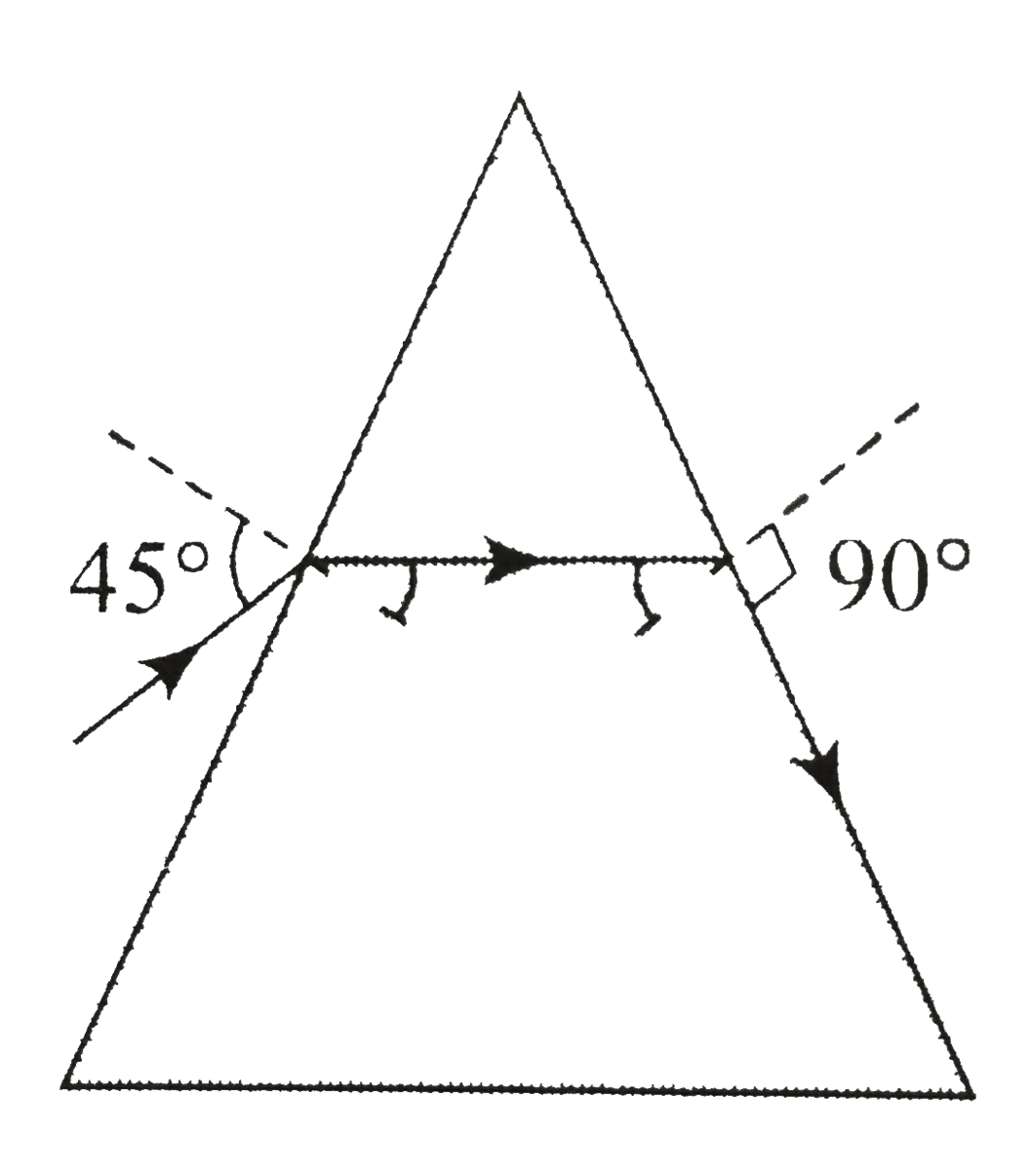
A ray of light is incident at small angle I on the surface of prism of small angle A and emerges normally from the oppsite surface. If the refractive index of the material of the prism is mu, the angle of incidence is nearly equal to

Options

A. `muA`
B. `(muA)/(2)`
C. `A//mu`
D. `A//2mu`

Answer

A

Explanation

To solve the problem, we need to determine the angle of incidence (I) of a ray of light incident on a prism with a small angle (A) and a refractive index (μ), given that the ray emerges normally from the opposite surface of the prism.

1. Understanding the Geometry of the Prism:
- The prism has an apex angle \( A \).
- A ray of light is incident at an angle \( I \) on one surface of the prism.
- The ray emerges normally from the opposite surface, which means the angle of emergence \( e = 0 \).

2. Using the Relation for Deviation:
- The angle of deviation \( \delta \) is related to the angle of incidence \( I \), angle of emergence \( e \), and the angle of the prism \( A \) by the formula:
\( \delta + A = I + e \)
- Since \( e = 0 \), we can simplify this to:
\( \delta + A = I \)

3. Finding the Angle of Deviation:
- The angle of deviation \( \delta \) for a prism can also be expressed in terms of the refractive index \( \mu \) and the angle of the prism \( A \):
\( \delta = A(\mu - 1) \)

4. Substituting the Deviation into the Relation:
- Now we substitute \( \delta \) into the deviation relation:
\( A(\mu - 1) + A = I \)
- This can be rearranged to:
\( I = A(\mu - 1) + A \)
- Simplifying this gives:
\( I = A\mu - A + A = A\mu \)

5. Conclusion:
- Therefore, the angle of incidence \( I \) is nearly equal to:
\( I \approx A\mu \)

Final Answer:
The angle of incidence \( I \) is nearly equal to \( A\mu \).