The energy of gamma (γ) ray photon is Eγ and that of an X-rays photon is EX. If the visible light photon has an energy of Ev, then we can say that
EX>Eγ>Ev
Eγ>Ev>EX
Eγ>EX>Ev
EX>Ev>Eγ
The correct Answer is:C
To solve the problem, we need to compare the energies of gamma ray photons, X-ray photons, and visible light photons. The energy of a photon is given by the formula:
E=hν
where:
- E is the energy of the photon,
- h is Planck's constant,
- ν (nu) is the frequency of the photon.
1. Understand the relationship between energy and frequency:
The energy of a photon is directly proportional to its frequency. This means that as the frequency increases, the energy of the photon also increases.
E∝ν
2. Identify the order of frequencies for different types of electromagnetic waves:
We know the order of electromagnetic waves based on their frequencies:
- Gamma rays have the highest frequency.
- X-rays have a lower frequency than gamma rays but higher than visible light.
- Visible light has the lowest frequency among the three.
Thus, we can express this relationship as:
νγ>νX>νv
3. Relate frequencies to energies:
Since energy is directly proportional to frequency, we can relate the energies of the photons:
- For gamma rays:
Eγ∝νγ
- For X-rays:
EX∝νX
- For visible light:
Ev∝νv
4. Establish the inequalities for energies:
From the frequency relationships, we can conclude:
Eγ>EX>Ev
5. Final conclusion:
Therefore, we can say that:
Eγ>EX>Ev
Final Answer:
The correct relationship is:
Eγ>EX>Ev
- E is the energy of the photon,
- h is Planck's constant,
- ν (nu) is the frequency of the photon.
The energy of a photon is directly proportional to its frequency. This means that as the frequency increases, the energy of the photon also increases.
We know the order of electromagnetic waves based on their frequencies:
- Gamma rays have the highest frequency.
- X-rays have a lower frequency than gamma rays but higher than visible light.
- Visible light has the lowest frequency among the three.
νγ>νX>νv
Since energy is directly proportional to frequency, we can relate the energies of the photons:
- For gamma rays:
Eγ∝νγ
- For X-rays:
EX∝νX
- For visible light:
Ev∝νv
From the frequency relationships, we can conclude:
Eγ>EX>Ev
Therefore, we can say that:
Eγ>EX>Ev
The correct relationship is:
Eγ>EX>Ev
Topper's Solved these Questions
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Match the columns
2 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
Similar Questions
Photon of which light has maximum energy :
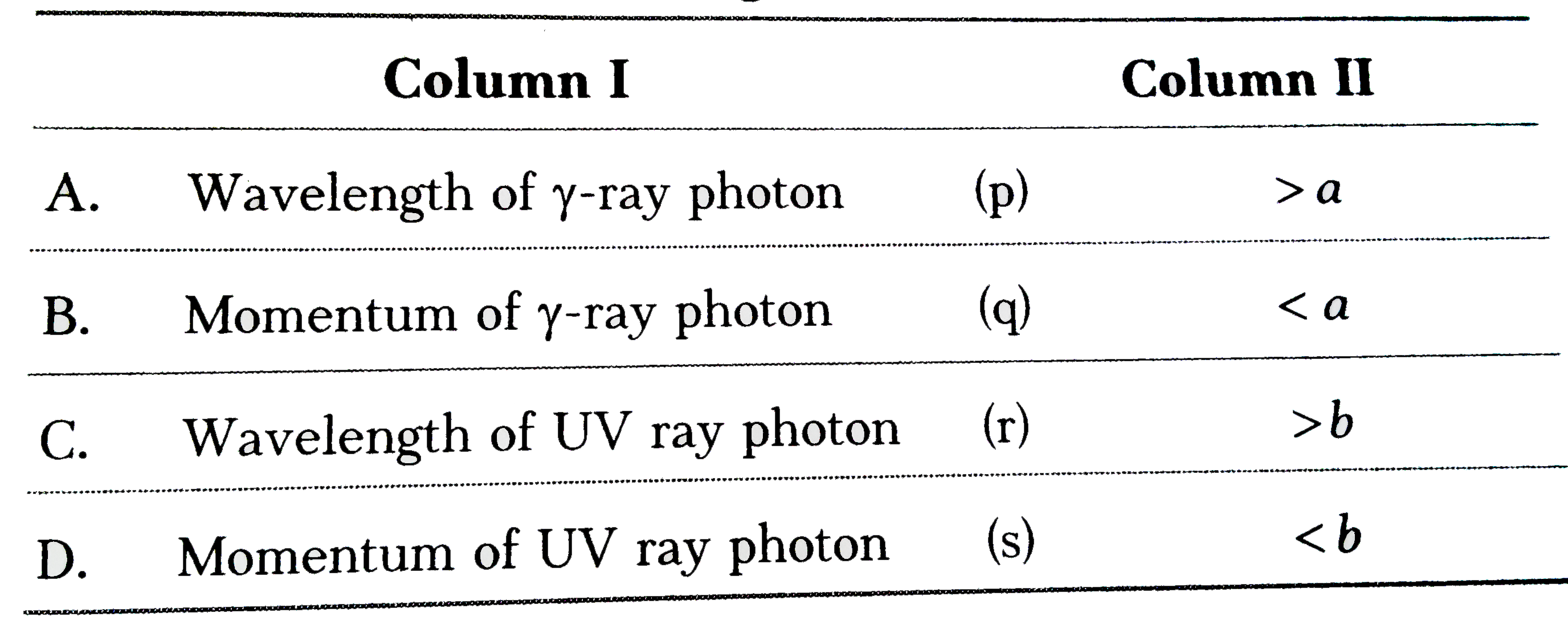
View SolutionWavelength of X-ray photon is a and momentum of X-ray photon is b.
Now, match the following two columns
View SolutionThe energy of an x-ray photon is 4 kev. Its frequency is nearly
View SolutionThe de-Broglie wavelength of an electron is the same as that of a 50 keV X-ray photon. The ratio of the energy of the photon to the kinetic energy of the electron is ( the energy equivalent of electron mass of 0.5 MeV)
View SolutionThe momentum of an x-ray photon with λ=0.5Å is
View SolutionThe wavelenths γof a photon and the de-Broglie wavelength of an electron have the same value.Find the ratio of energy of photon to the kinetic energy of electron in terms of mass m, speed of light and planck constatn
View SolutionThe ratio of wavelength of a photon and that of an electron of same energy E will be
View SolutionThe energy of a photon is equal to the kinetic energy of a
proton. The energy of the photon is E. Let λ1be the de-Broglie wavelength of the
proton and λ2 be the wavelength of the photon. The ratio λ1λ2 is proportional to
(a)E0 (b) E1/2 (c ) E−1 (d)E−2
View SolutionThe frequency order of for γ-rays (b) X-rays (a) UV-rays (c ):
View SolutionA high-energy gamma-photon may turn into an electron-positron pair in the field of heavy nuclei. What is the minimum energy of the gamma-photon?
View Solution
