Assertion: At frequency greater than resonance frequency circuit is inductive in nature.
Reason: XL∝ω
If both Assertion and Reason are true and Reason is the correct explanation of Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true but Reason is false.
If Assertion is false but Reason is true.
The correct Answer is:A
To solve the question, we need to evaluate the assertion and the reason provided:
Assertion: At frequency greater than resonance frequency, the circuit is inductive in nature.
Reason: XL∝ω
1. Understanding Resonance Frequency:
- The resonance frequency (f0) in an RLC circuit is given by the formula:
f0=12π√LC
- At this frequency, the inductive reactance (XL) and capacitive reactance (XC) are equal, leading to a purely resistive impedance.
2. Behavior of Reactances:
- Inductive reactance is given by:
XL=ωL
- Capacitive reactance is given by:
XC=1ωC
- Here, ω is the angular frequency, which is related to the frequency f by ω=2πf.
3. Analyzing Frequencies Greater than Resonance:
- When the frequency is increased beyond the resonance frequency (f>f0), the following occurs:
- XL increases because it is directly proportional to ω.
- XC decreases because it is inversely proportional to ω.
4. Conclusion on Circuit Nature:
- Since XL increases and XC decreases, the inductive reactance becomes greater than the capacitive reactance:
XL>XC
- This means the circuit behaves inductively, as the net reactance is positive, leading to an overall inductive nature.
5. Evaluating the Assertion and Reason:
- The assertion is true: at frequencies greater than the resonance frequency, the circuit is indeed inductive.
- The reason is also true: XL is proportional to ω, which explains why the circuit becomes inductive as frequency increases.
6. Final Conclusion:
- Both the assertion and reason are correct, and the reason correctly explains the assertion.
Final Answer:
Both the assertion and the reason are true, and the reason is the correct explanation for the assertion.
---
- The resonance frequency (f0) in an RLC circuit is given by the formula:
f0=12π√LC
- At this frequency, the inductive reactance (XL) and capacitive reactance (XC) are equal, leading to a purely resistive impedance.
- Inductive reactance is given by:
XL=ωL
- Capacitive reactance is given by:
XC=1ωC
- Here, ω is the angular frequency, which is related to the frequency f by ω=2πf.
- When the frequency is increased beyond the resonance frequency (f>f0), the following occurs:
- XL increases because it is directly proportional to ω.
- XC decreases because it is inversely proportional to ω.
- Since XL increases and XC decreases, the inductive reactance becomes greater than the capacitive reactance:
XL>XC
- This means the circuit behaves inductively, as the net reactance is positive, leading to an overall inductive nature.
- The assertion is true: at frequencies greater than the resonance frequency, the circuit is indeed inductive.
- The reason is also true: XL is proportional to ω, which explains why the circuit becomes inductive as frequency increases.
- Both the assertion and reason are correct, and the reason correctly explains the assertion.
Both the assertion and the reason are true, and the reason is the correct explanation for the assertion.
Similar Questions
Knowledge Check
To reduce the resonant frequency in an LCR series circuit with a generator
Athe generator frequency should be reduced.Banother capacitor should be added in parallel to the first.Cthe iron core of the inductor should be removed.Ddielectric in the capacitor should be removed.At resonance frequency the impedance in series LCR circuit is
AmaximumBminimumCzeroDinfinityAt resonant frequency the current amplitude in series LCR circuit is
AmaximumBminimumCzeroDinfinity
The natural frequency of the circuit shown in the figure is
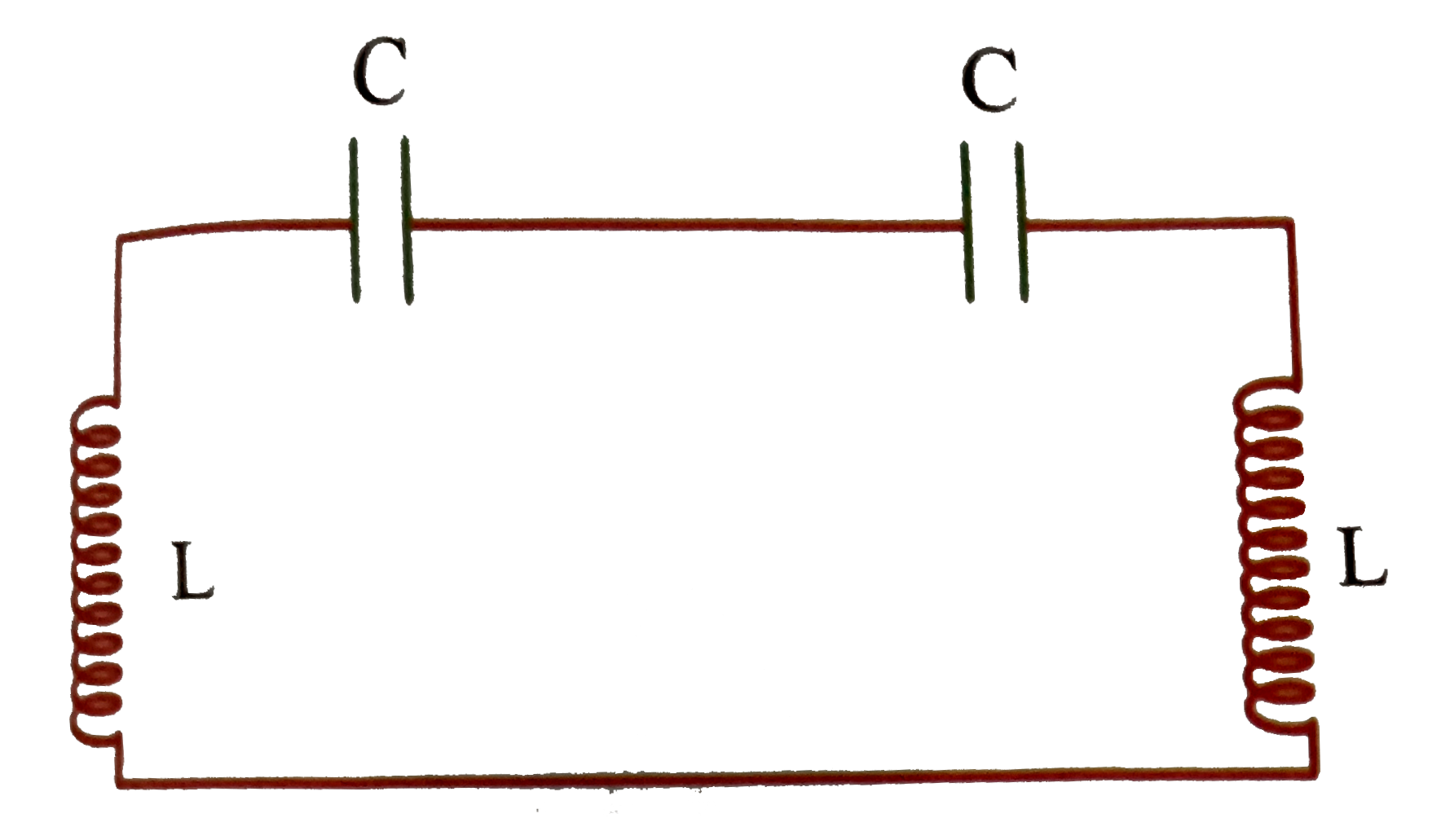
View SolutionThe natural frequency of the circuit shows in Fig. is

View SolutionAssertion : In series L−C−R circuit, voltage will lead the current function for frequency greater than the resonance frequency.
Reason : At resonance frequency, phase difference between current function and voltage function is zero.
View SolutionIf frequency of R- L circuit is f then impedence will be
View SolutionIn a series LCR circuit, the frequency of the source is more than resonance frequency. The current in the circuit leads the voltage in phases(True/false).
View Solution
