In a certain circuit current changes with time according to i=2√t RMS value of current between t=2s to t=4s will be
3A
3√3A
2√3
√3A
The correct Answer is:C
To find the RMS (Root Mean Square) value of the current i=2√t between t=2 seconds and t=4 seconds, we can follow these steps:
1. Understanding RMS Value:
The RMS value of a current i(t) over a time interval (a,b) is given by:
IRMS=√1b−a∫bai(t)2dt
2. Substituting the Current Function:
Here, i(t)=2√t. Therefore, we need to calculate i(t)2:
i(t)2=(2√t)2=4t
3. Setting Up the Integral:
We will now set up the integral for the interval from t=2 to t=4:
IRMS=√14−2∫424tdt
4. Calculating the Integral:
We calculate the integral ∫424tdt:
∫4tdt=2t2+C
Evaluating from 2 to 4:
(2t2)42=2(42)−2(22)=2(16)−2(4)=32−8=24
5. Substituting Back into the RMS Formula:
Now substitute the result of the integral back into the RMS formula:
IRMS=√12⋅24=√12=2√3
6. Final Answer:
Therefore, the RMS value of the current between t=2 seconds and t=4 seconds is:
IRMS=2√3A
The RMS value of a current i(t) over a time interval (a,b) is given by:
IRMS=√1b−a∫bai(t)2dt
Here, i(t)=2√t. Therefore, we need to calculate i(t)2:
i(t)2=(2√t)2=4t
We will now set up the integral for the interval from t=2 to t=4:
IRMS=√14−2∫424tdt
We calculate the integral ∫424tdt:
∫4tdt=2t2+C
Evaluating from 2 to 4:
(2t2)42=2(42)−2(22)=2(16)−2(4)=32−8=24
Now substitute the result of the integral back into the RMS formula:
IRMS=√12⋅24=√12=2√3
Therefore, the RMS value of the current between t=2 seconds and t=4 seconds is:
IRMS=2√3A
Similar Questions
Knowledge Check
In an inductor of self-inductance L=2 mH, current changes with time according to relation i=t2e−t. At what time emf is zero ?
A4sB3sC2sD1sIn an inductor of self-inductance L=2 mH, current changes with time according to relation i=t2e−t. At what time emf is zero ?
A4sB3sC2sD1sThe magnetic flux (ϕ) in a closed circuit of resistance 20Ω varies with time (t) according to the equation ϕ=7t2−4t where ϕ is in weber and t is in seconds. The magnitude of the induced current at t =0.25s is
A25mAB0.025mAC47 mAD175 mA
The current I through a conductor varies with time t as shown in figure. The average electric current during t=0 to t=10 s will be
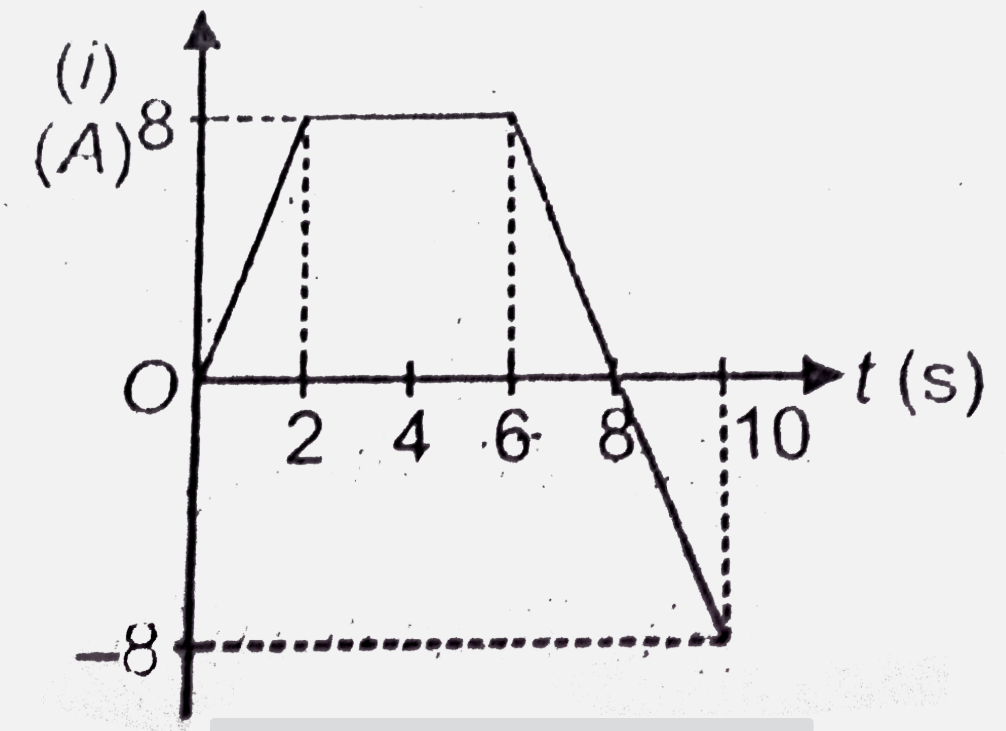
View SolutionThe current in a certain circuit varies with time as shown in figure. Find the average current and the rms current in terms of I0
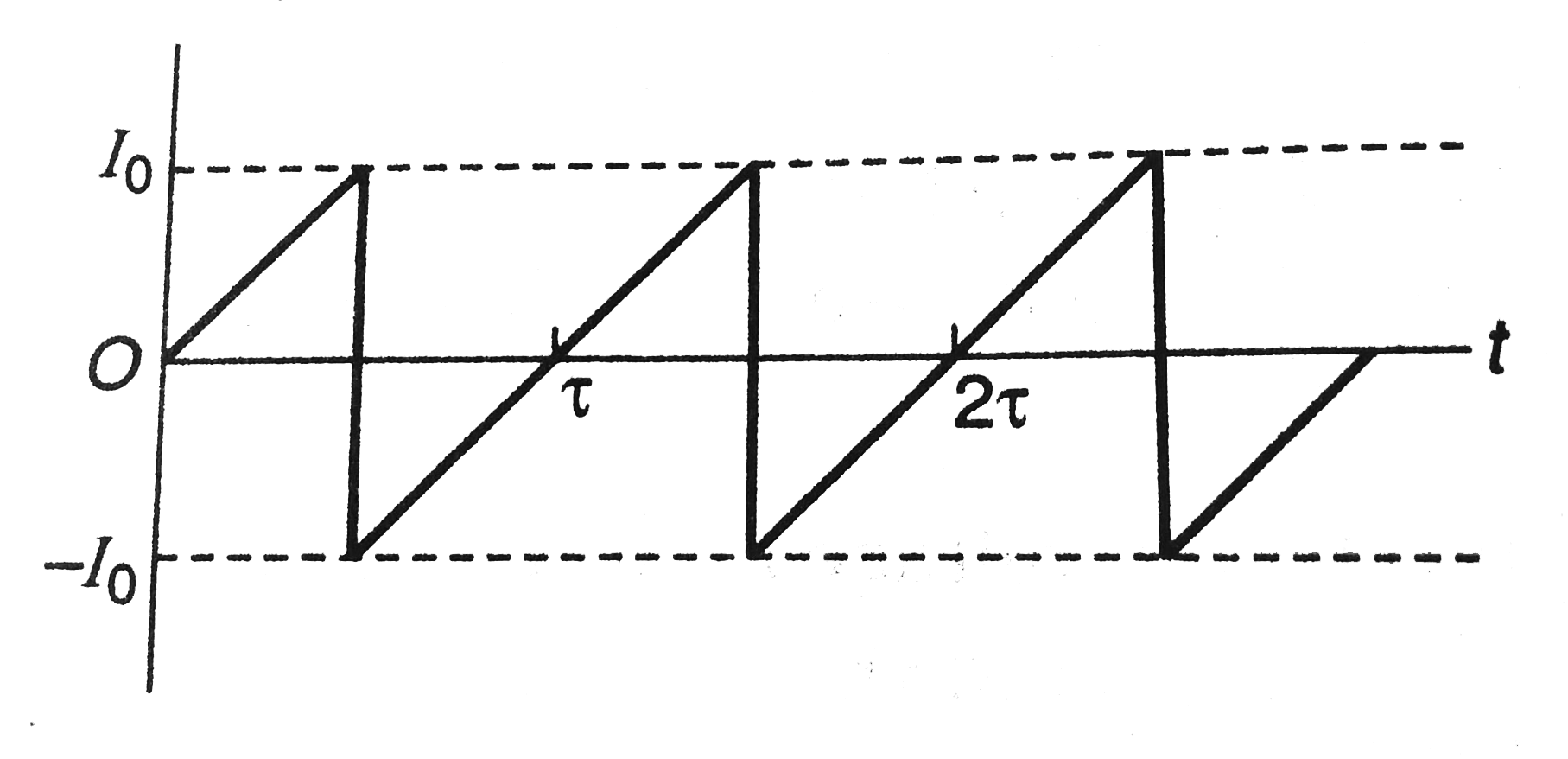 .
.
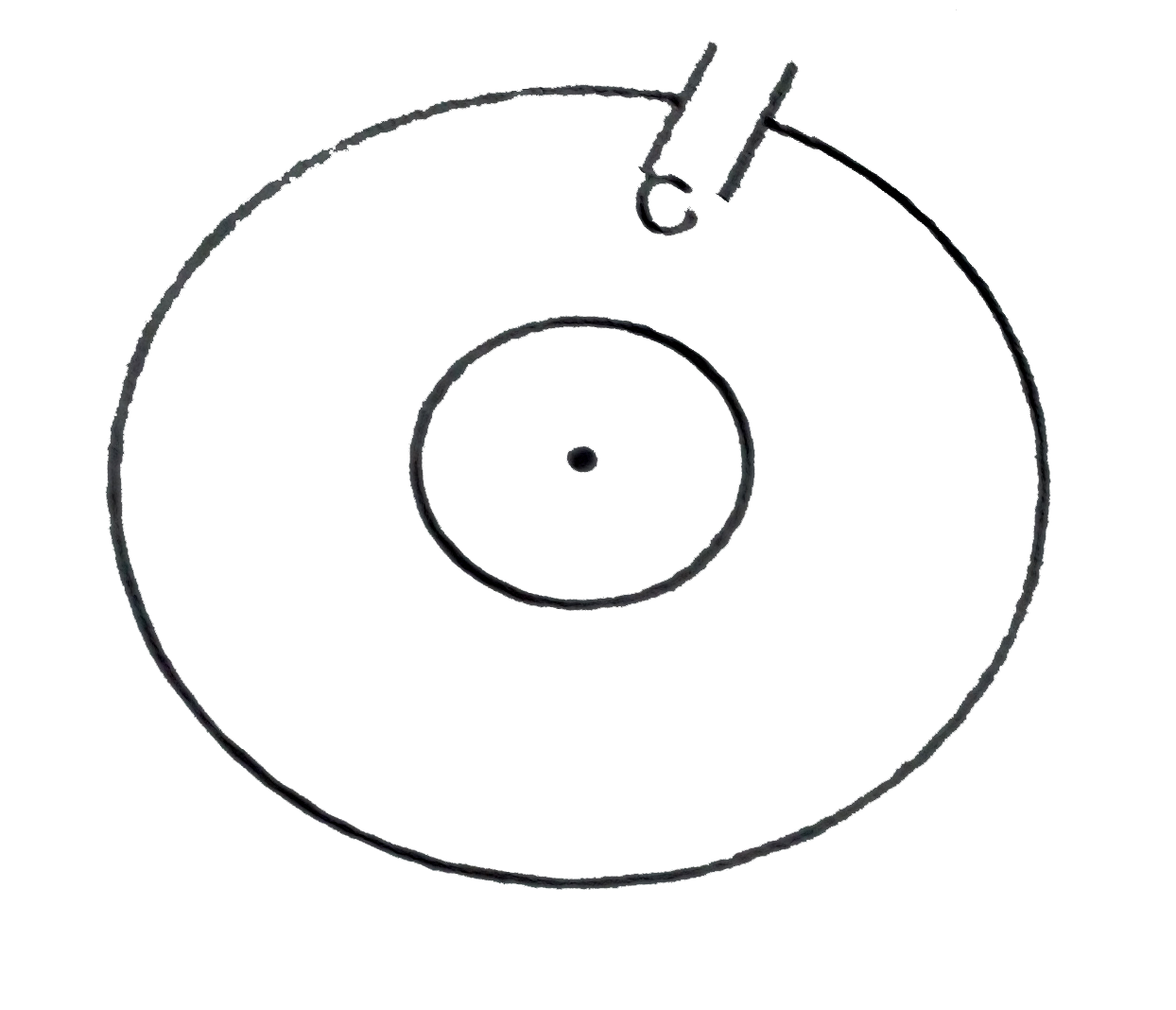
View SolutionIf the current in the inner loop changes according to i=2t2 then, find the current in the capacitor as a function of time.
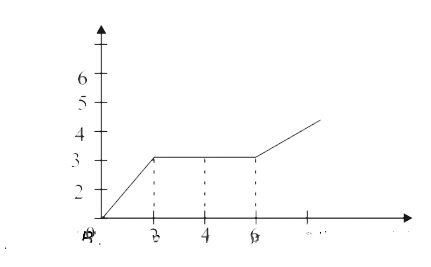
View SolutionThe charge on a capacitor plate in a circuit, as a function of time, is shown in the figure. What is the value of current at t=4s?
View SolutionFlux ϕ (in weber) in a closed circuit of resistance5 omega varies with time (in second) according to equation ϕ=3t2−5t+2. The magnitude of average induced current between0 to 2s is
View Solution
