The reactance of a 25μF capacitor at the AC frequency of 4000Hz is
5πΩ
√5πΩ
10Ω
√10Ω
The correct Answer is:A
To find the reactance of a capacitor, we can use the formula for capacitive reactance XC:
XC=1ωC
where:
- ω is the angular frequency in radians per second,
- C is the capacitance in farads.
Step 1: Calculate Angular Frequency ω
The angular frequency ω is given by the formula:
ω=2πf
where f is the frequency in hertz.
Given:
- f=4000Hz
Substituting the value of f:
ω=2π×4000
Step 2: Calculate Capacitance C
The capacitance C is given as:
C=25μF=25×10−6F
Step 3: Substitute ω and C into the Reactance Formula
Now we can substitute ω and C into the formula for XC:
XC=1ωC=1(2π×4000)×(25×10−6)
Step 4: Calculate XC
Calculating ω:
ω=2π×4000≈25132.74rad/s
Now substituting this value into the reactance formula:
XC=125132.74×25×10−6≈10.628318≈1.59155Ω
Step 5: Final Calculation
To simplify further, we can express this in terms of 5π:
XC≈5πΩ
Conclusion
Thus, the reactance of the 25μF capacitor at an AC frequency of 4000Hz is approximately:
XC≈5πΩ
- ω is the angular frequency in radians per second,
- C is the capacitance in farads.
- f=4000Hz
Similar Questions
(a) What is the reactance of a 2.00H inductor at a frequency of 50.0Hz?
(b) What is the inductance of an inductor whose reactance is 2.00Ω at 50.0Hz?
(c) What is the reactance of a 2.00μF capacitor at a frequency of 50.0Hz?
(d) What is the capacitance of a capacitor whose reactance is 2.00Ω at 50.0Hz?
View SolutionThe energy stored in 4μF capacitors is
View SolutionThe figure shows the graphical variation of the reactance of a capacitor with frequency of ac source. (a) find the capacitance of the capacitor (b)an ideal inductor has the same reactance at 100 Hz frequency as the capacitor has the same frequency find the value of the inductor. (c) draw the graph showing the variation of the reactance of this inductor with frequency

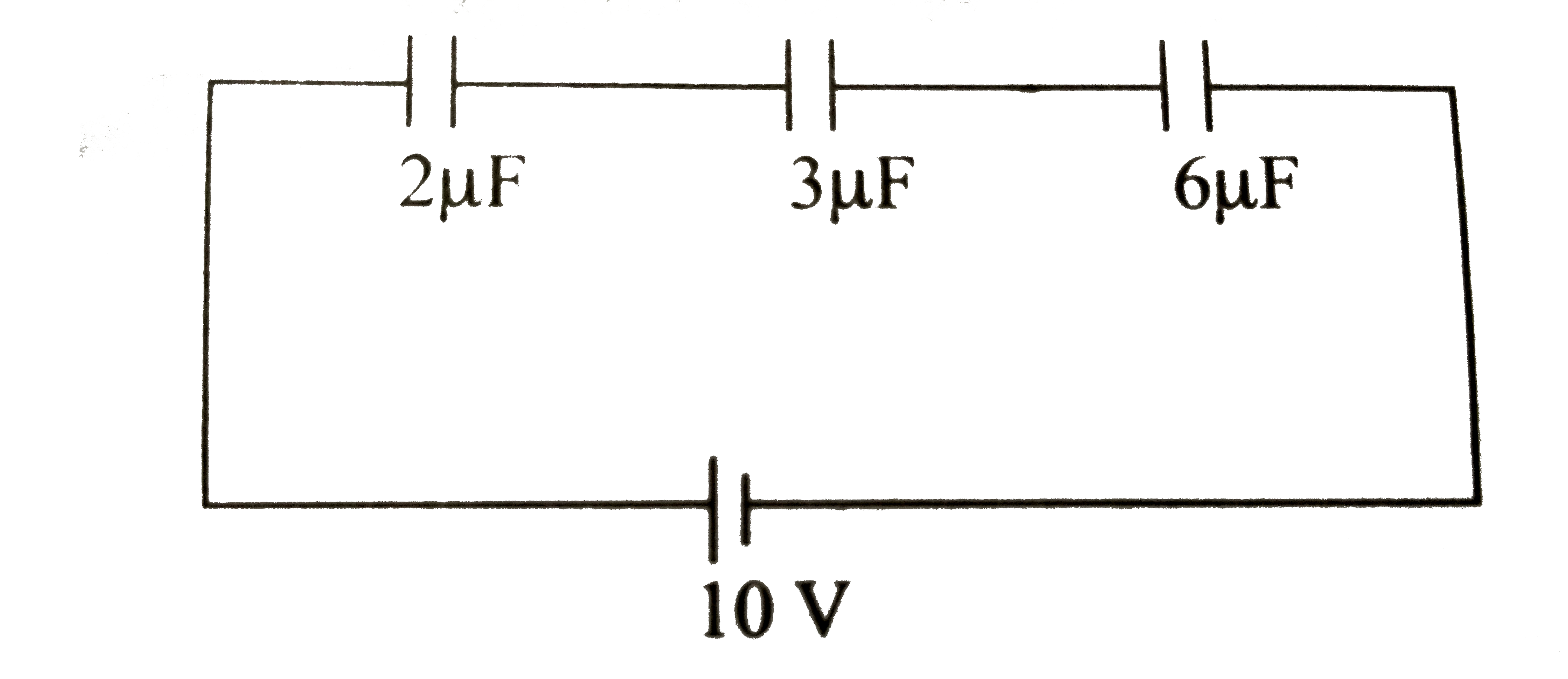
View SolutionThe charge on 3μF capacitor shown in the figure is
View SolutionThe reactance of a capacitor of capacitance C is X. If both the frequency and capacitance be doubled, then new reactance will be
(a) X (b) 2X (c) 4X (d) X4
View SolutionThe frequency for which a 5.0 muF capacitor has a reactance of 1000Ω is given by
View SolutionThe frequency for which a 5μF capacitor has a reactance of 11000Ωis given by
A.100π MHz B.1000π MHz C.11000Hz D.1000 Hz
View SolutionAn inductive coil has a reactance of 100Ω. When an AC signal of frequency 1000 Hz is applied to the coil, the voltage leads the current by 45∘. What is the inductance of the coil?
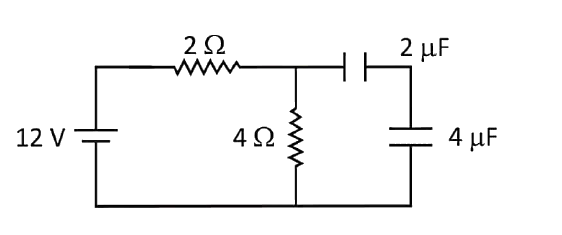
View SolutionThe charge on the 4μF capacitor in the steady state is
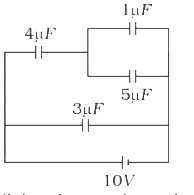
View SolutionThe charge on the 4μF capacitor at steady state is (in μC)
View Solution
