A capacitor of capacitance 250 pF is connected in parallel with a choke coil having inductance of 1.6×10−2H and resistance 20Ω. Calculate
(a) the resonance frequency and
(b) the circuit impedance at resonance.
To solve the given problem, we will follow these steps:
Step 1: Calculate the Resonance Frequency
The formula for the resonance frequency f in a parallel LC circuit is given by:
f=12π√LC√1−R2L2
Where:
- L is the inductance,
- C is the capacitance,
- R is the resistance.
Given:
- L=1.6×10−2H
- C=250pF=250×10−12F
- R=20Ω
Substituting the values into the formula:
1. Calculate LC:
LC=(1.6×10−2)×(250×10−12)=4×10−14H⋅F
2. Calculate R2 and L2:
R2=202=400Ω2
L2=(1.6×10−2)2=2.56×10−4H2
3. Calculate R2L2:
R2L2=4002.56×10−4=1562500
4. Now, substitute into the frequency formula:
f=12π√4×10−14√1−1562500
Since 1−1562500 is negative, it indicates that resonance cannot occur under these conditions. However, for the sake of calculation, we will proceed with the assumption that we are looking for the frequency without the damping factor.
5. Calculate f:
f=12π√4×10−14
f≈12π×2×10−7≈11.25664×10−6≈796178.34Hz≈7.96×105Hz
Step 2: Calculate the Circuit Impedance at Resonance
The impedance Z at resonance in a parallel LC circuit is given by:
Z=LCR
Substituting the values:
1. Calculate LC:
LC=1.6×10−2250×10−12=1.6×10−22.5×10−10=64000Ω
2. Now, substitute into the impedance formula:
Z=64000×20=1280000Ω=1.28×106Ω
Final Answers:
(a) The resonance frequency f≈7.96×105Hz
(b) The circuit impedance at resonance Z≈1.28×106Ω
---
- L is the inductance,
- C is the capacitance,
- R is the resistance.
- L=1.6×10−2H
- C=250pF=250×10−12F
- R=20Ω
LC=(1.6×10−2)×(250×10−12)=4×10−14H⋅F
R2=202=400Ω2
L2=(1.6×10−2)2=2.56×10−4H2
R2L2=4002.56×10−4=1562500
f=12π√4×10−14√1−1562500
f=12π√4×10−14
f≈12π×2×10−7≈11.25664×10−6≈796178.34Hz≈7.96×105Hz
LC=1.6×10−2250×10−12=1.6×10−22.5×10−10=64000Ω
Z=64000×20=1280000Ω=1.28×106Ω
(a) The resonance frequency f≈7.96×105Hz
Similar Questions
A condenser of 250μF is connected in parallel to a coil of inductance 0.16mH while its effective resistance is 20Ω. Determine the resonant frequency
View SolutionA 50 Hz AC signal is applied in a circuit of inductance of (1/π)H and resistance 2100Ω. The impedance offered by the circuit is
View SolutionA capacitor of capacitance 1μF is connected in parallel with a resistance 10Ω and the combination is connected across the terminals of a battery of EMF 50 V and internal resistance 1Ω. The potential difference across the capacitor at steady state is (in Volt)
View SolutionA coil having self-inductance of 0.7 H and resistance of 165Ω is connected to an a.c. source of 275 V, 50 Hz. If π=227 Calculate :
Impedance of the coil
View SolutionA coil having a resistance of 5 Ω and an inductance of 0.02H is arranged in parallel with another coil having a resistance of 1Ω and an inductance of 0.08H. Calculate the power absorbed when a voltage of 100V at 50Hz is applied.
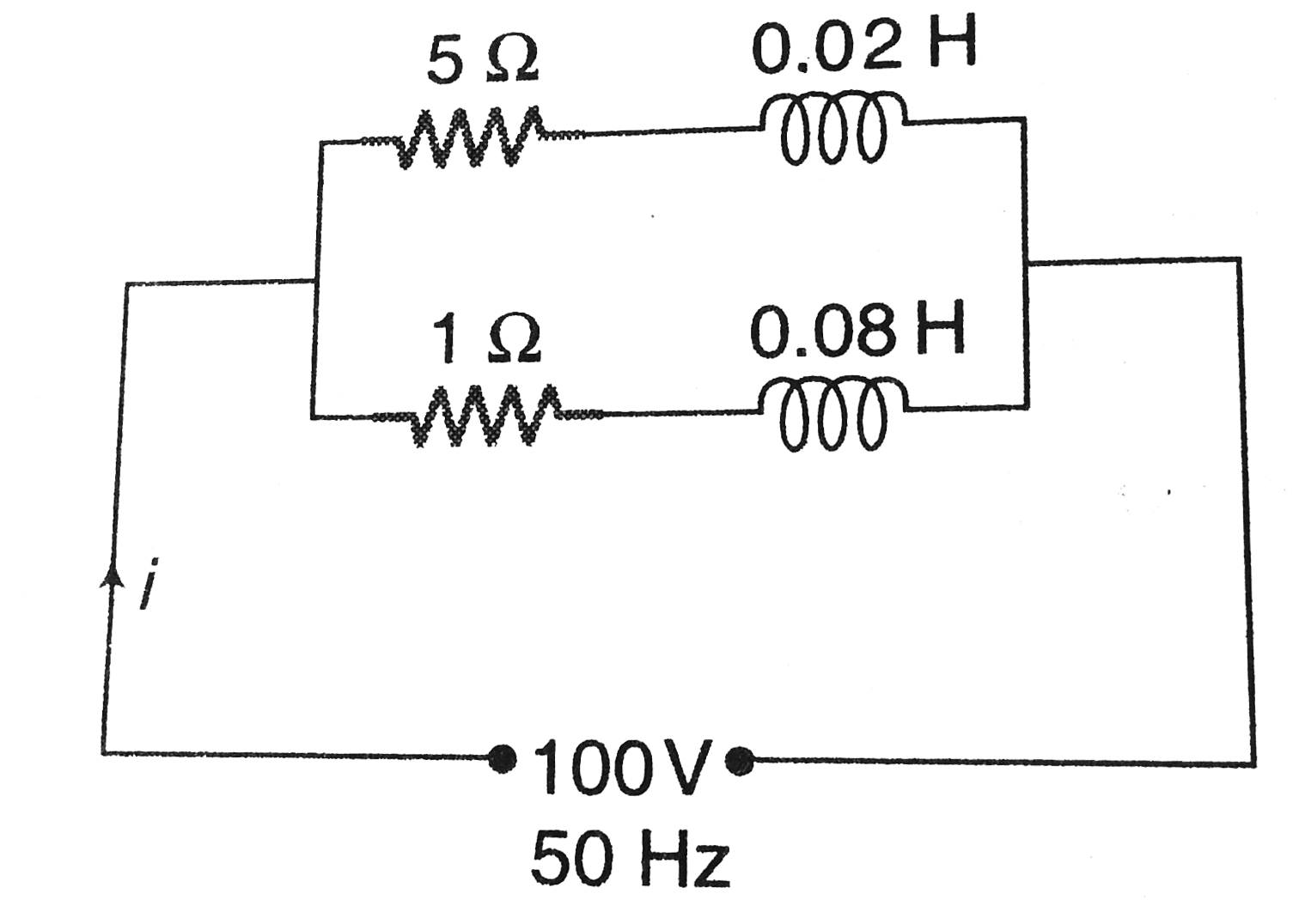 .
.
View SolutionA capacitor of capacitance 1μF is charged to a potential of 1V, it is connected in parallel to an inductor of inductance 10−3H. The maximum current that will flow in the circuit has the value
View SolutionA coil of inductance 0.20H is connected in series with a switch and a cell of emf 1.6V. The total resistance of the circuit is 4.0Ω. What is the initial rate of growth of the current when the switch is closed?
View Solutiontwo heading coils of resistances 10Ωand20Ω are connected in parallel and connected to a battery of emf 12 V and internal resistance 1Ω Thele power consumed by the n are in the ratio
View SolutionA coil having self-inductance of 0.7 H and resistance of 165Ω is connected to an a.c. source of 275 V, 50 Hz. If π=227 Calculate :
Reactance of the coil
View SolutionThe quality factor of LCR circuit having resistance ( R ) and inductance ( L ) at resonance frequency (ω) is given by
View Solution
