The space within a current carrying solenoid is filled with magneisum having magnetic suscepitbiliy. x=Mg=1.2×10−5. What will be the percentage increase in magnetic field?
To solve the problem of finding the percentage increase in the magnetic field when the space within a current-carrying solenoid is filled with magnesium, we can follow these steps:
Step 1: Understand the Initial Magnetic Field
The initial magnetic field B0 in a solenoid without any material inside is given by:
B0=μ0nI
where μ0 is the permeability of free space, n is the number of turns per unit length of the solenoid, and I is the current flowing through the solenoid.
Step 2: Determine the Magnetic Field with the Material
When the space inside the solenoid is filled with a material (in this case, magnesium), the magnetic field B is modified by the magnetic susceptibility χ of the material:
B=B0(1+χ)
Given that the magnetic susceptibility χ for magnesium is 1.2×10−5.
Step 3: Calculate the Change in Magnetic Field
The change in magnetic field ΔB can be calculated as:
ΔB=B−B0=B0(1+χ)−B0=B0χ
Step 4: Calculate the Percentage Increase in Magnetic Field
The percentage increase in the magnetic field can be calculated using the formula:
Percentage Increase=ΔBB0×100
Substituting ΔB:
Percentage Increase=B0χB0×100=χ×100
Step 5: Substitute the Value of χ
Now, substituting the value of χ:
Percentage Increase=1.2×10−5×100=1.2×10−3%
Final Answer
Thus, the percentage increase in the magnetic field is:
Percentage Increase=1.2×10−3%
---
The initial magnetic field B0 in a solenoid without any material inside is given by:
B0=μ0nI
where μ0 is the permeability of free space, n is the number of turns per unit length of the solenoid, and I is the current flowing through the solenoid.
When the space inside the solenoid is filled with a material (in this case, magnesium), the magnetic field B is modified by the magnetic susceptibility χ of the material:
B=B0(1+χ)
Given that the magnetic susceptibility χ for magnesium is 1.2×10−5.
The change in magnetic field ΔB can be calculated as:
ΔB=B−B0=B0(1+χ)−B0=B0χ
The percentage increase in the magnetic field can be calculated using the formula:
Percentage Increase=ΔBB0×100
Substituting ΔB:
Percentage Increase=B0χB0×100=χ×100
Now, substituting the value of χ:
Percentage Increase=1.2×10−5×100=1.2×10−3%
Thus, the percentage increase in the magnetic field is:
Percentage Increase=1.2×10−3%
Topper's Solved these Questions
- MAGNETISM AND MATTERBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - 5.1
15 Videos
- MAGNETISM AND MATTERBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - 5.2
15 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Videos
- MODERN PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICSEXERCISE - Integer Type Questions
17 Videos
Similar Questions
Knowledge Check
The percentage increase in magnetic field B when the space within a current carrying toroid is filled with aluminum (χ=2.1×10−5) is
A0.002B2×10−3%C2×10−2%D2×10−4%
A straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?
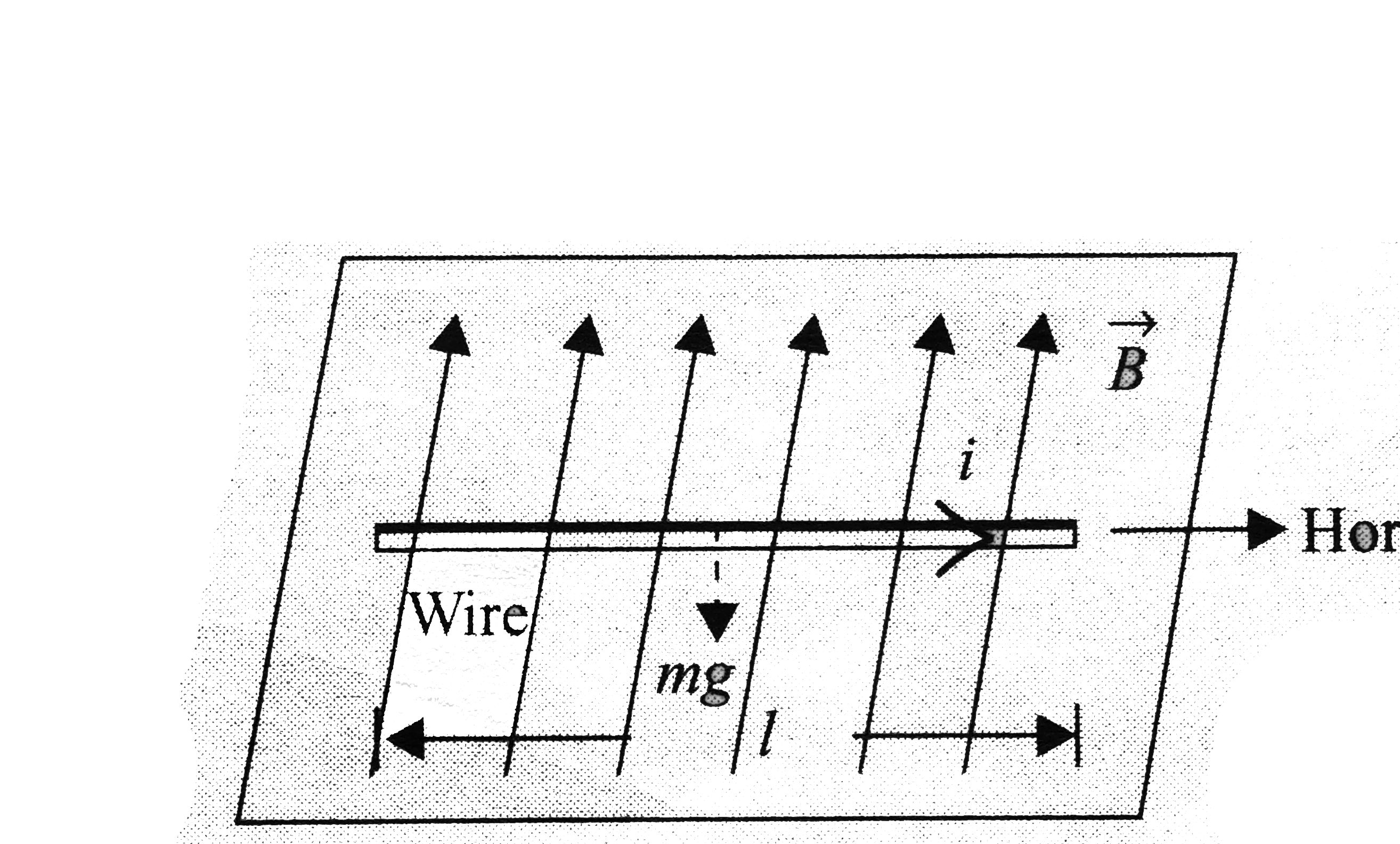
View SolutionA straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?

View SolutionA straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?

View SolutionThe magnitude of earth magnetic field at magnetic equator is 2.8×10−5T . Find magnitude of earth's magnetic field near geomagnetic poles .
View SolutionAt the centre of a current - carrying circular coil of radius 5 cm, magnetic field due to earth is 0.5×10−5Wb m−2. The current flowing through the coil, so that it equals the Earth's magnetic field, is
View SolutionThe magnetic field at a point near the centre but outside a current carrying solenoid is zero. Explain why?
View SolutionA bar magnet with magnetic moment 2.5×103JT−2 is rotating in horizontal plane in the space containing magnetic induction B=4×105T. The work done in rotating the magnet slowly from a direction parallel to the field to a direction 45∘ from the field, is (in joule).
View Solution
