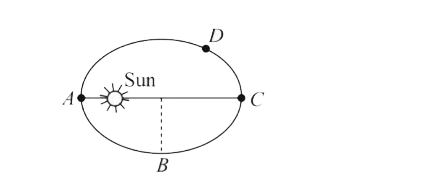
A planet revolves around the sun in an elliptical orbit. The linear speed of the planet will be maximum at:

Video Solution

Text Solution

Verified by Experts

Using the relation, $H = B_{e} cos θ,$ we get the magnitude of B given by
$B_{e} = \frac{H}{cos θ} = \frac{0.16}{{cos 60}^{\circ}} = \frac{0.16}{\frac{1}{2}}$
$= 0.16 \times 2 = 0.32 G$
$or = 0.32 \times 10^{- 4} T [∴ 1 G = 10^{- 4} T]$
Direction of B: The earth's field lies in a vertical plane $12^{\circ}$ west of geographic meridian at an angle of $60^{\circ}$ above the horizontal line.

|

Updated on:21/07/2023

Class 12 PHYSICS MAGNETISM AND MATTER

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A planet revolves in elliptical orbit around the sun. (see figure). The linear speed of the planet will be maximum at

AA
BB
CC
DD
Question 2 - Select One
A planet revolves around the sun in an elliptical orbit. If $v_{p}$ and $v_{a}$ are the velocities of the planet at the perigee and apogee respectively, then the eccentricity of the elliptical orbit is given by :
A $\frac{v_{p}}{v_{a}}$
B $\frac{v_{a} - v_{p}}{v_{a} + v_{p}}$
C $\frac{v_{p} + v_{a}}{v_{p} - v_{a}}$
D $\frac{v_{p} - v_{a}}{v_{p} + v_{a}}$

DC PANDEY ENGLISH-MAGNETISM AND MATTER-Medical gallery

Home
Ask Doubt
Profile