The horizontal and veritical components of eaths's field at a place ar 0.22 gauss of 0.38 gauss, respectively. Calculate the angle of dip and resultant intensity of earth's field.
To solve the problem, we need to find the angle of dip and the resultant intensity of Earth's magnetic field using the given horizontal and vertical components of the magnetic field.
1. Identify the Given Values:
- Horizontal component of Earth's magnetic field, BH=0.22 Gauss
- Vertical component of Earth's magnetic field, BV=0.38 Gauss
2. Calculate the Angle of Dip (φ):
- The angle of dip can be calculated using the formula:
tan(ϕ)=BVBH
- Substituting the values:
tan(ϕ)=0.380.22
- Simplifying the fraction:
tan(ϕ)=0.380.22≈1.727
- Now, calculate the angle φ:
ϕ=tan−1(1.727)
- Using a calculator:
ϕ≈59.56∘
3. Calculate the Resultant Intensity of Earth's Magnetic Field (B_net):
- The resultant intensity can be calculated using the formula:
Bnet=√B2V+B2H
- Substituting the values:
Bnet=√(0.38)2+(0.22)2
- Calculating the squares:
Bnet=√0.1444+0.0484=√0.1928
- Taking the square root:
Bnet≈0.439 Gauss
Final Answers:
- Angle of dip (φ) ≈ 59.56°
- Resultant intensity of Earth's magnetic field (B_net) ≈ 0.439 Gauss
- Horizontal component of Earth's magnetic field, BH=0.22 Gauss
- Vertical component of Earth's magnetic field, BV=0.38 Gauss
- The angle of dip can be calculated using the formula:
tan(ϕ)=BVBH
- Substituting the values:
tan(ϕ)=0.380.22
- Simplifying the fraction:
tan(ϕ)=0.380.22≈1.727
- Now, calculate the angle φ:
ϕ=tan−1(1.727)
- Using a calculator:
ϕ≈59.56∘
- The resultant intensity can be calculated using the formula:
Bnet=√B2V+B2H
- Substituting the values:
Bnet=√(0.38)2+(0.22)2
- Calculating the squares:
Bnet=√0.1444+0.0484=√0.1928
- Taking the square root:
Bnet≈0.439 Gauss
- Angle of dip (φ) ≈ 59.56°
- Resultant intensity of Earth's magnetic field (B_net) ≈ 0.439 Gauss
Topper's Solved these Questions
- MAGNETISM AND MATTERBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - 5.1
15 Videos
- MAGNETISM AND MATTERBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - 5.2
15 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Video
- MODERN PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICSEXERCISE - Integer Type Questions
17 Videos
Similar Questions
The horizontal and vertical components of earth's magnetic field at a place are 0.3G and 0.52G. The earth's magnetic field and the angle of dip are
View SolutionHorizontal and vertical components of earth’s magnetic field at a place are equal. The angle of dip at that place is ________.
View Solution
Knowledge Check
The horizontal and vertical components of earth's magnetic field at a place are 0.3G and 0.52G. The earth's magnetic field and the angle of dip are
A0.3gandδ=30∘B0.4gandδ=40∘C0.5gandδ=50∘D0.6gandδ=60∘The vertical component of earth's magnetic field at a place is √3 times the horizontal component the value of angle of dip at this place is
A30∘B45∘C60∘D75∘At a certain location in Africa, compass points 12∘ west of geographic north, figure. The north tip of magnetic needle of a dip circle placed in the plane of magnetic meridian points 60∘ above the horizontal. The horizontal component of earth's field is measured to be 0.16gauss. Specify the direction and magnitude of the earth's field at the location.
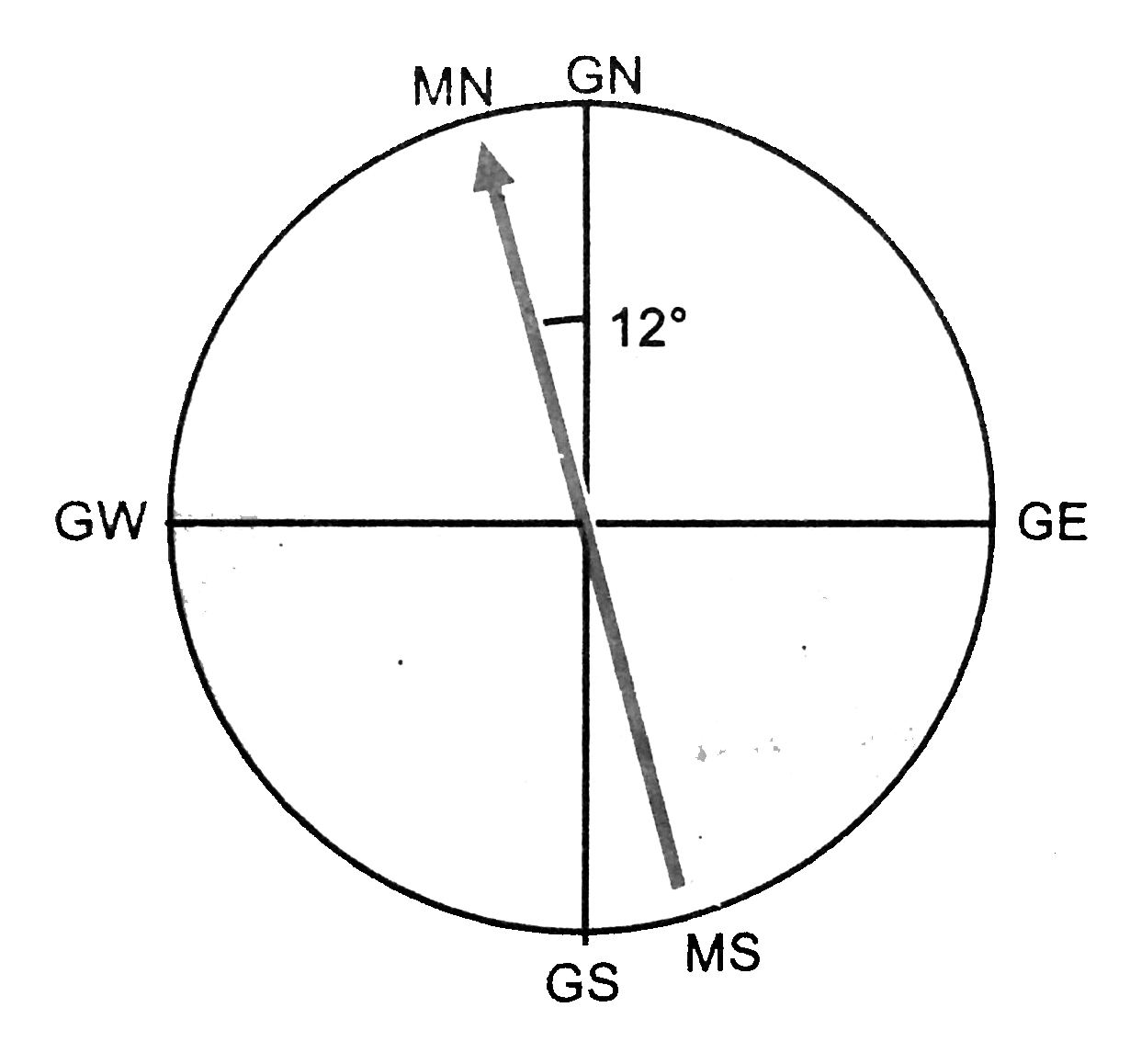 A0.32GB0.42GC4.2GD3.2G
A0.32GB0.42GC4.2GD3.2G
The vertical component of earth's magnetic field at a place is √3 times the horizontal component the value of angle of dip at this place is
View SolutionThe horizontal component of earth.s magnetic field On at a place is 1√3 times the vertical component. Determine the angle of dip at that place.
View SolutionThe value of horizontal component of earth's magnetic field at a place is −.35×10−4T. If the angle of dip is 60∘, the value of vertical component of earth's magnetic field is about:
View SolutionAt a place horizontal and vertical components of earthsmag≠ticfieldareasfollows<br>BH=1G 5^⋅ East of north
BV=1G vertically upward
Then inclination and declination are respectively
View SolutionWhat is the angle of dip at a place where horizontal and vertical components of earth's field are equal?
View Solution